Chapter 99 The Effects and Action of Scleral Buckles in the Treatment of Retinal Detachment
Effects of scleral buckles on the geometry of the eye
Axial length changes after scleral buckles
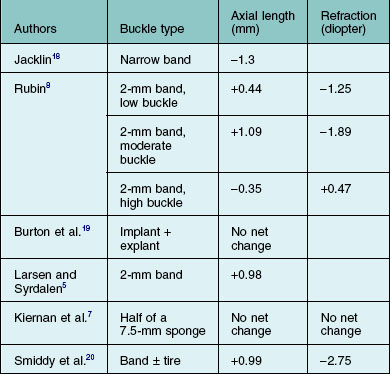
The axial length of the eye may change after placement of a scleral buckle. Radial soft silicone sponges appear to induce little change in the axial length of the eye. Segmental scleral buckles may cause a hyperopic shift while encircling scleral buckles may produce increases or decreases in axial length, depending on the scleral buckle material, the location of the buckle, and the height of the buckle.1,2 Hard silicone encircling buckles most commonly increase the axial length of the eye,3–5 although some eyes show no apparent change in axial length after placement of an encircling buckle.6,7 Occasionally, high encircling silicone buckles may decrease the axial length of the eye.4,8
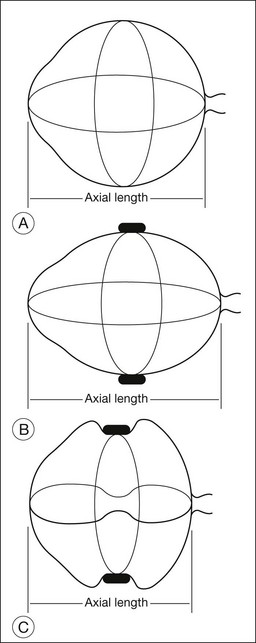
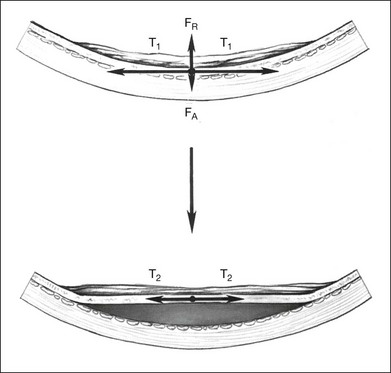
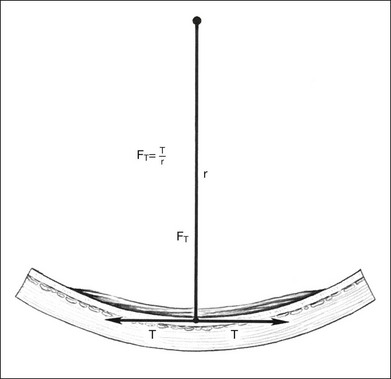
The changes in axial length induced by circumferential scleral buckles can be understood by analyzing the geometry of the eye with an encircling buckle in place. If a circumferential buckle is tightened around the equator of the eye, the first effect is to decrease the circumference of the eye in coronal cross-section, causing the eye to assume an elliptical shape in horizontal cross-section. The normally spherical human eye acquires the shape of a prolate spheroid after placement of a broad circumferential buckle (Fig. 99.1). A prolate spheroid is the result of the rotation of an ellipse about its major (longer) semiaxis. The eye is still circular in coronal cross-section, because the encircling buckle constricts the equator of the eye into a circle of smaller circumference. The eye becomes more elliptical in sagittal and horizontal cross-section. A decrease in the circumference of the eye caused by indentation from a scleral buckle in the coronal plane is accompanied by an increase in the anteroposterior dimension of the eye (Fig. 99.1) in the sagittal and horizontal planes as the eye becomes elongated by the equatorial constriction from the encircling scleral buckle. This change from a sphere to a prolate spheroid occurs primarily because of the relative inelasticity of the sclera at physiologic intraocular pressures in the fluid-filled eye. If the circumferential buckle is tightened high enough, the eye will assume the shape of a dumbbell. The anteroposterior axial length of the eye decreases at very high circumferential buckle heights because part of the circumference of the sclera in the horizontal and sagittal cross-sections is used to create the dumbbell-shaped indentation in the sclera (Fig. 99.1).
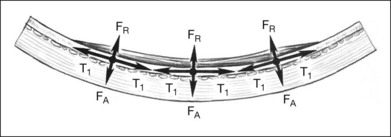
Placement of two mattress sutures per quadrant to invaginate the sclera beneath a circumferential buckle has additional effects on the geometry and axial length of the eye. Indentation of the sclera by tightening mattress sutures around a circumferential hard silicone exoplant causes a decrease in the axial length of the eye if there is no concomitant circumferential shortening of the encircling buckle.4 The mattress sutures pull together the anterior and posterior sclera where the suture bites are anchored, decreasing the axial length of the eye. As the mattress sutures are tightened, the sclera is indented by the circumferential buckle beneath the mattress sutures, causing the eye to acquire a dumbbell shape in horizontal and sagittal cross-sections.
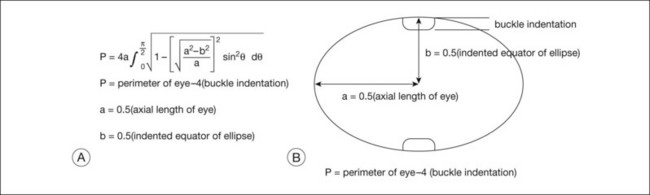
A geometric model to explain the effects of circumferential scleral buckles on the axial length and to predict these axial length changes was developed to improve the predictability of these axial length changes. The geometric model of the effects of scleral buckles on axial length is based on the following assumptions: First, the overall contour of the eye with a scleral buckle is assumed to be an ellipse. Second, the circumference of the eye is constant, because the buckle does not stretch or shrink the sclera substantially. Figure 99.2 gives a gross estimate of the circumference of the indented eye wall (which equals π times axial length) after a scleral buckle, based on the preoperative perimeter of the eye and the amount of indentation of the eye wall by the scleral buckle at the equator. The axial length of the eye can then be calculated following placement of the scleral buckle, using the formula to solve for the axial length to predict the effect of the scleral buckle on refraction.
Refractive errors caused by scleral buckles
Three major types of refractive error can be induced by scleral buckles used for retinal reattachment. The first type is an astigmatic error caused by changes in the corneal curvature; the second type is a change in the spherical equivalent induced by changes in axial length, anterior chamber depth, or position of the crystalline lens. The third is higher-order aberrations which were found to be greater when segmental scleral buckles are used rather than circumferential buckles.9 The higher-order aberrations persist at least 3 months but may improve over time.
Astigmatic errors
Regular and irregular corneal astigmatism are most likely to result from placement of segmental or radial exoplants.8,10–13 Some astigmatic errors may be persistent, requiring correction,1 although many astigmatic errors improve within several months following surgery.14 Corneal astigmatism usually results when a high, anterior radial buckle is placed. The indentation of a radial buckle in the anterior sclera can be transmitted to the cornea because of the inelasticity of both the sclera and the cornea. If the eye were highly elastic like a balloon, indentation of the eye from a radial buckle would be present only directly under the buckle. The radial buckle would have no effect on the surrounding sclera. Because the sclera is less elastic than the rubber skin of a balloon, the radial buckle causes some indentation in the surrounding sclera beyond the extent of the buckle itself. The greatest astigmatic errors occur in eyes in which a segmental buckle spans one to two quadrants.15 Encircling circumferential buckles of uniform width rarely produce substantial astigmatism.
Spherical equivalent errors
Changes in refraction from scleral buckles caused by changes in axial length and lens position are more common than are astigmatic errors. Most eyes with encircling buckles have a small shift toward myopia; this is associated with an increase in axial length.5,8,16 Shallowing of the anterior chamber associated with displacement of the lens anteriorly in phakic eyes with an encircling buckle may also contribute to a shift toward myopia.8 Anterior displacement of the lens becomes less pronounced several months after retinal reattachment in most eyes.16 The buckle height decreases substantially with radial buckles over a period of months, while buckle height does not decrease as much in eyes with circumferential buckles.17 Some eyes with high circumferential scleral exoplants have a shift in refraction toward hyperopia.7 The changes in axial length and refractive error with different scleral buckles are reported in the literature and are summarized in Table 99.1. When scleral buckles are placed in children, the scleral buckle may retard growth-related increases in the axial length of the eye, causing the eye to develop less myopia than the fellow eye.21 This has been considered as a possible method to slow progressive myopia in children if it could be made more predictable.
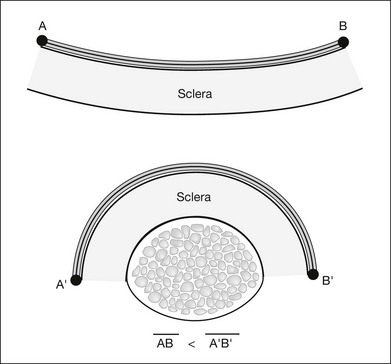
Scleral chord versus scleral arc length
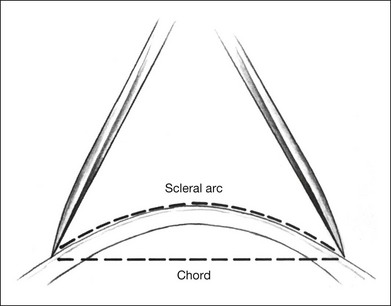
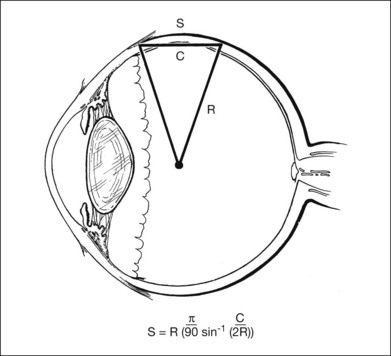
The curvature of the spherical globe must be considered when distances on the surface of the globe are measured. Calipers are commonly used to measure distances for placement of scleral sutures to hold the scleral buckle in place. Calipers measure the shortest line between two points on the spherical globe, which is called the scleral chord length (Fig. 99.3). The distance measured along the curved surface of the globe between two points is the scleral arc length. The scleral chord length measured by the calipers is always shorter than the scleral arc length.22 The scleral arc length can be calculated from the scleral chord length, and vice versa, using the formula in Figure 99.4. Scleral arc length and scleral chord length are similar when the chord length is a small percentage of the radius of the globe. A caliper setting (chord length) of 8 mm corresponds to a scleral arc length of 8.16 mm, a 2% error; a caliper setting of 13 mm corresponds to a scleral arc length of 13.74 mm, a 5.7% error. The discrepancy between scleral chord length and scleral arc length increases nonlinearly with larger caliper measurements and should be considered whenever calipers are used to measure large distances on the globe.
Effects on the internal geometry of the eye
The major variables that determine the internal geometry of indentation induced by the scleral buckle exoplant include: (1) shape of the buckle; (2) composition of the buckle (silicone sponge versus hard silicone); (3) suture placement with respect to the dimensions of the buckle; (4) suture tension; (5) distribution of tension from the suture to the buckle; and (6) intraocular pressure. An analysis of scleral indentation from a 5-mm radial silicone sponge showed that the following factors decreased indentation: (1) placement of the suture bites too close or too far apart; (2) high intraocular pressure; (3) short suture bites in the sclera; (4) loose sutures; and (5) use of a half-thickness sponge compared with a full-thickness sponge. Factors that increased scleral indentation included: (1) low intraocular pressure and (2) tight sutures.23,24
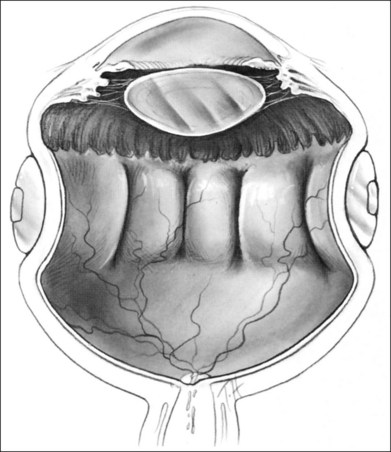
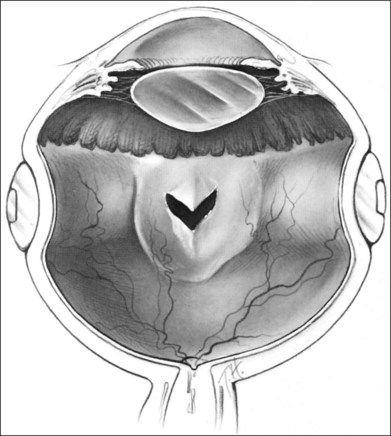
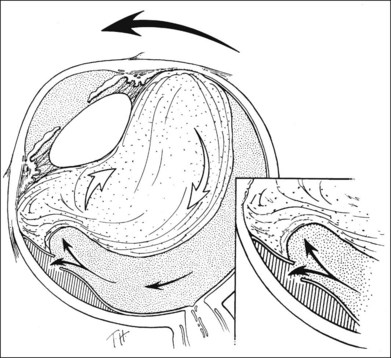
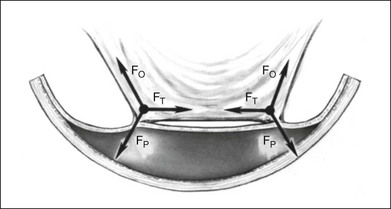
The orientation of the scleral buckle also helps to determine the topography of the indentation in the sclera. Radial buckles appear to offer advantages in the support of a solitary horseshoe-shaped retinal tear.25–27 Moderate to high circumferential encircling scleral buckles cause radial folding of the retina. This radial folding occurs because the encircling buckle forces a reduction in the normal circumference of the eye in the equatorial meridian. The sclera and retina are unable to shrink to the new, smaller circumference, so the “excess” retina, choroid, and sclera are thrown into radial folds to conform to the smaller circumference of the eye induced by the encircling buckle (Fig. 99.5). The circumferential shortening of the eye beneath an encircling buckle is the basis of the fishmouth phenomenon (Fig. 99.6).28,29 Wedge-shaped buckles and radial scleral buckles minimize the risk of the fishmouth phenomenon because they cause less circumferential shortening over the retinal tear than do encircling scleral buckles.
Volume changes in the eye after scleral buckles
Indentation of the eye wall by a scleral buckle displaces fluid from the vitreous cavity, causing a reduction in the volume of the vitreous cavity. This occurs because a sphere contains the largest volume of fluid with the least surface area. The amount of fluid displaced depends on the buckle type and configuration. The amount of volume displacement is small for most buckles but can be substantial for broader encircling buckles. Estimation of the intraocular volume of an eye with a scleral buckle is important in several circumstances: (1) estimation of how much fluid must be withdrawn from the vitreous cavity or drained from the subretinal space to permit placement of a specific scleral buckle; (2) injection of pharmacologic agents such as antibiotics or antimetabolites into the vitreous, when therapeutic and toxic concentrations must be considered and (3) injection of expansile gases into the vitreous. The volume displacement of a scleral buckle can be predicted as a function of the following variables: (1) the axial length of the eye; (2) the buckle width measured anterior and/or posterior to the equator; (3) the buckle circumference; and (4) the buckle height. The formula for determining volume displacement by a scleral buckle is given in Figure 99.7. A 5-mm radial silicone sponge displaces only about 0.2 mL, or 5%, of the vitreous cavity volume.30 This is why placement of a radial sponge in a nondrainage procedure only occasionally elevates the intraocular pressure substantially. A 2.5-mm wide silicone encircling band (#240 style) displaces about 0.5 mL, or 12%, of the vitreous cavity volume.30 A 7-mm wide hard silicone encircling buckle (#287 style) displaces from 1.3 mL (33%) to 1.7 mL (43%) of the vitreous cavity volume of a phakic eye, depending on the buckle geometry and height.30 The decrease in vitreous cavity volume increases with increasing buckle width and height for circumferential buckles, as shown in Table 99.2. Magnetic resonance imaging has been used to confirm the decreased volume of the eye induced by scleral buckles. An encircling band in this study reduced the vitreous cavity volume by an average of 1.7 mL.31
Table 99.2 Estimated vitreous cavity volume displacement of scleral buckles
| Scleral buckle | Vitreous cavity volume displacement (mL) |
|---|---|
| Half of 5-mm sponge | 0.09–0.15 |
| 3 × 5 mm sponge | 0.11–0.20 |
| 5-mm round sponge | 0.14–0.22 |
| #240 style (circumferential) | 0.47–0.48 |
| #276 style (circumferential) | 1.08–1.13 |
| #287 style (circumferential) | 1.32–1.57 |
| #280 style (circumferential) | 1.82–1.88 |
(Data from Thompson JT, Michels RG. Volume displacement of scleral buckle. Arch Ophthalmol 1985;103:1822–4.30)
Scleral buckles and ocular rigidity
Placement of a scleral buckle changes the normal ocular rigidity. Ocular rigidity is the change in intraocular pressure for a given change in intraocular volume and is a measure of the elasticity of the eye. Intraocular pressure normally increases rapidly as microliter volumes are injected into the eye. The increase in intraocular pressure (ocular rigidity) is decreased in eyes with an encircling scleral buckle because the volume of the vitreous cavity was decreased with placement of a scleral buckle.32,33 This decrease in volume is related to changes in the shape of the eye caused by the scleral buckle.30 As the intraocular pressure is increased by injection of saline solution or gas into the eye, the eye becomes less elliptical and more spherical as the sutures holding the buckle are stressed. The net effect is to decrease the buckling effect and to increase the intraocular volume slightly so that the intraocular pressure does not rise as rapidly as in the normal eye. This effect can be better understood by considering an eye with an encircling buckle and no invagination by scleral sutures. If water is injected into the eye, the encircling buckle will stretch as the eye assumes a more spherical shape. Once the buckle has stretched so that the eye returns to its original spherical shape (before placement of the buckle), the intraocular pressure will increase rapidly. The changes in ocular rigidity have several important clinical ramifications. First, methods of measuring intraocular pressure that depend on a standard ocular rigidity, such as the Schiøtz tonometer and Tonopen® are less accurate in eyes with scleral buckles. Second, an injection of fluid or gas into an eye with a scleral buckle will cause less elevation of intraocular pressure than injection of the same volume into a normal eye, if all other factors are equal. Placement of an intraocular gas bubble into the vitreous also reduces ocular rigidity because the gas in the vitreous cavity is more compressible than the vitreous fluid it replaces. Eyes with reduced ocular rigidity from an intraocular gas bubble and an encircling scleral buckle require even larger volumes of fluid aspiration to reduce the intraocular pressure than normal eyes with elevated intraocular pressure.34
Scleral buckles and ocular blood flow
Encircling scleral buckles also change ocular blood flow.35 This does not create clinically recognizable problems in most patients undergoing scleral buckle for retinal reattachment, but can create ocular ischemia or peripheral visual field defects.36 As a result, some surgeons advocate cutting the circumferential band routinely after the retina has reattached to improve ocular blood flow.37
Effects of scleral buckles on the RPE and retina
An overview of forces acting on the retina
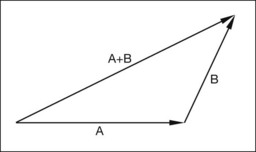
Vitreoretinal traction forces play an important role in the pathogenesis of retinal tears, rhegmatogenous retinal detachments, and traction retinal detachments. Forces that act on the retina have both magnitude and direction and are best represented by vectors. A force vector is represented graphically by an arrow with a length and a direction. The length of the vector is proportional to the magnitude of the force, and the direction indicates the direction along which the force acts (Fig. 99.8). Simple arithmetical operations such as addition and subtraction can be performed on vectors to determine the net force produced by two forces acting on the same point. The simplest way to add vectors is to draw the vectors so that the arrowhead of the first vector connects to the tail of the second vector. A new vector, which is the sum of the two vectors, is drawn from the tail of the first vector to the head of the second vector (Fig. 99.8).
A second important concept is the equilibrium of vector forces. If two vector forces of equal magnitude simultaneously act in opposite directions on the same point, there is no displacement of the object at that point. A simple example of equilibrium involving opposing vector forces is found in localized vitreous traction on the retina at the edge of a posterior vitreous detachment (PVD). If the force of vitreous traction on the retina at the edge of a PVD is counterbalanced by retinal adherence to the eye wall, no tear will develop. If vitreous traction on the retina exceeds photoreceptor adherence to the RPE and retinal tensile strength, a retinal tear will develop to re-establish equilibrium (Fig. 99.9). Vector algebra can be used to understand how various forces acting on the retina lead to retinal tears, traction retinal detachments, and rhegmatogenous retinal detachments. Scleral buckles help to alter the magnitude and direction of these vectors, thus promoting closure of retinal breaks and retinal reattachment.
Forces that lead to retinal tears and detachments
Vitreous traction
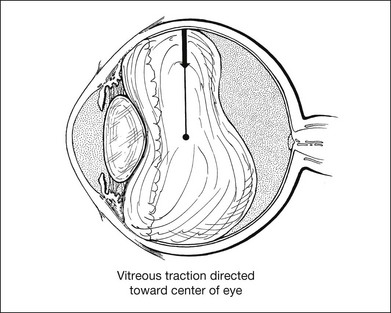
Vitreous traction may be exerted in many different directions (i.e., perpendicular, tangential, or oblique) with respect to the curved surface of the retina. An example of relatively pure perpendicular (or radial) vitreous traction occurs with a superior horseshoe-shaped tear resulting from posterior vitreous detachment (Fig. 99.10). As the posterior hyaloid detaches, gravity causes the vitreous gel to pull away from the superior retina. A focal adhesion of the vitreous to the retina may cause a tear to develop if the force exerted by the vitreous on the retina exceeds the adherence of the photoreceptors to the RPE and the tensile strength of the retina. The force exerted by the vitreous is primarily perpendicular or oblique to the surface of the retina. The importance of gravitational forces on the vitreous in the genesis of retinal tears is emphasized by the observation that most horseshoe-shaped tears occur in the superior retina. An example of relatively pure tangential traction on the surface of the retina occurs with a localized epiretinal membrane causing distortion of the macula (Fig. 99.11). Most of the traction from an epiretinal membrane occurs tangentially on the surface of the retina, although there is a small inward radial component to this traction because of the concave shape of the eye wall.38 The origin of the inward radial traction on curved surfaces is analyzed later in this chapter.
It appears that radial traction on the retina is more likely to produce retinal breaks than is tangential traction. Most vitreoretinal traction causing a retinal tear, traction retinal detachment, or rhegmatogenous retinal detachment is oblique to the surface of the retina. Oblique vitreoretinal traction can be separated into orthogonal vectors (oriented at 90°) composed of varying mixtures of radial and tangential traction (Fig. 99.12). Some of the forces that can cause traction on the retina include: (1) gravitational forces on the vitreous gel attached to retina; (2) inertial forces transmitted from the vitreous to the retina during ocular movements or blunt trauma; (3) contraction of the vitreous gel at sites of vitreoretinal attachment caused by cellular proliferation; and (4) contractile fibrocellular membranes on the surface of the retina posterior to a PVD. Myopic eyes with long axial lengths have higher shearing forces on the retina with saccadic eye movements than emmetropic eyes, which may predispose them to develop retinal tears.39
Fluid movement and retinal breaks
Fluid currents in eyes with retinal tears may allow fluid to move from the vitreous cavity into the subretinal space, creating a rhegmatogenous retinal detachment. Rotational eye movements appear to be especially important in forcing fluid through a retinal tear, leading to retinal detachment.40,41 Fluid movement associated with rotational eye movements appears more likely to cause a retinal detachment when vitreous traction elevates the flap of a retinal tear than when a retinal hole is present without vitreous traction. The elevated retinal flap with vitreous traction may trap vitreous fluid and funnel it into the tear. The vitreous fluid can then act as a wedge, interposing itself between the retina and the RPE as the eye rotates (Fig. 99.13).42 Once the normal adhesion of the retina to the RPE is disrupted, rotational eye movements can force additional fluid into the subretinal space, thereby extending the retinal detachment. A retinal hole in the absence of vitreous traction does not catch the vitreous fluid from rotational eye movements as easily and thus is less likely to lead to retinal detachment. If retinal detachment occurs, continued fluid flux through the retinal tear is important in maintaining the retinal detachment.43–45
Epiretinal membranes, cellular proliferation, and retinal breaks
Epiretinal membranes with cellular proliferation on the surface of the retina may also cause retinal breaks and retinal detachment. As the epiretinal membranes contract, they seek the shortest distance between areas of attachment to the concave inner surface of the retina. The shortest distance between two points on the curved retinal surface is a straight line, or chord; the shortest distance between three points on the curved retinal surface is a series of lines within a single plane. The epiretinal membrane that initially conforms to the concave inner surface of the retina can cause a traction detachment of the retina in the configuration of a plane. The planar configuration occurs because this will minimize the tangential tension within the epiretinal membrane. This is analogous to the clinical observation of a traction retinal detachment caused by epiretinal proliferation on the surface of the retina behind a PVD (Fig. 99.14). In most instances the vitreous gel also contributes to the traction retinal detachment. Attachment of the vitreous gel to fibrocellular epiretinal membranes may produce additional radial or oblique traction on the retina, leading to more complicated configurations of retinal detachments such as the table-top traction retinal detachment sometimes seen in eyes with severe proliferative diabetic retinopathy (Fig. 99.15). These radial, tangential, and oblique forces on the retina may also cause retinal breaks, creating a traction–rhegmatogenous retinal detachment. The biomechanics of these detachments are much more complicated because their configuration is determined by an equilibrium of vitreoretinal traction, retinal elasticity, and fluid flux through retinal breaks and absorption of subretinal fluid. The detached retina will assume the configuration that balances all of these synergistic and opposing forces.
Forces that promote attachment of the retina
A number of physiologic forces maintain or attempt to restore attachment of the neurosensory retina to the RPE (see Chapter 29, Cellular effects of detachment and reattachment on the neural retina and retinal pigment epithelium). Adhesions between the neurosensory retina and RPE/choroid also assist in maintaining reattachment. Scleral buckles alter the effects of vitreous traction, epiretinal membranes, and fluid movement.
Physiologic adhesion between retina and RPE
Several factors promote adhesion between the retina and the RPE. First, adhesion of the RPE and photoreceptors is assisted by a viscous mucopolysaccharide substance between the villous processes of the RPE interdigitating with the photoreceptors.46 This appears to account for only a small component of the adhesion between the retina and the RPE. Second, an oncotic pressure difference between the choroid and subretinal space makes another small contribution to promoting adhesion of the retina to the RPE.47,48 The oncotic pressure difference arises because proteins in the choroid cannot easily pass through the RPE and Bruch’s membrane into the subretinal space.49 Third, hydraulic forces on the retina promote adhesion of the retina to the RPE. The hydraulic forces are a result of intravitreal fluid pushing against the retina at a physiologic intraocular pressure. The hydraulic forces are produced by more rapid passage of fluid from the subretinal space through the sclera than from the vitreous through the retina.50 The hydraulic forces have been compared to an inner tube (the retina) forced against the inner wall of a tire (the sclera). Hydraulic forces are independent of the RPE pump and explain why the retina can remain attached after removal of the choroid and RPE in an eye wall resection, such as is sometimes used for removal of a choroidal melanoma.51 Hydraulic forces would be expected to produce a lower pressure in the subretinal space than in the intravitreal space. However, attempts to measure this small pressure difference directly have not been successful.52 Finally, the most important factor maintaining retinal attachment is the RPE pump, which actively removes fluid from the subretinal space, promoting adhesion of the RPE to the photoreceptors.26 These four types of force maintain apposition of the retina and the RPE under normal physiologic conditions. Vitreous traction and retinal breaks may overcome these adhesive forces, leading to retinal detachment.
Several properties of the RPE–photoreceptor adhesion are important in determining the types of retinal tears and retinal detachments that can occur with vitreoretinal traction. The adhesive force between the RPE and photoreceptors paradoxically appears to be weaker with small tractional forces than larger tractional forces in vitro.53 The variability of the strength of adhesion between the retina and the RPE as a function of the tractional force on the retina is a result of the viscoelastic properties of the mucopolysaccharide holding the retina and the RPE together. These viscoelastic properties can be understood by considering the force required to pull adhesive tape off a surface. The adhesion of the adhesive tape to a surface is lower at lower rates of traction than at higher rates of traction. Hence, the adhesion of the retina to the RPE would be expected to be lower at lower rates of traction than a higher rate of traction.53
The retina itself also behaves like a viscoelastic substance when tractional forces cause elongation of the retina. The retina has greater elongation at lower peeling rates than at higher peeling rates.53,54 Smaller tractional forces on the retina exerted over a longer period of time tend to produce larger traction retinal detachments than do larger tractional forces over a short period.55 This can explain why relatively minimal chronic vitreous traction can produce an extensive traction retinal detachment, whereas larger forces from short-lived vitreous traction associated with ocular trauma may produce no retinal detachment or may produce a retinal tear rather than a traction retinal detachment. These properties of the retina are a result of the mechanics of viscoelastic substances, which depend on the rate of peeling. The forces required to produce retinal tears and detachments are greater in vivo than in vitro because of active metabolic processes, such as the RPE pump, that favor retinal adhesion.56 The presence of vitreous traction, retinal breaks, and fluid currents in the eye may overcome these adhesive forces, leading to retinal detachment. The scleral buckle and chorioretinal adhesion counteract the forces that detach the retina and help re-establish an adhesion between the retina and the RPE.
Thermal chorioretinal adhesions
The final chorioretinal adhesions formed by diathermy, cryopexy, and laser have similar strengths and are certainly adequate to maintain apposition of the retina and the RPE if there is no substantial traction on the retina. The rapidity of onset of the chorioretinal adhesion is not the same for these three methods, however. Laser photocoagulation appears to induce a more rapid chorioretinal adhesion than cryopexy or diathermy.57,58 The chorioretinal adhesion induced by laser photocoagulation starts within 24 hours of treatment and increases rapidly within 3 days, whereas the adhesion formed by cryopexy or diathermy takes at least several days to start to form and does not reach maximum strength until about 2 weeks.57,58 Cryopexy given at the time of scleral buckle for retinal reattachment and laser photocoagulation 1 month later have similar efficacy in maintaining retinal reattachment in one randomized trial.59
Scleral buckles and vitreous traction
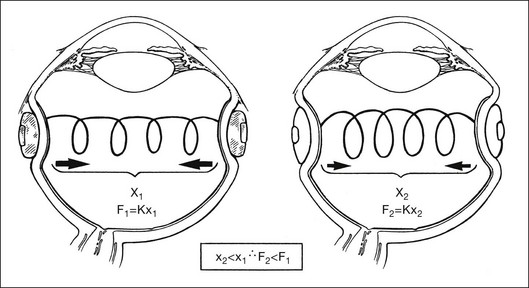
Scleral buckles help in several ways to counteract the forces that tend to detach the retina. Indentation of the eye wall produced by the scleral buckle can decrease vitreous traction on the retinal tear in rhegmatogenous retinal detachment. Scleral buckles may also decrease vitreous traction in traction retinal detachments, causing the detachment to decrease in size or to resolve completely. Vitreous traction causing elevation of the retinal break in a traction–rhegmatogenous retinal detachment may perpetuate the retinal detachment by allowing fluid from the vitreous cavity to pass through the retinal break into the subretinal space. The scleral buckle relieves this vitreoretinal traction by decreasing the magnitude and possibly changing the direction of the vitreous traction on the retinal tear. Circumferential scleral buckles help to decrease transretinal traction by decreasing the diameter and circumference of the vitreous base. This effect can be understood by comparing the vitreous to a spring and applying Hook’s law. The force exerted by a stretched spring is greater than a spring with minimal stretch (Fig. 99.16). The force of stretch is directly proportional to the distance the spring is stretched. Reducing the diameter of the vitreous cavity in the vitreous base with a circumferential buckle decreases the transvitreal traction. Changes in the magnitude and direction of vitreous traction on the retina shift the equilibrium back toward retinal reattachment. Optical coherence tomography has been used to image the retina overlying scleral buckles and has confirmed that residual vitreous traction and unsupported retinal breaks are important causes of persisting retinal detachment.60 The capability of the scleral buckle to relieve traction and promote retinal adhesion has been confirmed when scleral buckles are used to treat retinal detachments without the use of any chorioretinal adhesions. Two randomized trials found no difference in retinal reattachment rates with the use of scleral buckles whether or not any retinopexy was used.61,62
Scleral buckles and traction on the retinal surface
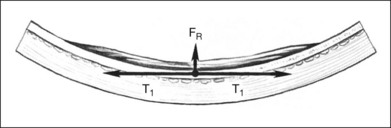
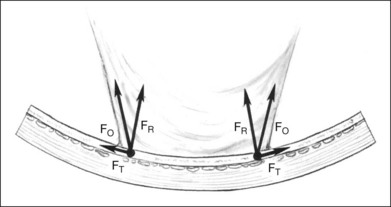
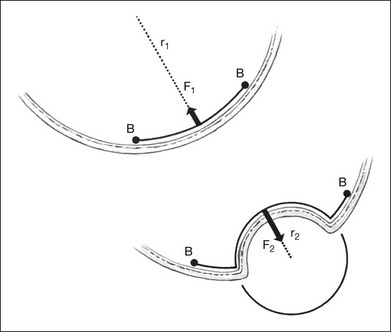
Cellular epiretinal proliferation adherent to the surface of the retina may also promote traction retinal detachment by exerting traction on the retina or may precipitate a rhegmatogenous detachment if the force is strong enough to tear the retina. An epiretinal membrane on the surface of the retina produces retinal traction along the concave eye wall. This traction is composed of two vectors. The first is tangential to the retina and is caused by tension in the contractile epiretinal membrane. The second is directed radially inward, toward the center of the eye, and is a result of tangential traction on a curved surface (Fig. 99.17). At each point along the epiretinal membrane is a small force tangential to the retina and a small force directed inward toward the center of the eye. The force directed radially inward tends to pull the epiretinal membrane and retina away from the RPE. This radial force is normally counterbalanced by adhesion of the retina to the RPE. If the tension on the epiretinal membrane becomes great enough, the radial inward force may exceed the RPE adhesive capacity, causing a retinal tear or detachment (Fig. 99.14).
The radial force from tangential traction on the retina can be approximated by the formula in Figure 99.18.38 The radial inward force on the retina per unit length is proportional to the tension of the epiretinal membrane. The greater the tension within the epiretinal membrane, the greater the radial inward force tending to detach the retina. The radial inward force from the epiretinal membrane is inversely proportional to the radius of curvature of the eye wall. Hence, an epiretinal membrane on an eye with a small radius of curvature exerts more radial inward force on the retina than does the same epiretinal membrane on an eye with a large radius of curvature, if all other variables are equal.
The formula in Figure 99.18 also explains how scleral buckles help to promote retinal reattachment in eyes with breaks adjacent to epiretinal membranes and in eyes with traction retinal detachments secondary to epiretinal proliferation. The scleral buckle alters the shape of the eye wall from the normal concave contour to a convex contour over the scleral buckle. Without the scleral buckle, the radial force induced by the epiretinal membrane on the retina is directed inward toward the center of the eye. With the scleral buckle, the radial force is directed toward the convex center of curvature of the radial buckle (Fig. 99.19). The scleral buckle reverses the direction of the radial force induced by the epiretinal membrane from an inward force, tending to detach the retina, to an outward force, promoting retinal reattachment. Furthermore, because the convex radius of curvature of the retina on the scleral buckle is much smaller than the concave radius of curvature of the eye, the magnitude of the radial outward force promoting retinal reattachment with a scleral buckle is larger than the original radial inward force tending to detach the retina.
This analysis of vector forces induced by an epiretinal membrane can be understood by considering a tight surgical glove on a hand. When the hand is cupped, the glove will lift off the palm of the hand because the glove is like a tight trampoline bridging the edges of the concave surface of the palm of the hand. The glove on a cupped hand is analogous to an epiretinal membrane lifting the retina off the RPE. Traction retinal detachments would always occur with epiretinal proliferation on the surface of the retina if no adhesive forces were present to counterbalance the radial inward force on the retina from the epiretinal membrane. When the palm of the hand is flattened and hyperextended, the elastic surgical glove is pulled tightly against the palm of the hand. The force vector that pulled the surgical glove off the cupped hand reverses when the hand is hyperextended, pulling the glove against the convex surface of the palm of the hand. This is analogous to the effect of a scleral buckle changing the concave eye wall to a convex configuration, promoting retinal reattachment. Radial scleral buckles may also assist in closure of retinal breaks by stretching the retina slightly on the scleral buckle, since the retina must conform to the increased arc length of the scleral buckle (Fig. 99.20).25
Scleral buckles and fluid movement
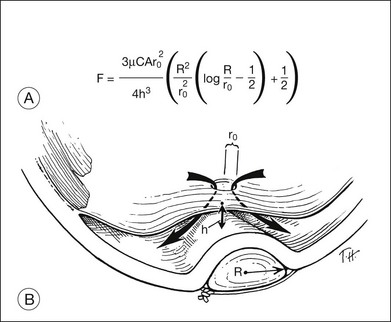
Fluid currents associated with rotational movements of the eye are important in creating and maintaining retinal detachments, as discussed previously.41 Scleral buckles may alter the direction and magnitude of fluid currents in eyes with retinal detachment, decreasing the movement of vitreous fluid through tears into the subretinal space. Scleral buckles may change the fluid flux through retinal tears. The distance between the RPE and a retinal tear is reduced when the eye wall is indented by a scleral buckle. This displaces the pre-existing subretinal fluid away from the tear and helps to bring the RPE and the retinal tear closer together. The distance from the retinal tear to the RPE is important in determining whether the retina will reattach or remain detached. The closer the retinal tear is to the RPE, the greater the force promoting retinal reattachment. A quantitative model of retinal reattachment force was developed to try to predict the magnitude of retinal reattachment forces in eyes with rhegmatogenous retinal detachment treated with a radial buckle (Fig. 99.21).43 The retinal reattachment force in this model results from the absorption of subretinal fluid by the RPE pump, which brings the retina and the RPE into closer apposition. This model predicts that the retinal reattachment force is inversely proportional to the cube of the distance from the retinal hole to the RPE on the buckle. Halving the distance from the retinal tear to the buckle causes an eightfold increase in the retinal reattachment force. This analysis would predict that most retinal tears within 3 mm of the RPE will spontaneously reattach without drainage of subretinal fluid, and this appears to be confirmed by clinical observations.43 A subsequent finite element analysis using this model made the somewhat surprising prediction that rapid eye movements may actually help to reattach the retina better than relative ocular immobility which has been advocated for years to help absorb persisting subretinal fluid.63
Scleral buckles can also displace vitreous fluid away from the tear, plugging the tear with solid vitreous gel. The movement of fluid from the vitreous cavity through the tear is impeded when the tear is occluded by solid vitreous gel.64 Larger scleral buckles may displace much of the liquid vitreous, leaving solid vitreous gel in the vitreous cavity to plug retinal breaks. If additional tamponade of retinal breaks is needed, intraocular gas bubbles can eliminate or decrease the movement of vitreous fluid through the retinal break because of the high surface tension at the gas–water interface at the site of the retinal tear.
1 Hayashi H, Hayashi K, Nakao F, et al. Corneal shape changes after scleral buckling surgery. Ophthalmology. 1997;104:831–837.
2 Okada Y, Nakamura S, Kubo E, et al. Analysis of changes in corneal shape and refraction following scleral buckling surgery. Jpn J Ophthalmol. 2000;44:132–138.
3 Flament J. Etude optico-echographique des modifications biometriques de l’axe antero-posterieur de l’oeil provoquées par quelques techniques de la chirurgie chorioretinienne. Arch Ophthalmol (Paris). 1973;33:397–412.
4 Harris MJ, Blumenkranz MS, Wittpenn J, et al. Geometric alterations produced by encircling scleral buckles: biometric and clinical considerations. Retina. 1987;7:14–19.
5 Larsen JS, Syrdalen P. Ultrasonographic study on changes in axial eye dimensions after encircling procedure in retinal detachment surgery. Acta Ophthalmol. 1979;57:337–343.
6 Burton TC. Irregular astigmatism following episcleral buckling procedure with the use of silicone rubber sponges. Arch Ophthalmol. 1973;90:447–448.
7 Kiernan JP, Leveille AS, Morse PH. Axial length following scleral buckling. Retina. 1982;2:176–178.
8 Rubin ML. The induction of refractive errors by retinal detachment surgery. Trans Am Ophthalmol Soc. 1975;73:452–490.
9 Okamoto F, Yamane N, Okamoto C, et al. Changes in higher-order aberrations after scleral buckling surgery for rhegmatogenous retinal detachment. Ophthalmology. 2008;115:1216–1221.
10 Goel R, Crewdson J, Chignell AH. Astigmatism following retinal detachment surgery. Br J Ophthalmol. 1983;67:327–329.
11 Mensher JH, Burton TC. Corneal curvature changes after scleral buckling. Current concepts in ophthalmology. Blodi FC, ed. Current concepts in ophthalmology. St Louis: Mosby; 1974;Vol. 4.
12 Wang HZ, Chen MT, Chang CH, et al. The changes in ocular axial length and corneal curvatures after scleral buckling for retinal detachment. Kaohsiung J Med Sci. 1994;10:77–83.
13 Wolter JR. Regular astigmatism resulting from retinal detachment surgery in a young man with a disinsertion. J Pediatr Ophthalmol. 1967;4:27–29.
14 Weinberger D, Lichter H, Loya N, et al. Corneal topographic changes after retinal and vitreous surgery. Ophthalmology. 1999;106:1521–1524.
15 Kinoshita M, Tanihara H, Negi A, et al. Vector analysis of corneal astigmatism after scleral buckling surgery. Ophthalmologica. 1994;208:250–253.
16 Fiore JV, Jr., Newton JC. Anterior segment changes following the scleral buckling procedure. Arch Ophthalmol. 1970;84:284–287.
17 Theodossiadis GP, Maniakis SG, Ladas ID, et al. The persistence of buckle height in relation to orientation of buckle fixation: an ultrasonographic study. Acta Ophthalmol. 1994;72:315–318.
18 Jacklin HN. Postoperative refractive changes following various scleral buckling techniques. In: Pruett RC, Regan CJ. Retina congress. New York: Appleton-Century-Crofts, 1972.
19 Burton TC, Herron BE, Ossoinig KC. Axial length changes after retinal detachment surgery. Am J Ophthalmol. 1977;83:59–62.
20 Smiddy WE, Loupe DN, Michels RG, et al. Refractive error changes after scleral buckling surgery. Arch Ophthalmol. 1989;107:1469–1471.
21 Sato T, Kawasaki T, Okuyama M, et al. Refractive changes following scleral buckling surgery in juvenile retinal detachment. Retina. 2003;23:629–635.
22 Thompson JT, Michels RG. Comparison of chord length and scleral arc length when placing sutures for episcleral exoplants. Retina. 1985;5:225–226.
23 Boldrey EE. Variation of technique of episcleral sponge placement: effect on scleral indentation for retinal detachment repair. Ann Ophthalmol. 1981;13:743–746.
24 Draeger J, Guthoff R, Moeller J. Correlations between intraocular pressure and resulting scleral indentation after detachment surgery. Ophthalmol Res. 1982;14:466–472.
25 Goldbaum MH, Smithline M, Poole TA, et al. Geometric analysis of radial buckling. Am J Ophthalmol. 1975;79:958–965.
26 Lincoff H. The rationale for radial buckling. Mod Probl Ophthalmol. 1974;12:484–491.
27 Lincoff H, Kreissig I. Advantages of radial buckling. Am J Ophthalmol. 1975;79:955–957.
28 Pruett RC. The fishmouth phenomenon. I. Clinical characteristics and surgical options. Arch Ophthalmol. 1977;95:1777–1781.
29 Pruett RC. The fishmouth phenomenon. II. Wedge scleral buckling. Arch Ophthalmol. 1977;95:1782–1787.
30 Thompson JT, Michels RG. Volume displacement of scleral buckle. Arch Ophthalmol. 1985;103:1822–1824.
31 Shi MG, Qiao BD, Zhou YX. The volume and dimensions of eyeball analyzed by MRI following encircling scleral buckles. Zhonghua Yan Ke Za Zhi. 2006;24:150–154.
32 Friberg TR, Fourman SB. Scleral buckling and ocular rigidity. Arch Ophthalmol. 1990;108:1622–1627.
33 Johnson MW, Han DP, Hoffman KE. The effect of scleral buckling on ocular rigidity. Ophthalmology. 1990;97:190–195.
34 Simone JN, Whitacre MM. The effect of intraocular gas and fluid volumes on intraocular pressure. Ophthalmology. 1990;97:238–243.
35 Movaffaghy A, Pharmakakis NM, Chamot SR. Effect of squatting on sub-foveal blood flow defect in pseudophakic eyes operated by cerclage. Klin Monbl Augenheikd. 2001;21:323–326.
36 Kimura I, Shinoda K, Eshita T, et al. Relaxation of encircling buckle improved choroidal blood flow in a patient with visual field defect following encircling procedure. Jpn J Ophthalmol. 2006;50:554–556.
37 Lincoff H, Stopa M, Kreissig I, et al. Cutting the encircling band. Retina. 2006;26:650–654.
38 Michels RG, Thompson JT, Rice TA, et al. Effect of scleral buckling on vector forces caused by epiretinal membranes. Am J Ophthalmol. 1987;104:667–669.
39 David T, Smye S, James T, et al. Time-dependent stress and displacement of the eye wall tissue of the human eye. Med Eng Phys. 1997;19:131–139.
40 Lindner K. Uber die Herstellung von Modellen zu Modellversuchen der Netzhautabhebung. Klin Monatsbl Augenheilkd. 1933;90:289–300.
41 Rosengren B, Osterlin S. Hydrodynamic events in the vitreous space accompanying eye movements. Ophthalmologica. 1976;173:513–524.
42 Wilkinson CP, Rice TA. Michels’ retinal detachment, 2nd edn. St Louis: Mosby; 1997.
43 Hammer ME. Retinal reattachment forces created by absorption of subretinal fluid. Doc Ophthalmol Proc Ser. 1981;25:61–75.
44 Pederson JE, Cantrill HL. Experimental retinal detachment. V. Fluid movement through the retinal hole. Arch Ophthalmol. 1984;102:136–139.
45 Tsuboi S, Taki-Noie J, Emi K, et al. Fluid dynamics in eyes with rhegmatogenous retinal detachments. Am J Ophthalmol. 1985;99:673–676.
46 Berman ER, Bach G. The acid mucopolysaccharides of cattle retina. Biochem J. 1968;108:75–88.
47 Marmor MF, Abdul-Rahim AS, Cohen DS. The effect of metabolic inhibitors on retinal adhesion and subretinal fluid resorption. Invest Ophthalmol Vis Sci. 1980;19:893–903.
48 Negi A, Marmor MF. The resorption of subretinal fluid after diffuse damage to the retinal pigment epithelium. Invest Ophthalmol Vis Sci. 1983;24:1475–1479.
49 Toris CB, Pederson JE. Experimental retinal detachment. VII. Intravenous horseradish peroxidase diffusion across the blood–retinal barrier. Arch Ophthalmol. 1984;102:752–756.
50 Fatt I, Shantinath K. Flow conductivity of retina and its role in retinal adhesion. Exp Eye Res. 1971;12:218–226.
51 Foulds WS. Do we need a retinal pigment epithelium (or choroid) for the maintenance of retinal apposition? Br J Ophthalmol. 1985;69:237–239.
52 Maurice DM, Salmon J, Zauberman H. Subretinal pressure and retinal adhesion. Exp Eye Res. 1971;12:212–217.
53 deGuillebon H, Zauberman H. Experimental retinal detachment: biophysical aspects of retinal peeling and stretching. Arch Ophthalmol. 1972;87:545–548.
54 Hammer ME, Peters H, Wu W. Basic mechanical properties of retina in simple elongation. Invest Ophthalmol Vis Sci. 1987;28(suppl.):116.
55 Zauberman H, deGuillebon H, Holly FJ. Retinal traction in vitro: biophysical aspects. Invest Ophthalmol. 1972;11:46–55.
56 Zauberman H, deGuillebon H. Retinal traction in vivo and postmortem. Arch Ophthalmol. 1972;87:549–554.
57 Bloch D, O’Connor P, Lincoff H. The mechanism of the cryosurgical adhesion. III. Statistical analysis. Am J Ophthalmol. 1971;71:666–673.
58 Yoon YH, Marmor MF. Rapid enhancement of retinal adhesion by laser photocoagulation. Ophthalmology. 1988;95:1385–1388.
59 Lira RP, Takasaka I, Arieta CE, et al. Cryotherapy vs laser photocoagulation in scleral buckle surgery: A randomized clinical trial. Arch Ophthalmol. 2010;128:1519–1522.
60 Oster SF, Mojana F, Freeman WR. Spectral-domain optical coherence tomography imaging of postoperative scleral buckles. Retina. 2011;31:1493–1499.
61 Figueroa MS, Corte MD, Sbordone S, et al. Scleral buckling technique without retinopexy for treatment of rhegmatogenous: a pilot study. Retina. 2002;22:288–293.
62 Mahdizadeh M, Masoumpour M, Ashraf H. Anatomical retinal reattachment after scleral buckle with and without retinopexy: a pilot study. Acta Ophthalmol. 2008;86:297–301.
63 Foster WJ, Dowla N, Joshi SY, et al. The fluid mechanics of scleral buckling surgery for the repair of retinal detachment. Graefes Arch Clin Exp Ophthalmol. 2010;248:31–36.
64 Foulds WS. The vitreous in retinal detachment. Trans Ophthalmol Soc UK. 1975;95:412–416.