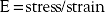Forces

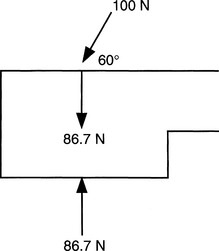
The International System (SI units), which is based on the metric system, is the nomenclature used by the biomedical engineering profession. The newton (N) is a direct measure of force and is recorded as intrinsic units: kg(m)/sec². As defined by Newton’s second law, force is equivalent to the product of mass and acceleration. Forces, when applied to the spine, not only consist of a magnitude but also have a directional component. The combination of a force with direction is a vector. Vectors can be displayed graphically or by trigonometric relationships. Vectors can be used to analyze biomechanical forces acting simultaneously on a biologic structure or implant material by making a free body diagram that assumes a state of equilibrium, thereby defining the forces inside the structure or implant material as dependent and proportional to those outside the structure (Fig. 20-1).

FIGURE 20-1 Free body diagram of a lumbar vertebra with an externally applied load of 100 N at an incident angle of 60 degrees. The vertebra will have to resist the shear component as well (horizontal component—not shown in the figure—of the incident force). The resultant downward force on the vertebral body is sin (60) × 100 N = 86.7 N. The vertebra is in equilibrium with its surroundings and not moving. Therefore, a force of the same magnitude is acting on the caudal end plate.

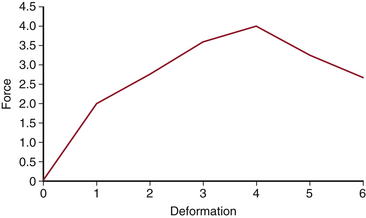
A very important principle for the spine surgeon to understand is the force-deformation relationship (Fig. 20-2). When force and deformation are graphically displayed, the result is a characteristic curve. The force-deformation curve has a straight or elastic region in which materials can deform and recover to their original shape (see Fig. 20-2, first portion of curve). As the load increases beyond the elastic region, the deformation increases into the curved or plastic region (see Fig. 20-2, second portion of curve); when the specimen is unloaded, it will be permanently deformed. If deformation is continued, the specimen will eventually fail (e.g., fracture; see Fig. 20-2, third portion of curve).

FIGURE 20-2 Force versus deformation curve. The force has a straight or elastic region in which materials can deform and recover to their original shape. As load increases beyond the elastic region, the deformation increases into the plastic region; when the specimen is unloaded, it will be permanently deformed. If the deformation is limited, the specimen eventually will fail (i.e., fracture).

The integrity of the spine is multifactorial. The vertebral body ossifies from three primary centers, one for the centrum, which will form the major portion of the body, and the other two for neural arches. The cartilaginous growth plate is mainly responsible for longitudinal vertebral growth. The vertebral body design, therefore, provides the requirement for optimal load transfer by maximal strength with minimal weight. Bone mineral density (BMD), bone quality, microarchitecture, and material properties are the important factors that contribute to bone strength.³ In addition, force and displacement have been demonstrated in animal spine models. It has been demonstrated in a biomechanical cadaver study that after dorsal laminectomy and partial discectomy, the neutral zone and range of motion were not different from those in the native spine specimen. However, after pedicle screw-rod fixation, the neutral zone and range of motion of the instrumented specimen decreased significantly compared with the native specimen and the specimen after dorsal laminectomy.⁴

Atomic Bonds, Structures, and Property Relationships

All materials are composed of molecules that interact via intermolecular forces. These bonds determine the properties of the material as a whole. If materials were composed of only one type of molecule and these molecules were perfectly consistent in their orientation, then chemistry alone would be sufficient for deriving all of the elements’ properties. However, materials typically are composed of numerous molecules of considerable diversity. Nevertheless, despite the variety of molecules in metals, certain observations can be made from their chemical composition.

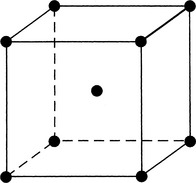
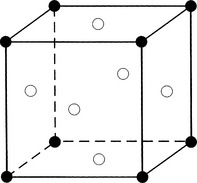
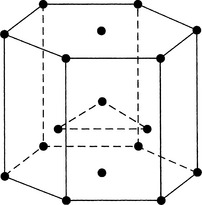
Metals are created through the interaction of crystals. These crystals are formed when the electrons that surround the atoms in clouds are given up and conducted as electricity. Metal structures are polycrystalline (i.e., they are formed by a multitude of crystals). Atoms within a crystal can form one of several relationships, which define the crystal structure. They include body-centered cubic, face-centered cubic, and hexagonal close-packed arrangements (Figs. 20-3 to 20-5).

FIGURE 20-3 The unit cell is the smallest group of atoms showing a characteristic structure. The body-centered cube is the most ductile.

FIGURE 20-4 The face-centered cube is moderately ductile.

FIGURE 20-5 The hexagonal close-packed cube is the least ductile.

In addition to variations in the unit cell of the crystal, metals have many imperfections in the crystals, consisting of line defects, point defects, missing atoms, additional atoms, and impurities with foreign atoms. Metals can be further contaminated with larger impurities from nonmetallic elements such as oxides and sulfides.

Point defects occur when a lattice site within a crystal is empty and not occupied by an atom.^1,5 Point defects are present in all metals and provide a mechanism for diffusion, which is the movement of solute through a solvent.

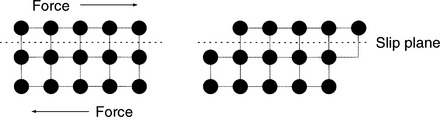
Line defects are microscopic dislocations and are the major defect affecting a given metals mechanical properties. Line defects occur when there is an incomplete chain of atoms inside a crystal. This results in a local distortion of the structure of the crystal because of the resultant dislocation. There is considerable internal strain in the immediate vicinity of the dislocation. When a force is applied, the line defect can propagate through the crystal structure, resulting in a permanent structural change (Figs. 20-6 and 20-7). This is termed plastic deformation. When a metal is plastically deformed, a permanent structural change persists after the force is removed from the metal.¹

FIGURE 20-6 During stress, individual atomic bonds are disrupted and the atoms slip along a plane.

FIGURE 20-7 Deformation occurs when parallel and opposite forces are applied to a structure with one side immobilized.

An example of an area defect is a grain boundary.¹ When metal begins the solidification process, crystals form independently of one another. Each crystal grows into a crystalline structure, or grain. The size and number of grains developed by a certain amount of metal depend on the rate of nucleation, which is the initial stage of formation of a crystal. Rapid cooling usually produces smaller grains, whereas slower cooling produces larger grains. The orientation of crystal boundaries (grain boundaries) is very influential in the spread of dislocations that become cracks.

A high nucleation rate yields a high number of grains for a given amount of metal. Therefore, the grain size will be small. If the rate of growth of the crystals is high relative to their nucleation rate, however, fewer grains will develop, and they will be of larger size.

As a grain grows, it eventually comes in contact with another grain. The surfaces that separate grains are termed grain boundaries. Grain boundaries are the junction areas of the many metal crystals that compose an implant. The grain size has a significant effect on the mechanical properties of a metal. A higher number of grain boundaries increases strength. Grain boundaries prevent line defects from propagating from one grain to another. A higher number of grain boundaries necessitates a higher force required to induce a plastic deformation. Since a higher number of grain boundaries occurs in alloys with smaller grains, smaller grains yield an increase in strength, whereas larger grains are generally associated with low strength and ductility.

The many ways in which a metal can acquire defects affecting its strength has led to the development of various strengthening mechanisms to improve the performance of a metal or alloy. All strengthening mechanisms act on the theory that impeding line defects results in increased strength.

Solid solution strengthening occurs when one or more elements are added to a metal. Atoms of the solute will take places within the crystalline lattice by substituting for a solvent (metal) atom. Alternatively, the solute atom may occupy a site not previously occupied by a solvent atom by lying in an interstitial site. Interstitial atoms usually are much smaller than the solvent, whereas substituting elements often are similar in size to the solvent. Interstitial solid solution strengthening often is more effective. The effect of solid solution strengthening is to stop line defects from spreading a dislocation by developing solute-rich regions in the area surrounding the line defect. As a result, increased force is needed to induce a plastic deformation.

Cold working deforms the metal and results in an increase in strength. Deformation of a metal increases the amount of line defects within the metal. These dislocations then entangle with one another. The result is an increasing amount of energy that continues to move these line defects within the grain. The increase in strength from cold working comes at the expense of a decrease in ductility.

Hot working involves the use of high temperature to deform the metal. This often is used to allow a metal to form a shape while altering the microstructure of the alloy. It is possible to obtain a reduction in grain size by hot working. By increasing the temperature to a level that causes a deformation, the dislocations become disentangled. The metal then undergoes recrystallization, and new dislocation-free grains are formed.

Mechanical Properties

Knowing the dimensions of a material, when a force is applied, permits the stress or load per unit area to be determined. Stress is recorded as N/m² (Pascal) and is a small quantity. Therefore, most materials are tested with thousands of N/m², or megapascals. Strain is a dimensionless unit that is the percentage of elongation (or shortening) during application of force. When both the load and the deformation are divided by the original area or length of the specimen, respectively, the result is stress and strain, which can be displayed graphically (see Fig. 20-7).

Spine surgeons should have a basic understanding of the typical stress-strain curve (see Fig. 20-2). The stress-strain curve defines the mechanical behavior of a metal under various degrees of stress and strain. The ratio of stress to strain is the modulus, or elastic modulus. The relationship is as follows:

The modulus (E) reflects the stiffness of the material. Stiffness, in turn, depends on the relative difficulty of stretching atoms from their resting position in a crystal lattice. It is important to note that the modulus is not affected to a significant degree by line defects.

At a certain amount of stress, plastic deformation occurs. At this point, line deformations begin to cause structurally relevant deformations, which propagate through the grain. The linear relationship between stress and strain breaks down, and the slope of the modulus decreases. The point at which this occurs is the proportional limit. Yield stress is arbitrarily defined as the point at which permanent deformation reaches 0.2% of the metal. Ultimate stress is defined as the highest stress reached during testing of the metal. Percent elongation, a measure of ductility, is the degree of plastic deformation acquired prior to failure.

Fatigue strength is another property of metal that is important when considering bioimplantation. Fatigue is a process whereby repetitive stress or strain is applied to a metal, eventually leading to breakdown, crack formation, and eventual failure of the metal. By definition, the fatigue strength is the cyclic stress required to cause failure of the metal at a given number of cycles. When a metal fails from fatigue, it usually takes much longer and a greater number of cycles to form the initial crack than to achieve complete failure. Consequently, any material that acts to prevent crack formation will improve the metal’s resistance to fatigue.

Fatigue failures have become less common in implants because of improved materials and strengthening processes. When they do occur, crack formation usually is the inciting factor. Most of these cracks form at the alloy surface. Therefore, surface conditions become very important in preventing fatigue failure.

Spinal Implants: Rigid versus Dynamic

Spinal implants can be described as rigid, dynamic, or hybrid. Dynamic implants allow some subsidence between segments. The advantage of a dynamic implant is that it is capable of offsetting stress at the implant-bone interface and therefore does not provide stress shielding of the bone graft.

The purpose of a rigid construct is to completely immobilize the spine. Because of the properties of bone, this is rarely achieved. Movement in a rigid system often increases with the passage of time, through weakening of the implant-bone interface. Repetitive movement under sufficient stress eventually will lead to failure at the interface, unless bony fusion occurs first.

Rigid fixation does not completely optimize bony fusion acquisition because of stress shielding. The goal of rigid fixation is only to hold long enough for bony fusion to take place.

The widespread use of instrumentation in the lumbar spine has led to high rates of fusion. This has been accompanied by a marked rise in adjacent-segment disease, which is considered to be an increasingly common and significant consequence of lumbar or lumbosacral fusion. Numerous biomechanical studies have demonstrated that segments fused with rigid metallic fixation lead to significant amounts of supraphysiologic stress on adjacent discs and facets. Although this form of arthrodesis does not completely prevent adjacent-segment disease, the dynamic component of this stabilization technique may minimize its occurrence.⁶ Posterior dynamic stabilization (PDS) devices have shown a substantial reduction in stress-shielding characteristics. Higher axial load was noted with the PDS devices, which could slow the degeneration process of bony structures and lower the possibility of implant failure.⁷

The purpose of a dynamic construct is to provide for intersegmental subsidence. Although excessive movement can inhibit fusion, the minimal intersegmental movement (which facilitates compression) increases the rate of bone fusion. Also, the minimal intersegmental movement absorbs some of the strain that is encountered at the implant-bone interface.

Biologic Materials

Bone is the “gold standard” of implant materials as a biologic material. It consists of a framework of type I collagen fibers, a matrix of calcium hydroxyapatite, and small amounts of protein polysaccharides and mucopolysaccharides (ground substance or cement). The organic content of bone is relatively constant at 0.6 g/mL, whereas the mineral content varies (up to 2 g/mL). Bone is slightly viscoelastic, in that rapid deformations result in 95% of the eventual displacement caused by slow deformations. Because of this difference, the energy required for fracture is higher under rapid loading conditions. Under very rapid (ballistic) conditions, bone shatters into comminuted fragments. The total energy required to create a fracture is thus reduced. Bone is anisotropic, with a fiber pattern that is parallel to the predominant axis of loading. It displays both elastic and plastic behavior.

The stiffness of bone is approximately 10% that of stainless steel and 20% that of titanium alloy (Ti-6Al4V, or simply 116-4). This means that the stiffness of bone is closer to that of titanium than steel implants. This fact has been used to suggest that there is a better “match” between titanium and bone than between stainless steel and bone. In certain circumstances this might be important, such as when a permanently implanted device will be subjected to repeated deformations while the bone-implant juncture could loosen. Alternatively, the use of steel implants creates a construct of higher stiffness. Surgical constructs of higher stiffness have been associated with higher fusion rates, both clinically and experimentally. Because the optimal stiffness of a surgical construct for bone healing is unknown, the selection of implant material should not be influenced by minor variations in the stiffness of materials.

Stainless Steel

Stainless steel implants are iron- and carbon-based alloys. Medical grade stainless steel alloys typically contain chromium (18–22%), nickel (18–22%), molybdenum (2.5%), manganese (2.5%), and carbon (0.03–0.08%) by weight.

Initial trials of stainless steel as an implant showed that resisting corrosion by improving resistance to chloride degradation was insufficient. The addition of molybdenum and chromium reduced the incidence of corrosion and pitting by aiding the defense against chloride degradation.^1,5

The 316 stainless steels, which have a face-centered cubic structure, are the steels that are used most commonly for spinal implants. There are two grades, grade 1 (316) and grade 2 (316L), of which grade 2 has the lower carbon content. The lower carbon content aids in reducing the formation of metal carbides, which leads to a decrease in the corrosion resistance of the alloy.^1,8 These 316 alloys are commonly used in fracture fixation devices. They are commonly hot- or cold-worked and solid solution–strengthened.

Titanium-Based Alloys

Titanium-based alloys currently are the alloys most commonly used for bioimplantation. Pure titanium (cP-Ti) and an alloy with aluminum and vanadium (Ti-6Al-4V) are the most common compositions for titanium in the United States.^1,5

Titanium-based alloys are advantageous for several reasons. They have both high strength and fatigue resistance. Commercially pure titanium has a hexagonal, close-packed structure; various grades differ in their oxygen concentration. In small quantities, oxygen serves to solid-solution strengthen the alloy by interstitial placement of the atom. However, in excessive amounts, oxygen can weaker the material by decreasing the number of grain boundaries. This results in a much lower fatigue strength and surface ductility.^1,5,8 Titanium-based alloys also have decreased stiffness when compared to stainless steel. The reduction in stiffness facilitates transfer of the stress at the bone-implant interface to the alloy; this minimizes bone resorption at the interface. Titanium-based alloys have higher fatigue strength when compared with stainless steel. However, titanium alloys are vulnerable to any surface flaws. Any scratch or notch can rapidly accelerate the fatigue failure process. Titanium, in its pure form, is generally weaker than stainless steel, but it can be cold-worked to increase its strength.^1,2 Titanium alloys also lack any known immunogenicity, an important advantage for any foreign body implant.

Surface Structure and Modifications of Alloys

As discussed previously, any surface component that prevents crack formation will decrease an alloy’s sensitivity to fatigue. Implant alloys typically use an oxide film for their surfaces. These oxide films are considered passive films because they are the result of oxidation of the outermost metal atoms on the surface of the alloy.¹

Corrosion is an oxidative process that is a threat to alloys. Corrosion would ensue rapidly without passive films. Stainless steels have oxide films composed of Cr₂O₃, FeO, and Fe₂O₃. This thin film separates the metal from its surrounding, corrosive environment and is the major factor in resistance to decay. Titanium alloys form TiO₂, which plays a similar role to that of chromium oxide with stainless steel. Oxide films also serve as a protective barrier on articulating surfaces of the alloy.

Immersion of steel alloy surfaces in nitric acid baths also delays corrosion. Nitric baths dissolve impurities anywhere they exist on the surface of the metal implants, thereby ensuring an intact oxide film.

Surface modification is used to increase local strength and hardening of implants. Two types of surface modification include ion implantation and vapor deposition.^1,5 Ion implantation, the direct implantation of gas phase ions into the alloy, involves the acceleration of ions toward the surface of the alloy. The ions penetrate and increase the number and type of subsurface defects and dislocations, which leads to an increase in the durability of the metal surface and a reduction in its susceptibility to corrosion.^1,9

Another method of surface modification is either chemical or physical vapor deposition. This technique adds a new hardened coating to the alloy surface, usually composed of chromium nitride (CrN) or titanium nitride (TiN). One shortcoming of hardened coatings is that they have variable adhesion with the alloy.

Finally, nitriding is a process that modifies the surface of a material by a chemical reaction with nitrogen, which places nitrides on the surface. A portion of the surface of stainless steel or titanium can undergo nitriding by causing the surface to react with either gaseous ammonia or molten potassium cyanate.^1,5 The result is a great increase in surface hardness of the alloy.

Ceramics

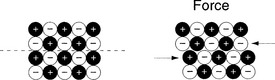
In contrast to metals, ceramics have chemical bonds that are predominantly ionic, with a densely packed array of oppositely charged atoms (Fig. 20-8). These atoms have limited mobility because of the interaction of charges of nearby atoms. This charge interaction results in a stiff material with low ductility and no plasticity. Ceramics are composed of crystals oriented randomly in a dense framework that consists of metallic oxides. They may have inorganic chain molecules, such as silicon dioxide in a glass phase. Traditional ceramic materials often have impurities and internal microporous inclusions that limit their strength to less than the theoretical maximum. Newer ceramics are synthesized with chemically pure materials with high densities or as composites with greatly increased strength.^10–12

FIGURE 20-8 Ceramics have strong ionic bonds and these charge interactions result in a stiff material with low ductility and no plasticity.

An advantage of oxide ceramics is their wear reduction compared to that of alloys.^1,10 Metals and alloys have a protective oxide film that can be peeled off by adherence to opposing surface polymers.^1,10 This causes local ion release from the alloy. This loss and reformation of the oxide film is a repetitive process that can accelerate degradation of the surface of the alloy implant. Oxide ceramics do not have a passive oxide film and may, as a result, have less long-term breakdown.

Synthetic Polymers

Synthetic polymer production is a rapidly expanding field of implant technology. Polymers, commonly known as plastics, typically are very large molecules made from a large number of individual subunits called monomers. Polymers are chemical compounds that are formed by combining these smaller, repeating structural units. The subunits repeat in various patterns, following principles similar to those of molecular biology. The covalent bonds in polymers have a fixed length. The complex folding of polymers is created by weak hydrogen bond cross-links that permit unfolding and elongation. The two most commonly used polymers are polymethylmethacrylate (PMMA) and ultra-high molecular weight polyethylene (UHMWPE).¹

Stiffening the “backbone” molecular chain and increasing the cross-links, the polymer can be made less flexible. Numerous other properties can be influenced by chemical changes, including density, crystallization, solubility, thermal stability, and strength. UHMWPE has been extensively used for artificial joints because of its favorable surface wear and creep properties. In spine surgery, PMMA has been used extensively because of the additional polymerization that occurs when the powder and liquid are mixed.

The intermediate phase of polymerization yields a doughy material that can be worked and shaped into complex defects before it hardens. PMMA has many molecular and macroscopic defects that contribute to its characteristically weak tensile strength. These defects originate in the powder phase, which consists of microspheres. The microspheres are bound together as the methylmethacrylate monomer (liquid phase) polymerizes into a matrix that incorporates the microspheres. Even after hardening, the juncture between the powder phase microspheres and the liquid phase remains relatively weak. Additionally, the polymer chains have very few cross-links. For all these reasons, the polymerized PMMA has a low tensile strength.

Composite materials are a combination of a filler and matrix. Traditionally, the filler is glass or carbon fibers, whereas the matrix is epoxy, carbon, UHMWPE, PMMA, or a variety of other materials. The fibers can be particulate or relatively large and stiff, in which case they are termed whiskers. Composites with whiskers have high tensile strength but can be brittle. Fiber orientation in relation to the direction of loading is important. A complex variety of stress responses can be obtained in polymer matrix composites, making these materials anisotropic. Biologic materials such as bone, ligament, and tendon also are composites with anisotropy. An example of a composite used in spine surgery is the carbon fiber cage for interbody fusion, which is composed of long-fiber carbon and Ultrapek (polyether ketone; BASF Aktiengesellschaft, Germany).¹³

Bone Morphogenetic Protein

Bone morphogenetic protein (BMP) is a group of proteins of the transforming growth factor beta (TGF-β) family that can induce bone growth. Currently, they are synthesized using recombinant DNA and work via signal transduction through BMP receptors. Of the several BMPs, the most commonly used is BMP-2, a disulfide-linked homodimer that induces bone and cartilage formation, playing a key role in osteoblast differentiation and making it a useful adjunct in spinal fusion.

The use of growth factors such as BMPs is showing great promise in spinal surgery and has been used successfully in spine fusion and fracture healing.¹⁴ Several animal models have demonstrated that BMP-containing allograft or synthetic carrier medium is as effective as or superior to autograft bone in promoting spinal fusion.¹⁵ Burkus et al. have illustrated that in ventral lumbar fusions, allograft cortical bone with rhBMP had equivalent fusion rates as autogenous bone graft without the associated graft site morbidity.¹⁶

Recombinant human bone morphogenetic protein type 2 (rhBMP-2) has been demonstrated to be safe and effective in posterior lumbar interbody fusion procedures when used as an absorbable collagen sponge carrier as an alternative to iliac crest bone graft.¹⁷ The efficacy of rhBMP-2 in augmenting fusion also has been shown when used in non-instrumented posterior lumbar decompressive surgery and leads to satisfactory outcomes, specifically improved pain relief, function, and bone formation, in elderly patients without the use of instrumentation.¹⁸ In addition to lumbar surgery, a prospective nonrandomized study in patients undergoing anterior cervical discectomy and fusion has demonstrated that those performed with BMP allograft are as effective as iliac bone graft in terms of patient outcomes and fusion rates.¹⁹

Biocompatibility

All surgical procedures are associated with a disruption of normal anatomic tissue planes. This results in an accumulation of exudative fluid, fibrin, platelets, and polymorphonuclear leukocytes. From days 3 to 5 after surgery, macrophages accumulate and remove the surgical debris. By 10 days, the macrophages are no longer present, and lymphocytes predominate. This stage is followed by fibroblasts, which complete the cellular phase of healing. Ceramic implants are very biocompatible, because the cellular response to wound healing is not significantly altered.

However, in the presence of a metal implant, the immune system is activated, with the production of protein-metal hapten complexes, complement activation, and the resultant cellular and humoral immune responses. A chronic inflammatory state with sustained populations of macrophages, lymphocytes, and occasional plasma cells persists for several weeks or months. Eventually, as the inflammatory response subsides, these foreign bodies are sequestered by dense fibrous tissue. Direct apposition of bone to an implant, without an interposed fibrous layer, is very rare, with the exception of titanium and bioactive ceramics such as calcium hydroxyapatite.

In total joint replacement, wear debris accumulates as a function of the force across the joint, the relative displacement of the articular surfaces, and a variety of wear mechanisms, including abrasion, corrosion, fretting (micromotion), and third-body wear resulting from wear debris between the articular surfaces. Wear debris also can cause adverse local responses to the implant, such as osteolysis and regional lymphadenopathy. These phenomena are of increasing interest to neurosurgeons, because articulating artificial discs have become more widely used as an alternative to spinal fusion.^20–22

For most surgical constructs, stainless steel implants are sufficiently nonreactive to permit bone fusion before the deleterious consequences of the normal inflammatory response, such as severe pain or loosening. The presence of a metal implant may lead to an increased risk of infection. In vitro testing of stainless steel and cobalt alloy materials has shown inhibition of macrophage chemotaxis and phagocytosis, which may contribute to the increased risk of infection. The avascular fibrous layer that accumulates around metal implants in bone also may contribute to this risk. Sites associated with PMMA are especially vulnerable, most likely due to the 0.1- to 0.5-mm layer of necrotic bone that is created by the direct toxicity of the methacrylate monomer and the heat released during hardening.

The fibrous layer around implants may be associated with painful late loosening of the device. For example, after an intertransverse lumbar fusion has occurred, micromotions may persist between the vertebral bodies, resulting in painful movements of vertebral bone in relation to pedicle screws. In theory, bone in-growth and direct adhesion to an implant (such as what occurs with titanium) may lessen the risk of infection and painful late loosening.

Metal allergy is widely prevalent and well recognized, but is poorly understood. Metal ions alone will not stimulate the immune system. When linked with proteins, metals such as cobalt, chromium, and especially nickel are immunogenic. The characteristic immune response to metals is delayed hypersensitivity. This has been proposed as the cause of premature loosening in total hip arthroplasties. Delayed development of sensitivity after prolonged exposure to a metal implant has been well documented, using the leukocyte migration inhibition test.²³ The clinical significance of metal allergy in spinal surgery is unknown, but these data suggest that patients reporting skin sensitivity to metal should be considered for titanium implants rather than stainless steel before elective spinal surgery.

The mechanical strength immediately after instrumentation is determined by the worst case of device-graft subsidence; after instrumentation, bone will adapt itself to the changed loading conditions and therefore reduce the risk of subsidence.²⁴ Knoller et al. demonstrated a relationship between the stability of a spinal instrumentation and bone mineral density and a significant influence of bone mineral density on range of motion.²⁵

Osteolysis, or periprosthetic bone loss, may occur at the site of an implant. Structural remodeling of surrounding bone occurs in response to stress shielding. This bone destruction can lead to loosening and possible failure of the implant. Factors that are thought to play a role in osteolysis include the formation of particulate debris and loosening, or motion, of the implant.⁶ Once the particles are generated, macrophages proliferate and attack the periprosthetic space. This leads to activation of an inflammatory cascade and the induction of osteoclastic pathways.

Summary

Contemporary spinal surgeons are faced with the task of making informed decisions regarding the choice of implant for stabilization. The spinal surgeon must have an understanding of the physical and chemical properties of any material that he or she is contemplating implanting into the patient. Various advantageous properties of alloys, and biomaterials in general, include strength, ductility, modulus, hardness, and biocompatibility. Each material possesses different advantages and disadvantages. It is up to the surgeon to understand this increasingly complex aspect of the field and to make decisions accordingly.