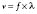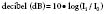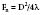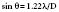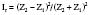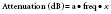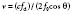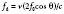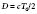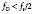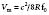Properties of Ultrasound

In echocardiography, the heart and great vessels are insonated with ultrasound, which is sound above the human audible range. The ultrasound is sent into the thoracic cavity and is partially reflected by the cardiac structures. From these reflections, distance, velocity, and density of objects within the chest are derived.

An ultrasound beam is a continuous or intermittent train of sound waves emitted by a transducer or wave generator. It is composed of density or pressure waves and can exist in any medium with the exception of a vacuum (Figure 12-1). Ultrasound waves are characterized by their wavelength, frequency, and velocity.¹ Wavelength is the distance between the two nearest points of equal pressure or density in an ultrasound beam, and velocity is the speed at which the waves propagate through a medium. As the waves travel past any fixed point in an ultrasound beam, the pressure cycles regularly and continuously between a high and a low value. The number of cycles per second (Hertz) is called the frequency of the wave. Ultrasound is sound with frequencies above 20,000 Hz, which is the upper limit of the human audible range. The relationship among the frequency (f), wavelength (λ), and velocity (v) of a sound wave is defined by the following formula:

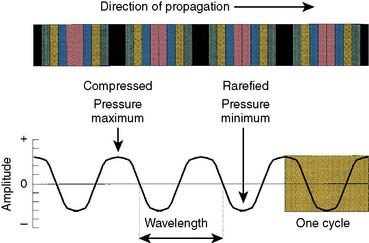
Figure 12-1 A sound wave is a series of compressions and rarefactions.

The combination of one compression and one rarefaction represents one cycle. The distance between the onset (peak compression) of one cycle and the onset of the next is the wavelength.

(From Thys DM, Hillel Z: How it works: Basic concepts in echocardiography. In Bruijn NP, Clements F [eds]: Intraoperative use of echocardiography. Philadelphia: JB Lippincott, 1991.)

The velocity of sound varies with the properties of the medium through which it travels. In low-density gases, molecules must transverse long distances before encountering the adjacent molecules, so ultrasound velocity is relatively slow. In contrast, in solid, where molecules are constrained, ultrasound velocity is relatively high. For soft tissues, this velocity approximates 1540 m/sec but varies from 1475 to 1620 m/sec. In comparison, the velocity of ultrasound in air is 330 m/sec and 3360 m/sec in bone. Because the frequency of an ultrasound beam is determined by the properties of the emitting transducer, and the velocity through soft tissue is approximately constant, wavelengths are inversely proportional to the ultrasound frequency.

Ultrasound waves transport energy through a given medium; the rate of energy transport is expressed as “power,” which is usually expressed in joules per second or watts.¹ Because medical ultrasound usually is concentrated in a small area, the strength of the beam usually is expressed as power per unit area or “intensity.” In most circumstances, intensity usually is expressed with respect to a standard intensity. For example, the intensity of the original ultrasound signal may be compared with the reflected signal. Because ultrasound amplitudes may vary by a factor of 10⁵ or greater, amplitudes usually are expressed using a logarithmic scale. The usual unit for intensity comparisons is the decibel, which is defined as:

where I₁ is the intensity of the wave to be compared and I₀ is the intensity of the reference waves.

Notably, positive values imply a wave of greater intensity than the reference wave, and negative values indicate a lower intensity. Increasing the wave’s intensity by a factor of 10 adds 10 dB to the decibel measurement and doubling the intensity adds 3 dB.

Ultrasound Beam

Piezoelectric crystals convert between ultrasound and electrical signals. Most piezoelectric crystals that are used in clinical applications are the man-made ceramic ferroelectrics, the most common of which are barium titanate, lead metaniobate, and lead zirconate titanate. When presented with a high-frequency electrical signal, these crystals produced ultrasound energy; conversely, when they are presented with an ultrasonic vibration, they produce an electrical alternating current signal. Commonly, a short ultrasound signal is emitted from the piezoelectric crystal, which is directed toward the areas to be imaged. This pulse duration is typically 1 to 2 microseconds. After ultrasound wave formation, the crystal “listens” for the returning echoes for a given period and then pauses before repeating this cycle. This cycle length is known as the “pulse repetition frequency” (PRF). This cycle length must be long enough to provide enough time for a signal to travel to and return from a given object of interest. Typically, PRF varies from 1 to 10 kHz, which results in 0.1 to 1 millisecond between pulses. When reflected ultrasound waves return to these piezoelectric crystals, they are converted into electrical signals, which may be appropriately processed and displayed. Electronic circuits measure the time delay between the emitted and received echo. Because the speed of ultrasound through tissue is a constant, this time delay may be converted into the precise distance between the transducer and tissue. The amplitude or strength of the returning ultrasound signal provides information about the characteristics of the insonated tissue.

The 3D shape of the ultrasound beam is dependent on both physical aspects of the ultrasound signal and the design of the transducer. An unfocused ultrasound beam may be thought of as an inverted funnel, where the initial straight columnar area is known as the “near field” (also known as Fresnel zone) followed by a conical divergent area known as the “far field” (also known as Fraunhofer zone). The length of the “near field” is directly proportional to the square of the transducer diameter and inversely proportional to the wavelength; specifically,

where F_n is the near-field length, D is the diameter of the transducer, and λ is the ultrasound wavelength. Increasing the frequency of the ultrasound increases the length of the near field. In this near field, most energy is confined to a beam width no greater than the transducer diameter. Long Fresnel zones are preferred with medical ultrasonography, which may be achieved with large-diameter transducers and high-frequency ultrasound. The angle of the “far-field” convergence (θ) is directly proportional to the wavelength and inversely proportional to the diameter of the transducer and is expressed by the equation:

Further shaping of the beam geometry may be adjusted using acoustic lenses or the shaping of the piezoelectric crystal. Ideally, imaging should be performed within the “near-field” or focused aspect of the ultrasound beam because the ultrasound beam is most parallel with the greatest intensity and the tissue interfaces are most perpendicular to these ultrasound beams.

Attenuation, Reflection, and Scatter

Waves interact with the medium in which they travel and with one another. Interaction among waves is called interference. The manner in which waves interact with a medium is determined by its density and homogeneity. When a wave is propagated through an inhomogeneous medium (and all living tissue is essentially inhomogeneous), it is partly reflected, partially absorbed, and partly scattered.

Ultrasound waves are reflected when the width of the reflecting object is larger than one fourth of the ultrasound wavelength. Because the velocity of sound in soft tissue is approximately constant, shorter wavelengths are obtained by increasing the frequency of the ultrasound beam (see Eq. 1). Large objects may be visualized using low frequencies (i.e., long wavelengths), whereas smaller objects require higher frequencies (i.e., short wavelength) for visualization. In addition, the object’s ultrasonic impedance (Z) must be significantly different from the ultrasonic impedance in front of the object. The ultrasound impedance of a given medium is equal to the medium density multiplied by the ultrasound propagation velocity. Air has a low density and propagation velocity, so it has a low ultrasound impedance. Bone has a high density and propagation velocity, so it has a high ultrasound impedance. For normal incidence, the fraction of the reflected pulse compared with the incidence pulse is:

where I_r is intensity reflection coefficient, and Z₁ and Z₂ are acoustical impedance of the two media.

The greater the differences in ultrasound impedance between two objects at a given interface, the greater the ultrasound reflection. Because the ultrasound impedances of air or bone are significantly different from blood, ultrasound is strongly reflected from these interfaces, limiting the availability of ultrasound to deeper structures. Echo studies across lung or other gas-containing tissues or across bone are not feasible. Reflected echoes, also called “specular echoes,” usually are much stronger than scattered echoes. A grossly inhomogeneous medium, such as a stone in a water bucket or a cardiac valve in a blood-filled heart chamber, produces strong specular reflections at the water–stone or blood–valve interface because of the significant differences in ultrasound impedances. Furthermore, if the interface between the two objects is not perpendicular, the reflected signal may be deflected at an angle and may not return to the transducer for imaging.

In contrast, if the objects are small compared with the wavelength, the ultrasound wave will be scattered. Media that are inhomogeneous at the microscopic level, such as muscle, produce more scatter than specular reflection because the differences in adjacent ultrasound impedances are low and the objects are small. These small objects will produce echoes that reflect throughout a large range of angles with only a small percentage of the original signal reaching the ultrasound transducer. Scattered ultrasound waves will combine in constructive and destructive fashions with other scattered waves, producing an interference pattern known as “speckle.” Compared with specular echoes, the returning ultrasound signal amplitude will be lower and displayed as a darker signal. Although smaller objects can be visualized with higher frequencies, these higher frequencies result in greater signal attenuation, limiting the depth of ultrasound penetration.

Attenuation refers to the loss of ultrasound power as it transverses tissue. Tissue attenuation is dependent on ultrasound reflection, scattering, and absorption. The greater the ultrasound reflection and scattering, the less ultrasound energy is available for penetration and resolution of deeper structures; this effect is especially important during scanning with higher frequencies. In normal circumstances, however, absorption is the most significant factor in ultrasound attenuation.² Absorption occurs as a result of the oscillation of tissue caused by the transit of the ultrasound wave. These tissue oscillations result in friction, with the conversion of ultrasound energy into heat. More specifically, the transit of an ultrasound wave through a medium causes molecular displacement. This molecular displacement requires the conversion of kinetic energy into potential energy as the molecules are compressed. At the time of maximal compression, the kinetic energy is maximized and the potential energy minimized. The movement of molecules from their compressed location to their original location requires conversion of this potential energy back into kinetic energy. In most cases, this energy conversion (either kinetic into potential energy or vice versa) is not 100% efficient and results in energy loss as heat.¹

The absorption is dependent both on the material through which the ultrasound is passing and the ultrasound frequency. The degree of attenuation through a given thickness of material, x, may be described by:

where a is the attenuation coefficient in decibels (dB) per centimeter at 1 MHz, and freq represents the ultrasound frequency in megahertz (MHz).

Examples of attenuation coefficient values are given in Table 12-1. Whereas water, blood, and muscle have low ultrasound attenuation, air and bone have very high tissue ultrasound attenuation, limiting the ability of ultrasound to transverse these structures. Table 12-2 gives the distance in various tissues at which the intensity or amplitude of an ultrasound wave of 2 MHz is halved (the half-power distance).

TABLE 12-1 Attenuation Coefficients

TABLE 12-2 Half-Power Distances at 2 MHz

M-Mode

The most basic form of ultrasound imaging is M-mode echocardiography. In this mode, the density and position of all tissues in the path of a narrow ultrasound beam (i.e., along a single line) are displayed as a scroll on a video screen. The scrolling produces an updated, continuously changing time plot of the studied tissue section several seconds in duration. Because this is a timed motion display (normal cardiac tissue is always in motion), it is called M-mode. Because only a limited part of the heart is being observed at any one time and because the image requires considerable interpretation, M-mode is not used currently as a primary imaging technique. This mode is, however, useful for the precise timing of events within the cardiac cycle and often is used in combination with CFD for the timing of abnormal flows (see later). Quantitative measurements of size, distance, and velocity also are easily performed in the M-mode without the need for sophisticated analysis stations. Because M-mode images are updated 1000 times per second, they provide greater temporal resolution than two-dimensional (2D) echocardiography; thus, more subtle changes in motion or dimension can be appreciated.

B-Mode

The different reflectivities of various cardiac structures result in variations of the reflected ultrasound wave. The detected signals are translated from the amplitude of the reflected signal to luminance and displayed as a brightness-mode or B-mode image. By rapid, repetitive scanning along many different radii within an area in the shape of a fan (sector), echocardiography generates a 2D image of a section of the heart. This image, which resembles an anatomic section, can be interpreted more easily than an M-mode display. Information on structures and motion in the plane of a 2D scan is updated 20 to 40 times per second. This repetitive update produces a “live” (real–time) image of the heart. Scanning 2D echocardiography (2DE) devices usually image the heart using an electronically steered ultrasound beam (phased–array transducer).

Harmonic Imaging

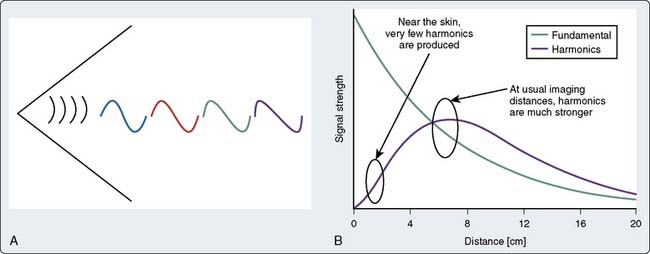
Harmonic frequencies are ultrasound transmission of integer multiples of the original frequency. For example, if the fundamental frequency is 4 MHz, the second harmonic is 8 MHz, the third fundamental is 12 MHz, and so on. Harmonic imaging refers to a technique of B-mode imaging in which an ultrasound signal is transmitted at a given frequency but will “listen” at one of its harmonic frequencies.^3,4 As ultrasound is transmitted through a tissue, the tissue undergoes slight compressions and expansions that correspond to the ultrasound wave temporarily changing the local tissue density. Because the velocity of ultrasound transit is directly proportional to density, the peak amplitudes will travel slightly faster than the trough. This differential velocity transit of the peak with the trough wave results in distortion of the propagated sine wave, resulting in a more peaked wave. This peaked wave will contain frequencies of the fundamental frequency, as well as the harmonic frequencies (Figure 12-2). Although little distortion occurs in the near field, the amount of energy contained within these harmonics increases with ultrasound distance transversed as the ultrasound wave becomes more peaked. Eventually, the effects of attenuation will be more pronounced on these harmonic waves with subsequent decrease in harmonic amplitude. Because the effects of attenuation are greatest with high-frequency ultrasound, the second harmonic usually is used.

Figure 12-2 Harmonic imaging.

A, Because the velocity of ultrasound transit is directly proportional to density, the peak amplitudes will travel slightly faster than the trough. With time, this differential velocity transit of the peak with the trough wave results in distortion of the propagated sin wave resulting in a more peaked wave. B, Relation between imaging distance and strength of fundamental and harmonics frequencies. As ultrasound pulse propagates, strength of fundamental frequency declines, whereas strength of harmonic frequency increases. At usual imaging distances for cardiac structures, strength of harmonic frequency is maximized. Note: Harmonic frequency strength is exaggerated in this schematic. Harmonic frequency signal strength is much lower than fundamental frequency signal strength.

(B, From Thomas JD, Rubin DN: Tissue harmonic imaging: Why does it work? J Am Soc Echocardiogr 11:803–808, 1998, by permission.)

The use of tissue harmonic imaging is associated with improved B-mode imaging. Near-field scatter is common with fundamental imaging. Because the ultrasound wave has not yet been distorted, little harmonic energy is generated in the near field, minimizing near-field scatter when harmonic imaging is used. Because higher frequencies are used, greater resolution may be obtained. Finally, with tissue harmonic imaging, side-lobe artifacts are substantially reduced and lateral resolution is increased.

Doppler Techniques

Most modern echo scanners combine Doppler capabilities with their 2D imaging capabilities. After the desired view of the heart has been obtained by 2DE, the Doppler beam, represented by a cursor, is superimposed on the 2D image. The operator positions the cursor as parallel as possible to the assumed direction of blood flow and then empirically adjusts the direction of the beam to optimize the audio and visual representations of the reflected Doppler signal. Currently, Doppler technology can be utilized in at least four different ways to measure blood velocities: pulsed, high-repetition frequency, continuous wave, and color flow. Although each of these methods has specific applications, they are seldom available concurrently.

The Doppler Effect

Information on blood flow dynamics can be obtained by applying Doppler frequency shift analysis to echoes reflected by the moving red blood cells.^5,6 Blood flow velocity, direction, and acceleration can be instantaneously determined. This information is different from that obtained in 2D imaging, and hence complements it.

The Doppler principle as applied in echocardiography states that the frequency of ultrasound reflected by a moving target (red blood cells) will be different from the frequency of the reflected ultrasound. The magnitude and direction of the frequency shift are related to the velocity and direction of the moving target. The velocity of the target is calculated with the Doppler equation:

where v = the target velocity (blood flow velocity); c = the speed of sound in tissue; f_d = the frequency shift; f₀ = the frequency of the emitted ultrasound; and θ = the angle between the ultrasound beam and the direction of the target velocity (blood flow). Rearranging the terms,

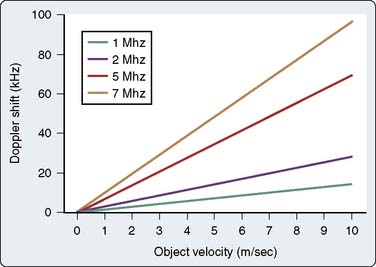
As is evident in Equation 8, the greater the velocity of the object of interest, the greater the Doppler frequency shift. In addition, the magnitude of the frequency shift is directly proportional to the initial emitted frequency (Figure 12-3). Low emitted frequencies produce low Doppler frequency shifts, whereas higher emitted frequencies produce high Doppler frequency shifts. This phenomenon becomes important with aliasing, as is discussed later in this chapter. Furthermore, the only ambiguity in Equation 7 is that theoretically the direction of the ultrasonic signal could refer to either the transmitted or the received beam; however, by convention, Doppler displays are made with reference to the received beam; thus, if the blood flow and the reflected beam travel in the same direction, the angle of incidence is zero degrees and the cosine is +1. As a result, the frequency of the reflected signal will be higher than the frequency of the emitted signal.

Figure 12-3 Graph of Doppler shift frequency versus velocity for various emitted ultrasound frequencies. A lower emitted ultrasound frequency will produce a lower Doppler frequency shift for a given velocity. This lower Doppler frequency shift will allow for a higher velocity measurement before aliasing occurs.

Equipment currently used in clinical practice displays Doppler blood-flow velocities as waveforms. The waveforms consist of a spectral analysis of velocities on the ordinate and time on the abscissa. By convention, blood flow toward the transducer is represented above the baseline. If the blood flows away from the transducer, the angle of incidence will be 180 degrees, the cosine will equal -1, and the waveform will be displayed below the baseline. When the blood flow is perpendicular to the ultrasonic beam, the angle of incidence will be 90 or 270 degrees, the cosine of either angle will be zero, and no blood flow will be detected. Because the cosine of the angle of incidence is a variable in the Doppler equation, blood-flow velocity is measured most accurately when the ultrasound beam is parallel or antiparallel to the direction of blood flow. In clinical practice, a deviation from parallel of up to 20 degrees can be tolerated because this results in an error of only 6% or less.

Pulsed-Wave Doppler

In PW Doppler, blood-flow parameters can be determined at precise locations within the heart by emitting repetitive short bursts of ultrasound at a specific frequency (PRF) and analyzing the frequency shift of the reflected echoes at an identical sampling frequency (f_s). A time delay between the emission of the ultrasound signal burst and the sampling of the reflected signal determines the depth at which the velocities are sampled; the delay is proportional to the distance between the transducer and the location of the velocity measurements. To sample at a given depth (D), you must allow sufficient time for the signal to travel a distance of 2 × D (from the transducer to the sample volume and back). The time delay, T_d, between the emission of the signal and the reception of the reflected signal, is related to D, and to the speed of sound in tissues (c), by the following formula:

The operator varies the depth of sampling by varying the time delay between the emission of the ultrasonic signal and the sampling of the reflected wave. In practice, the sampling location or sample volume is represented by a small marker, which can be positioned at any point along the Doppler beam by moving it up or down the Doppler cursor. On some devices, it is also possible to vary the width and height of the sample volume.

The trade-off for the ability to measure flow at precise locations is that ambiguous information is obtained when flow velocity is very high. Information theory suggests that an unknown periodic signal must be sampled at least twice per cycle to determine even rudimentary information such as the fundamental frequency; therefore, the rate of PRF of PW Doppler must be at least twice the Doppler-shift frequency produced by flow.⁷ If not, the frequency shift is said to be “undersampled.” In other words, this frequency shift is sampled so infrequently that the frequency reported by the instrument is erroneously low.¹

A simple reference to Western movies will clearly illustrate this point. When a stagecoach gets under way, its wheel spokes are observed as rotating in the correct direction. As soon as a certain speed is attained, rotation in the reverse direction is noted because the camera frame rate is too slow to correctly observe the motion of the wheel spokes. In PW Doppler, the ambiguity exists because the measured Doppler frequency shift (f_D) and the sampling frequency (f_s) are in the same frequency (kHz) range. Ambiguity will be avoided only if the f_D is less than half the sampling frequency:

The expression f_s/2 is also known as the Nyquist limit. Doppler shifts above the Nyquist limit will create artifacts described as “aliasing” or “wraparound,” and blood-flow velocities will appear in a direction opposite to the conventional one (Figure 12-4). Blood flowing with high velocity toward the transducer will result in a display of velocities above and below the baseline. The maximum velocity that can be detected without aliasing is dictated by:

Figure 12-4 Example of aliasing.

Pulse-wave Doppler spectrum through bioprosthetic aortic valve. Because the high-velocity blood through the bioprosthetic aortic valve results in a Doppler shift, which is greater than the Nyquist limit, the high-velocity flow away from the transducer is represented as flow toward the transducer. This effect is call aliasing.

where V_m = the maximal velocity that can be unambiguously measured; c = the speed of sound in tissue; R = the range or distance from the transducer at which the measurement is to be made; and f₀ = the frequency of emitted ultrasound.

Based on Equation 11, this “aliasing” artifact can be avoided by either minimizing R or f₀. Decreasing the depth of the sample volume in essence increases f_s. This higher sampling frequency allows for the more accurate determination of higher Doppler shifts frequencies (i.e., higher velocities). Furthermore, because f_o is directly related to f_d (see Eq. 7), a lower emitted ultrasound frequency will produce a lower Doppler frequency shift for a given velocity (see Figure 12-3). This lower Doppler frequency shift will allow for a higher velocity measurement before aliasing occurs.

Continuous-Wave Doppler

The CW Doppler technique uses continuous, rather than discrete, pulses of ultrasound waves. Ultrasound waves are continuously being both transmitted and received by separate transducers. As a result, the region in which flow dynamics are measured cannot be precisely localized. Because of the large range of depths being simultaneously insonated, a large range of frequencies is returned to the transducer. This large frequency range corresponds to a large range of blood-flow velocities. This large velocity range is known as “spectral broadening.” Spectral broadening during CW Doppler interrogation contrasts the homogenous envelope that is obtained with PW Doppler (Figure 12-5). Blood-flow velocity is, however, measured with great accuracy even at high flows because sampling frequency is very high. CW Doppler is particularly useful for the evaluation of patients with valvular lesions or congenital heart disease (CHD), in whom anticipated high-pressure/high-velocity signals are anticipated. It also is the preferred technique when attempting to derive hemodynamic information from Doppler signals (Box 12-2).

Figure 12-5 Pulse-wave (PW) versus continuous-wave (CW) Doppler.

Both images are Doppler spectra through the mitral valve. Left, PW Doppler is used. Because a specific region of interest is defined by the Doppler gate, a clean envelope of transmitral flow is displayed. Right, CW Doppler is used. Because spatial specificity is lost, spectral broadening of velocities is displayed.

BOX 12-2 Pulsed-Wave Versus Continuous-Wave Doppler Analysis

• Pulsed-wave Doppler

• Spatial specificity

• Ambiguity in the measurement of high velocities

• Continuous-wave Doppler

• Able to measure high velocities accurately

• Spatial ambiguity

Three-Dimensional Reconstruction

Echocardiography has become a vital tool in the practice of contemporary cardiac anesthesiology. As with any technology, a considerable evolution has occurred since it was first introduced into the operating rooms in the early 1980s. Among the most important advances has been the progression from one-dimensional (1D; e.g., M-mode) imaging to 2D imaging, as well as spectral Doppler and real-time color-flow mapping superimposed over a 2D image. The heart, however, remains a 3D organ. Although multiplane 2D images can be acquired easily with modern TEE probes by simply rotating the image plane electronically from 0 to 180 degrees, the final process occurs by the echocardiographer stitching the different 2D planes together and creating a “mental” 3D image. Transmitting this “mental” image to other members of the surgical team can sometimes be quite challenging. By directly displaying a 3D image onto the monitor, cardiac anatomy and function could be assessed more rapidly and communication between the echocardiographer and the cardiac surgeon facilitated before, during, and immediately after surgery.⁹

Historic Overview

Early concepts of 3D echocardiography (3DE) found their roots in the 1970s.¹⁰ Because of the limitations of hardware and software capabilities in that era, the acquisition times required to create a 3D image prohibited widespread clinical acceptance, limiting its use for research purposes only. Technologic advances in the 1990s enabled 3D reconstruction from multiple 2D images obtained from different imaging planes. By capturing an image every 2 to 3 degrees as the probe rotated 180 degrees around a specific region of interest (ROI), high-powered computers were able to produce a 3D image, which could be refined further with postprocessing software. These multigated image planes must be acquired under electrocardiographic and respiratory gating to overcome motion artifact. The limitations of this technology are the time required to process and optimize the 3D image and the inability to obtain instantaneous, real-time imaging of the heart.

In 2007, a real-time 3D TEE probe with a matrix array of piezo-electric crystals within the transducer head was released on the market. This 3D imaging matrix array, as opposed to conventional 2D imaging transducers, not only has columns in a single 1D plane but also rows of elements. That is, instead of having a single column of 128 elements, the matrix array comprises more than 50 rows and 50 columns of elements (Figure 12-6). Although this “matrix” technology was available for transthoracic (precordial) scanning, a breakthrough in engineering design was required before the technology could be transitioned into the limited space of the head of a TEE probe.

Figure 12-6 Matrix array transducer consisting of 50 rows and 50 columns of piezoelectric elements.

A human hair demonstrates the size of each individual element.

Display of Three-Dimensional Images

The classic 20 views of 2D TEE are not required in 3DE because entire volumetric datasets are acquired that can be spatially orientated and cropped at the discretion of the echocardiographer. As previously stated, the limiting factor in 3DE is no longer processer performance, but the speed of sound traveling through tissue (1540 m/sec). Although the matrix configuration of the elements allows “live” and instantaneous scanning, the size of this sector is limited to guarantee adequate image resolution and frame rate. If larger sectors are to be scanned, the constraint of transmit time of ultrasound is sidestepped by stitching four to eight gated beats together, which enables wider volumes to be generated while maintaining frame rate and resolution. Several modes of 3DE are described in the following subsections.

Narrow Sector (Live 3D)—Real Time

In this mode, a 3D volume pyramid is obtained. The image shown in this mode is real time. The 3D image changes as the transducer is moved just as in live 2D imaging. Manipulations of the TEE probe (e.g., rotation, change in position) lead to instantaneous changes in the image seen on the monitor (Figure 12-7).

Figure 12-7 Live three-dimensional image of mitral valve and left ventricle (LV).

Because of the matrix structure of the ultrasound transducer, this image represents a true live image. Change in transesophageal echocardiographic probe positioning by the echocardiographer will result in instantaneous changes in the volumetric data set.

Wide Sector Focused (3D Zoom)—Real Time

If only a specific ROI requires imaging, the “zoom mode” can be used in a similar fashion as in 2DE. A typical example for this mode would be the mitral valve (MV) apparatus. The 3D zoom mode displays a small magnified pyramidal volume that may vary from 20 × 20 degrees up to 90 × 90 degrees, depending on the density setting. This small data set can be spatially orientated at the discretion of the echocardiographer. A key advantage to this mode is the fact that the real-time 3D images are devoid of rotational artifacts, as are commonly encountered with electrocardiogram (ECG)-gated 3D acquisitions (Figure 12-8).

Figure 12-8 Three-dimensional (3D) zoom mode acquisition of a mitral valve repair from the left atrial perspective.

Annuloplasty ring is easily visualized. In analogy to the live 3D mode, the 3D zoom mode acquires instantaneous images.

Large Sector (Full Volume)—Gated

Because of insufficient time for sound to travel back and forth in large volumes while maintaining a frame rate greater than 20 Hz and reasonable resolution in live scanning modes, one maneuver to overcome this limitation entails stitching four to eight gates together to create a “full-volume” mode. These gated “slabs” or “subvolumes” represent a pyramidal 3D dataset as would be acquired in the live 3D mode. This technique can generate more than 90-degree scanning volumes at frame rates greater than 30 Hz. Increasing the gates from four to eight creates smaller 3D slabs; this can be used to maintain frame rates and/or resolution as the volumes (pyramids) become larger (Figure 12-9).

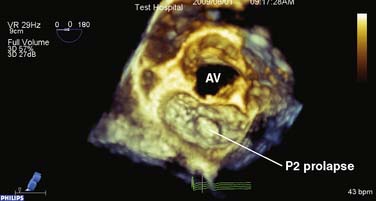
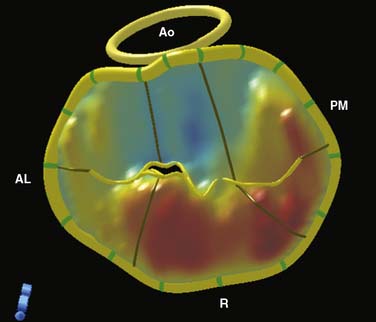
Figure 12-9 Full-volume mode acquisition of the mitral valve from the left atrial perspective.

Although the sector size is similar to Figure 12-8, note the improvement in temporal resolution as a consequence of the four-beat acquisition (9 vs. 29 Hz). This mode does not permit instantaneous “live” imaging. AV, aortic valve.

Unfortunately, as with any conventional gating technique, patients with arrhythmias are prone to motion artifacts when the individual datasets are combined; however, as long as the RR intervals fall within a reasonable range, a full-volume dataset still can be reconstructed (e.g., atrial fibrillation, electrocautery artifact). The acquired real-time 3D dataset subsequently can be cropped, analyzed, and quantified using integrated software in the 3D operating system (QLAB; Philips Healthcare, Andover, MA; Figure 12-10).

Figure 12-10 Mitral valve quantification (MVQ) of a mitral valve acquired with the full-volume mode. Red coloring of the leaflet indicates areas that exceed the annular plane (type II dysfunction). AL, anterolateral; Ao, aorta; PM, posteromedial.

Three-dimensional Color Doppler—Gated

Because of the large amount of data that must be acquired with 3D color Doppler mode, a gating method must be utilized similar to that of the full-volume mode; however, because of the large amount of data required, 8 to 11 beats need to be combined to create an image. Jet direction, extent, and geometry easily can be recognized using this technique. Reports started emerging in the late 1990s showing that the strength of this methodology lies in its ability to quantitate severity of regurgitant lesions; 3D quantification of mitral regurgitation correlates better than 2D imaging, when using angiography as the gold standard.¹¹ In an experimental setting, 3D quantification was more accurate (2.6% underestimation) than 2D or M-mode methods, which had the tendency to underestimate regurgitant volumes (44.2% and 32.1%, respectively; Figure 12-11).¹²

Figure 12-11 Color three-dimensional acquisition of a mitral valve (MV) bioprosthesis.

The aortic valve (AV) is for orientation. Three paravalvular leaks can be identified.

Contrast Echocardiography

Normally, red blood cells scatter ultrasound waves weakly, resulting in their black appearance on ultrasonic examination. Contrast echocardiography is performed by injecting nontoxic solutions containing gaseous microbubbles. These microbubbles present additional gas-liquid interfaces, which substantially increase the strength of the returning signal. This augmentation in signal strength may be used to better define endocardial borders, optimize Doppler envelope signals, and estimate myocardial perfusion.

Gramiak and Shah¹⁵ originally reported the use of contrast echocardiography in 1969. They described visualization of aortic valve (AV) incompetence during left-heart catheterization¹⁵ (Box 12-3). Subsequently, contrast echocardiography has been used to image intracardiac shunts,¹⁶ valvular incompetence,¹⁷ and pericardial effusions.¹⁸ In addition, LV injections of hand-agitated microbubble solutions have been used to identify semiquantitative LV endocardial edges,¹⁹ cardiac output,²⁰ and valvular regurgitation.²¹

Contrast agents are microbubbles, consisting of a shell surrounding a gas. Initial contrast agents were agitated free air in either a saline or blood-saline solution. These microbubbles were large and unstable, so they were unable to cross the pulmonary circulation; they were effective only for right-heart contrast. Because of their thin shell, the gas quickly leaked into the blood with resultant dissolution of the microbubble. Agents with a longer persistence subsequently were developed.

More modern contrast agents have improved the shell surrounding the microbubble, as well as modification of the gas. The shell must inhibit the diffusion of gas into the blood and must enhance the pressure that a microbubble can tolerate before dissolving.²² Gases with low shell diffusivity and blood saturation concentration result in a microbubble of increased survival because the gas would rapid equilibrate with blood, and the gas would tend to stay within the shell. Improvements in the shell both increase the tolerance of the microbubble to ultrasound energies and decrease the diffusion of the gas into the blood; both changes further increase the persistence of the microbubbles. At the same time, there must be an element of fragility; the microbubbles must be disrupted by ultrasound signals producing appropriate imaging effects. The use of high-molecular-weight and less-soluble gases further increases the persistence of the contrast agents. Currently, the perfluorocarbons are the most common gases used in contrast agents. The microbubbles need to be small enough to transverse the pulmonary circulation with a predominant size particle that approached the size of an erythrocyte. The number of larger particles needs to be minimized to reduce the risk for obstruction of pulmonary capillary flow. Because the reflected energy of contrast agents is high, attenuation of the ultrasound signal is common. This signal attenuation interferes with visualization of distal structures.

An ultrasound signal produces compression and rarefaction (expansion) of the medium through which it travels. When this compression and rarefaction impact a microbubble, the bubble is compressed and expanded, respectively.²³ These changes result in changes in the bubble volume, causing bubble vibrations with subsequent effects on the returning ultrasound signal. These bubble pulsations may result in changes in the bubble radius by a factor of 20 or more.²⁴

The acoustic properties of these microbubbles depend on the amplitude of the ultrasound signal. The amplitude of an ultrasound signal usually is defined by its mechanical index, which is the peak negative pressure divided by the square root of the ultrasound frequency. Normally, when bubbles are insonated by ultrasound at their intrinsic resonant frequency, they vibrate; during the peak of the signal, they are compressed, and at the nadir of the signal, they expand. An ideal bubble would oscillate at the insonated ultrasound frequency.²⁵ At low ultrasound amplitudes (mechanical index < 0.1), the microbubbles oscillate at the frequency of the insonated signal with the degree of compression being equal to the degree of expansion. This is called linear oscillation. With fundamental imaging, no special contrast echo signals are produced.²⁶ With increasing signal amplitudes (mechanical index, 0.1 to 0.7), the degree of expansion exceeds the degree of compression, which results in nonlinear oscillations. These nonlinear oscillations result in the creation of ultrasound waves at harmonic frequencies of the delivered ultrasound waves. Although some bubble destruction will occur at all amplitudes, further increases in ultrasound amplitude (mechanical index, 0.8 to 1.9) result in more compression and expansion with subsequent extensive bubble destruction. This bubble destruction, called scintillation, results in a brief but high output signal appearing as swirling. Because of the extensive bubble destruction, intermittent imaging must be performed to allow contrast replenishment. The role of most contrast imaging modalities is to create and display these nonlinear components while suppressing the linear echoes from tissue and tissue motion.²⁷

Further improvements in image acquisition can be achieved using harmonic imaging.²⁴ As explained previously, nonlinear oscillations result in the creation of harmonics. It was theorized that if the receiver was tuned to receive the first harmonic of the transmitted ultrasound signal, the signal-to-noise ratio can be improved by predominantly imaging signals from the microbubbles producing these harmonics. Because tissues also produce harmonics, tissue grayscale imaging also was enhanced. Further improvements may include subharmonic and ultraharmonic imaging, which may provide more specific contrast enhancement. Harmonic imaging with TEE improves endocardial visualization and allows partial assessment of myocardial perfusion.²⁸ Harmonic-power Doppler is more sensitive for detecting basilar perfusion in the far field compared with harmonic grayscale imaging.²⁹

The first-generation agents were Albunex and Levovist. Currently, Optison (Mallinckrodt, St. Louis, MO) and Definity (DuPont Pharmaceuticals, Waltham, MA) are available in the United States for use; Levovist and Sonovue (Bracco Diagnostics, Princeton, NJ) are approved in Europe. Albunex is no longer available. Albunex utilized albumin encapsulation to stabilize a 4-μm air bubble that could opacify the left ventricle but did not result in good microvascular perfusion. Levovist uses an air microbubble within a fatty acid shell.

Optison is a refinement of Albunex, with the substitution of perfluoropropane within an albumin shell. Definity uses perfluoropropane within a liposome shell. SonoVue consists of hexafluoride with a phospholipid shell. New agents under development may use polymer shells whose flexibility and size can be controlled more precisely. These agents may be targeted to specific organs or vectors.

The safety of contrast echocardiography must be considered. The contrast agents themselves must have a high therapeutic index. Multiple large bubbles may obstruct pulmonary microcirculation. The disruption of microbubbles by high-amplitude ultrasound may rupture capillaries and injure surrounding tissue.³⁰ Rare allergic and life-threatening anaphylactic/anaphylactoid reactions occur at a rate of approximately 1 per 10,000.²⁷ Premature ventricular contractions have been described during high-intensity triggered imaging.³¹ Other investigators were not able to demonstrate an increase in premature ventricular complex occurrence during or after imaging with triggered ultrasound at a mechanical index of 1.³² Contraindications to the use of perflutren-containing agents include pulmonary hypertension, serious ventricular arrhythmias, severe pulmonary disease, cardiac shunting, or hypersensitivity to perflutren, blood, blood products, or albumin. If current recommendations are followed, contrast echocardiography rarely results in significant side effects.²²

Uses

Diagnostic applications for contrast echocardiography include enhancement of endocardial borders from qualitative assessment of wall motion abnormalities, measurement of LV function, assessment of CHD, quantification of valvular regurgitation, enhancement of CFD signals, and assessment of myocardial perfusion. During cardiac surgery, the special and unique applications of myocardial contrast echocardiography include measurements of perfusion area after coronary artery bypass graft (CABG) surgery, assessment of quality of coronary bypass grafts and cardioplegia distribution, and correct assessment of the results of surgery for ventricular septal defect. Noncardiac intraoperative applications include assessment of perfusion in the kidney and in skeletal muscle. Work is ongoing to investigate the potential for analyzing cerebral blood flow with contrast-echo techniques.

Enhancement of Right-Sided Structures

Hand-agitated saline solutions are still useful to enhance right-sided structures. These saline solutions can be prepared easily by hand agitation of saline between two 10-mL Luer lock syringes connected by a three-way stopcock; small amounts of blood or air may be added to improve right-sided opacification. This technique is used most commonly to opacify the right atrium (RA) and right ventricle, assisting in the diagnosis of intra-atrial and ventricular shunts, and to enhance pulmonary arterial Doppler signals. The most common indication is the detection of a patent foramen ovale (PFO). After obtaining a bicaval view, a Valsalva maneuver is induced and hand-agitated saline is injected into a large vein. After the RA is opacified, the Valsalva is released and the left atrium (LA) is examined for contrast.

Left Ventricular Opacification

The commercially available contrast agents allow for left ventricular opacification (LVO) as well. Relatively low mechanical index modes usually are used (<0.2), to allow for bubble detection without bubble destruction. The images are processed such that the linear scatters from tissue are completely eliminated, leaving only nonlinear scatters from the bubble contrast. The LVO allows enhancement of LV endocardial borders in patients in whom normal studies are challenging.^33,34 Such challenging studies include patients who are obese, with pulmonary disease, are critically ill, or on a ventilator.²⁷ The use of LVO substantially increases the accuracy of LV volume determination compared with electron beam computed tomography measurements, decreases interobserver variability associated with these measurements, and increases the number of myocardial segments that may be described accurately during stress echocardiography.^35,36 Underestimation of LV volume measurements, which is common with standard echocardiography, may be virtually eliminated with the use of LVO.³⁷ Finally, LVO provides greater visualization of structural abnormalities such as apical hypertrophy, noncompaction, ventricular thrombus, endomyocardial fibrosis, LV apical ballooning (Takotsubo), LV aneurysms or pseudoaneurysms, and myocardial rupture.²⁷

Aortic Dissections

Echocardiographic contrast may be used to diagnose aortic dissections. Artifacts may be distinguished from true aortic dissection and artifact, by the homogenous distribution of contrast within the aortic lumen.²⁷ The intimal flap may be visualized, the entry and exit point may be identified, and the extension into major aortic branches may be more easily defined. The use of contrast further increases the successful differentiation between the true and false lumen.

Doppler Enhancement

The administration of contrast will enhance the echocardiographic Doppler spectrum, where the signal is weak or suboptimal.³⁸ The enhancement is particularly useful in the evaluation of aortic stenosis (AS) but also may be used with transmitral evaluation, pulmonary venous flow determination, or regurgitant tricuspid valvular flow (Figure 12-12). Whereas the threshold for detecting contrast is substantially less for Doppler compared with 2D imaging, contrast agents usually are used initially for the latter application.

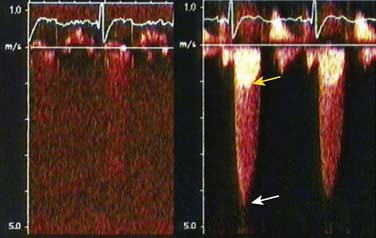
Figure 12-12 Doppler enhancement of aortic stenosis.

Doppler spectrum through the left ventricular outflow tract in a patient with aortic stenosis is shown. The image on the left is without contrast, and the image on the right is after contrast enhancement. The contrast-enhanced image clearly demonstrates the high-velocity envelope consistent with aortic stenosis, which could not be visualized without contrast.

(Reproduced from Mulvagh SL, Rakowski H, Vannan MA, et al: American Society of Echocardiography Consensus Statement on the Clinical Applications of Ultrasonic Contrast Agents in Echocardiography. J Am Soc Echocardiogr 21:1179–1201, 2008, by permission.)

Myocardial Perfusion

The second-generation agents allow for perfusion of the myocardial microcirculation. This perfusion allows for assessment of perfusion patterns, coronary artery stenosis, and myocardium at risk during acute coronary syndromes.²⁶ Currently, only Imagify has U.S. Food and Drug Administration approval for myocardial perfusion imaging.

Lindner et al³⁹ described a method for the quantification of myocardial blood flow using contrast echocardiography. If a contrast agent is administered at a steady rate, the blood concentration and myocardial concentration of the contrast agent will equilibrate. If a single high-amplitude (i.e., high mechanical index) ultrasound pulse is delivered to a myocardial ROI, the microbubbles will be destroyed; they will be replenished as the contrast-filled blood perfuses the myocardium. The rate of contrast replenishment in the myocardium is directly related to myocardial blood flow. Repeated ultrasound pulses are delivered at shorter frequencies until a maximum myocardial contrast-enhanced ultrasound signal is obtained. A time-myocardial contrast intensity curve is constructed. Myocardial-contrast echocardiographic-derived indications of myocardial perfusion rate have relatively good between-study and between-reading reproducibility.⁴⁰

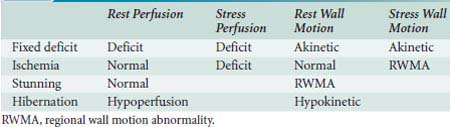
If contrast echocardiography is used in conjunction with traditional echocardiography, different flow patterns can be described as outlined in Table 12-3. A fixed myocardial deficit may be diagnosed with a perfusion deficit during rest and stress and with akinetic segments during both of these periods. An ischemic segment may be defined as a segment with normal perfusion and wall motion with rest, and a perfusion deficit during stress that is accompanied by a regional wall motion abnormality (RWMA). Myocardial stunning may be diagnosed if normal perfusion is observed during rest in the presence of a hypokinetic rest wall motion, and hibernation may be diagnosed with rest hypoperfusion and with hypokinetic rest wall motion. The addition of myocardial-contrast echocardiography (MCE) may increase the sensitivity, but not specificity, of dipyridamole-exercise echocardiography. Moir et al⁴¹ combined MCE with dipyridamole-exercise echocardiography in 85 patients. They detected significant coronary artery stenosis in 43 patients involving 69 coronary areas. The addition of MCE improved sensitivity for the detection of CAD (91% vs. 74%; P = 0.02) and accurate recognition of disease extent (87% vs. 65% of territories; P = 0.003).

TABLE 12-3 Diagnosis of Fixed Myocardial Deficits, Ischemia, Stunning, and Hibernation Based on Perfusion and Wall Motion Findings

Measurements of myocardial blood flow by MCE are comparable with other techniques. Senior et al⁴² compared MCE and single-photon emission computerized tomography (SPECT) for the detection of coronary artery disease (CAD) in 55 patients with a medium probability of CAD. The sensitivity of MCE was significantly greater than that of SPECT for the detection of CAD (86% vs 43%; P < 0.0001); however, the specificities were not significantly different (88% and 93%; P = 0.52). In another investigation, quantitative real-time MCE with dipyridamole defined the presence and severity of CAD in a manner that compared favorably with quantitative SPECT.⁴³

Microvascular perfusion is a prerequisite for ensuring viability early after acute myocardial infarction (AMI). For adequate assessment of myocardial perfusion, both myocardial blood volume and velocity need to be evaluated. Because of its high frame rate, low-power continuous MCE can assess both myocardial blood volume and velocity, allowing for assessment of microvascular perfusion.⁴⁴ To differentiate necrotic from viable myocardium after reperfusion therapy, Janardhanan et al⁴⁴ examined 50 patients with low-power continuous MCE 7 to 10 days after acute myocardial perfusion. Myocardial perfusion by contrast opacification was assessed over 15 cardiac cycles after the destruction of microbubbles, and wall thickening was assessed at baseline. Regional and global LV function were reassessed after 12 weeks. Of the segments without contrast enhancements, 93% showed no recovery of function; in the segments with contrast opacification, 84% exhibited functional recovery. The greater the extent and intensity of contrast opacification, the better the LV function at 3 months (P < 0.001; r = −0.91). Almost all patients (94%) with less than 20% perfusion in dysfunctional myocardium (assessing various cutoffs) failed to demonstrate an improvement in LV function.

Janardhanan et al⁴⁵ performed MCE in 70 patients with AMI after thrombolysis. Myocardial perfusion was examined in the akinetic areas in 20 patients with an occluded infarct-related artery that was subsequently revascularized. Contractile reserve was evaluated in these segments 12 weeks after revascularization with dobutamine–echocardiography. Of the 102 akinetic segments, 37 (36%) showed contractile reserve. Contractile reserve was present in 24 of the 29 segments (83%) with homogenous contrast opacification and absent in 60 of the 73 segments (82%) with reduced opacification. Quantitative measurements of myocardial blood flow were significantly greater (P < 0.0001) in the segments with contractile reserve than in those without contractile reserve. MCE may, thus, be used as a reliable bedside technique for the accurate evaluation of collateral blood flow in the presence of an occluded infarct-related artery after AMI. MCE performed early after percutaneous coronary interventions provides information on the extent of infarction, and hence the likelihood for recovery of contractile reserve. The presence of perfusion before this intervention predicts the maintenance of perfusion and recovery of systolic function.⁴⁶

MCE may be a useful tool in the detection of myocardial viability before coronary revascularization. In Korosoglou et al’s⁴⁷ study, contrast echocardiography was compared with low-dose dobutamine stress echocardiography and with combined technetium-99 sestamibi SPECT and fluorodeoxyglucose-18 positron emission tomography. Myocardial recovery was predicted by contrast echocardiography with a sensitivity of 86% and a specificity of 43%, by nuclear imaging with a sensitivity of 90% and specificity of 44%, whereas DSE was similarly sensitive (83%) but more specific (76%). A combination of quantitative MCE and dobutamine stress echocardiography provided the best diagnostic characteristics, with a sensitivity of 96%, a specificity of 63%, and an accuracy of 83%. Fukuda et al⁴⁸ performed myocardial contrast echocardiography on 28 patients with chronic stable CAD and LV dysfunction before and after coronary revascularization. Of the 101 revascularized dysfunctional segments, MCE was adequately visualized in 91 (90%) segments, and wall motion was recovered in 45 (49%) segments. Quantitative measurements of myocardial blood flow in the recovery segments were significantly greater than that in nonrecovery segments. The investigators concluded that quantitative intravenous MCE can predict functional recovery after coronary revascularization.

Basic Principles

The conversion of reflected ultrasound echoes into 2D video images is a complicated process involving numerous electronic and digital manipulations. A 2D echo image is generated by scanning the heart every 17 msec, or 60 times each second. The image generated from a single 1/60th–second scan is called a field. A process called interlacing combines two scans or fields into a frame of 1/30th of a second. Because the eye cannot capture an image lasting 1/30th of a second, microprocessors further process the frame electronically in real time. The intrinsic persistence of the television screen enhances image quality, and the end result is a fairly smooth picture.

Resolution

An ultrasound image may be described by its axial, lateral, and elevational resolution (Box 12-4). Axial resolution is the minimum separation between two interfaces located in a direction parallel to the beam so that they can be imaged as two different interfaces. The most precise image resolution is along this axial plane. The higher the frequency of the ultrasound signal, the greater the axial resolution, because ultrasound waves of shorter wavelengths may be utilized. Shorter bursts of ultrasound waves (i.e., short pulse length) provide greater axial resolution. Pulse length should be no more than two or three cycles. The range of frequencies contained within a given ultrasound transmission is referred to as the “frequency bandwidth.” Generally, the shorter the pulse of the ultrasound produced, the greater the frequency bandwidth. Because of the relation between short pulse lengths and high bandwidths, high bandwidths are associated with better axial resolution. High transducer bandwidths also allow for better resolution of deeper structures.

Lateral resolution is the minimum separation of two interfaces aligned along a direction perpendicular to the ultrasound beam. The most important determinant of lateral resolution is the ultrasound beam width or ultrasound beam focusing; the narrower the beam, the better the lateral resolution. If a small object appears within the “near field,” it can be resolved laterally accurately; however, if it appears within the “far field” the size of this small object will appear to increase with the increase in the width of the ultrasound beam. This increase in size associated with object resolution in the far field results in blurring of deeper structures. Elevational resolution refers to the ability to determine differences in the thickness of the imaging plane. The thickness of the ultrasound beam is a major determinant of elevational resolution.

Preprocessing

Ultrasound echoes are received and converted to electronic signals by the transducer. On most modern echo scanners, the analog electronic signals undergo several modifications before being digitized and eventually displayed as an image. Preprocessing describes the modifications performed on the analog and digital signal before storage.

Dynamic Range Manipulation

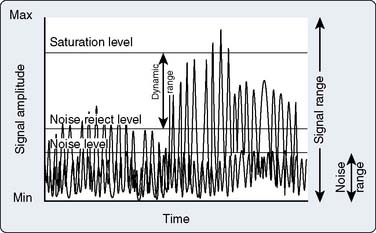
The intensity of echo signals spans a wide range from very weak to very strong. Very strong signals falling beyond the saturation level of the electronic circuitry and very weak signals below the sensitivity of the instrument are automatically eliminated. The dynamic range of the instrument is defined by the limits at which extremely strong or weak signals are eliminated; dynamic range is under operator control (Figure 12-13). In this manner, signals of low intensity that contain little useful information, and mostly noise, can be selectively rejected.

Figure 12-13 Dynamic range of a representative echocardiographic display system.

All ultrasound signals begin at zero signal level and can increase in amplitude until they reach the signal “saturation” level. Many of the low-intensity signals fall within the range of the background noise and are, therefore, obscured. All systems have a built-in system reject, which eliminates both the system noise and the low-intensity echoes that lie just above the noise level. The dynamic range of the system is between the noise reject level and the saturation level. Signals within the dynamic range appear on the image display.

(From Thys DM, Hillel Z: How it works: Basic concepts in echocardiography. In Bruijn NP, Clements F [eds]: Intraoperative use of echocardiography. Philadelphia: JB Lippincott, 1991.)

A wide dynamic range is necessary for high resolution, whereas a narrow range facilitates the discrimination between true image signals and noise. In clinical echocardiography, strong signals that arise from dense tissues (e.g., cardiac valves) and weaker signals arising from soft tissues (e.g., myocardium) are of interest. To give the weaker signals a greater representation in the dynamic range, an amplifier converts the linear signal intensity scale into a logarithmic scale. Although this increases the number of weaker signals detected, it also, unfortunately, tends to amplify noise.

Lateral Gain Control

A recent innovation allows the application of gain control to selected sectors of the ultrasound image. This feature appears particularly useful for the enhancement of image strength of structures that are nearly parallel to the ultrasound beam (e.g., the septum and lateral wall on a short–axis (SAX) view at the level of the papillary muscles; Figure 12-14).

Figure 12-14 A, Standard two-dimensional short-axis view of the left ventricle at the level of the papillary muscles. Note that the image drops out in the septal and lateral walls (vertical portions of the wall). B, Identical view after application of lateral gain adjustments. The septal and lateral walls appear brighter and less dropout is seen.

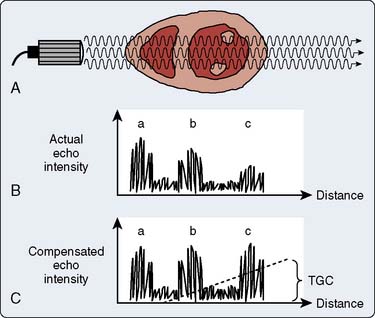
Time-Gain Compensation

Because any wave traveling through tissues is attenuated proportionally to the traveled distance, it is necessary to compensate for the fact that echoes returning from more distant objects will be weaker than those from equally dense objects closer to the transducer. A mechanism called depth compensation or time-gain compensation (TGC) is used to achieve this. The manner in which time-gain compensation is obtained is illustrated in Figure 12-15. Time-gain compensation can be manually or automatically controlled.

Figure 12-15 A, As an ultrasound beam is aimed across the heart, specular echoes are reflected at the right ventricular wall (a), the septum (b), and the left ventricular wall (c). B, The normal loss of echo strength is due to the decreasing intensity of the beam as it propagates through the heart. C, Time-gain compensation (TGC) allows the intensity of the far-field signals to be increased selectively.

(From Thys DM, Hillel Z: How it works: Basic concepts in echocardiography. In Bruijn NP, Clements F [eds]: Intraoperative use of echocardiography. Philadelphia: JB Lippincott, 1991.)

Leading-Edge Enhancement

Leading-edge enhancement, or differentiation, is another type of preprocessing used to sharpen the ultrasound image. The reflected echo signal undergoes half–wave rectification and is smoothed into a signal envelope (Figure 12-16A, B). An amplifier then differentiates the leading edge of the smoothed signal envelope from its first mathematical derivative (see Figure 12-16C), and a narrower and brighter image spot is formed (see Figure 12-16D). Because a 2D image is composed of multiple radially juxtaposed scan lines, excessive edge enhancement narrows bright spots in the direction of travel of the echo beam (i.e., axially but not laterally). For this reason, leading-edge enhancement primarily is performed on M-mode scans, whereas instruments with 2D mode capability use little or no edge enhancement in the 2D mode. Therefore, M-mode images often have better resolution than 2D images and are better suited for quantitative measurements.

Figure 12-16 Leading-edge enhancement techniques.

A, Radiofrequency (RF) type of echo display. B, This video represents the average height of the upper half of the RF signal. C, Differentiation is obtained by taking the first derivative of the video display. D and E, Intensity modulation represents the conversion of signal amplitude to intensity, changing the signal from a spike to a dot.

(From Thys DM, Hillel Z: How it works: Basic concepts in echocardiography. In Bruijn NP, Clements F [eds]: Intraoperative use of echocardiography. Philadelphia: JB Lippincott, 1991.)

Postprocessing

Digital Scan Conversion

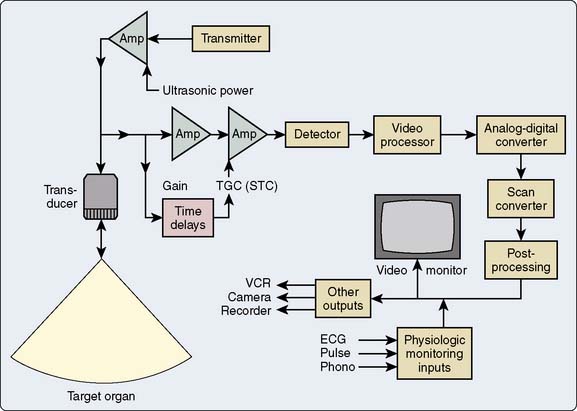
After completing analog preprocessing, ultrasound devices digitize the image data with an analog-to-digital (A–D) converter (Figure 12-17). Further processing is done while data are stored in the digital memory (input processing) or as they are received from the memory (output processing). An early step in digital processing uses a scan converter to transform the information obtained as radial sector scan lines into a rectangular (Cartesian) format for television screen display.

Figure 12-17 Schematic of a modern ultrasound scanner.

Arrows indicate the directions for the flow of information or electronic power. Amp, electronic amplifier; ECG, electrocardiogram; TGC (STC), time-gain compensation; VCR, videocassette recorder.

(From Thys DM, Hillel Z: How it works: Basic concepts in echocardiography. In Bruijn NP, Clements F [eds]: Intraoperative use of echocardiography. Philadelphia: JB Lippincott, 1991.)

The memory stores the information of two adjacent scan fields consisting of a total of 128 scan lines. Each scan line is assigned to one column of memory. There is also one row of memory for each of the 512 horizontal television image lines (raster lines). Therefore, a typical television display of an echo image consists of 128 columns by 512 rows for a total of 65,536 picture elements, or pixels. Although the monitor displays only 64 shades of gray for each pixel, the memory unit assigned to each pixel has the capacity to store 1024 degrees of brightness. Each pixel is assigned 10 binary bits of memory for a total of 2¹⁰ (1024) possible storage combinations.

Image Storage

All modern echo scanners allow the operator to store or “freeze” a single echo image on the display screen. This allows the scrutiny of any unusual transient anatomic or physiologic observations. Once frozen, an image also can be subjected to some simple quantitative measurements. With the continuous motion of the cardiac structures, it is often difficult, however, to capture the exact frame that is to be analyzed. For this reason, techniques to acquire several consecutive frames have been developed.

Safety Guidelines and Contraindications

To ensure the continued safety of TEE, the following recommendations have been made: The probe should be inspected before each insertion for cleanliness and structural integrity. If possible, the electrical isolation also should be checked. The probe should be inserted gently, and if resistance is met, the procedure aborted. Minimal transducer energy should be used and the image frozen when not in use. Finally, when not imaging, the probe should be left in the neutral, unlocked position to avoid prolonged pressure on the esophageal mucosa.

Absolute contraindications to TEE in intubated patients include esophageal stricture, diverticula, tumor, recent suture lines, and known esophageal interruption. Relative contraindications include symptomatic hiatal hernia, esophagitis, coagulopathy, esophageal varices, and unexplained upper gastrointestinal bleeding. Notably, despite these relative contraindications, TEE has been used in patients undergoing hepatic transplantation without reported sequelae.^78,79