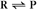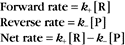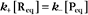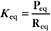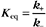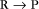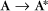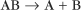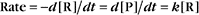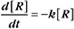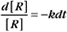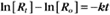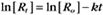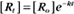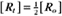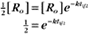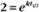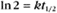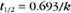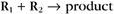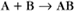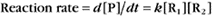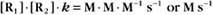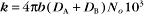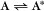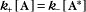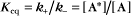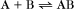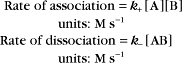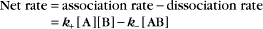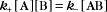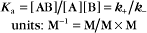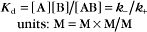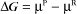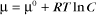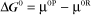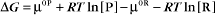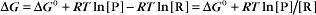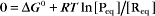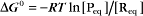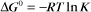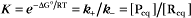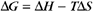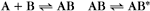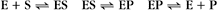First-Order Reactions

First-order reactions have one reactant (R) and produce a product (P). The general case is simply

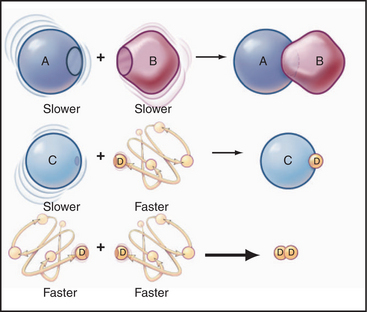
Some common examples of first-order reactions (Fig. 4-1) include conformational changes, such as a change in shape of protein A to shape A^*:

Figure 4-1 first-order reactions. In first-order reactions, a single reactant undergoes a change. In these examples, molecule A changes conformation to ^* and the bimolecular complex AB dissociates to A and B. The rate constant for a first-order reaction (arrows) is a simple probability.

and the dissociation of complexes, such as

The rate of a first-order reaction is directly proportional to the concentration of the reactant (R, A, or AB in these examples). The rate of a first-order reaction, expressed as a differential equation (rate of change of reactant or product as a function of time [t]), is simply the concentration of the reactant times a constant, the rate constant k, with units of s⁻¹ (pronounced “per second”):

The rate of the reaction has units of M s⁻¹, where M is moles per liter and s is seconds (pronounced “molar per second”). As the reactant is depleted, the rate slows proportionally.

A first-order rate constant can be viewed as a probability per unit of time. For a conformational change, it is the probability that any A will change to ^* in a unit of time. For dissociation of complex AB, the first-order rate constant is determined by the strength of the bonds holding the complex together. This “dissociation rate constant” can be viewed as the probability that the complex will fall apart in a unit of time. The probability of the conformational change of any particular A to ^* or of the dissociation of any particular AB is independent of its concentration. The concentra-tions of A and AB are important only in determining the rate of the reaction observed in a bulk sample (Box 4-2).

To review, the rate of a first-order reaction is simply the product of a constant that is characteristic of the reaction and the concentration of the single reactant. The constant can be calculated from the half-time of a reaction (Box 4-2).

Second-Order Reactions

Second-order reactions have two reactants (Fig. 4-2). The general case is

Figure 4-2 second-order reactions. In second-order reactions, two molecules must collide with each other. The rate of these collisions is determined by their concentrations and by a collision rate constant (arrows). The collision rate constant depends on the sum of the diffusion coefficients of the reactants and the size of their interaction sites. The rate of diffusion in a given medium depends on the size and shape of the molecule. Large molecules, such as proteins, move more slowly than small molecules, such as adenosine triphosphate (ATP). A protein with a diffusion coefficient of 10⁻¹¹ m² s⁻¹ diffuses about 10 mm in a second in water, while a small molecule such as ATP diffuses 100 times faster. The rate constants (arrows) are about the same for A + B and C + D because the large diffusion coefficient of D offsets the small size of its interaction site on C. Despite the small interaction size, D + D is faster because both reactants diffuse rapidly.

A common example in biology is a bimolecular association reaction, such as

where A and B are two molecules that bind together. Some examples are binding of substrates to enzymes, binding of ligands to receptors, and binding of proteins to other proteins or nucleic acids.

The rate of a second-order reaction is the product of the concentrations of the two reactants, R₁ and R₂, and the second-order rate constant, k:

The second-order rate constant, k, has units of M⁻¹ s⁻¹ (pronounced “per molar per second”). The units for the reaction rate are

the same as a first-order reaction.

The value of a second-order “association” rate constant, k₊, is determined mainly by the rate at which the molecules collide. This collision rate depends on the rate of diffusion of the molecules (Fig. 4-2), which is determined by the size and shape of the molecule, the viscosity of the medium, and the temperature. These factors are summarized in a parameter called the diffusion coefficient, D, with units of m² s⁻¹. D is a measure of how fast a molecule moves in a given medium. The rate constant for collisions is described by the Debye-Smoluchowski equation, a relationship that depends only on the diffusion coefficients and the area of interaction between the molecules:

where b is the interaction radius of the two particles (in meters), the Ds are the diffusion coefficients of the reactants, and N _o is Avogadro’s number. The factor of 10³ converts the value into units of M⁻¹ s⁻¹.

For particles the size of proteins, D is approximately 10⁻¹¹ m² s⁻¹ and b is approximately 2 × 10⁻⁹ μ, so the rate constants for collisions of two proteins are in the range of 3 × 10⁸ M⁻¹ s⁻¹. For small molecules such as sugars, D is approximately 10⁻⁹ m² s⁻¹ and b is approximately 10⁻⁹ μ, so the rate constants for collisions of a protein and a small molecule are about 20 times larger than collisions of two proteins, in the range of 7 × 10⁹ M⁻¹ s⁻¹. On the other hand, experimentally observed rate constants for the association of proteins are 20 to 1000 times smaller than the collision rate constant, on the order of 10⁶ to 10⁷ M⁻¹ s⁻¹. The difference is attributed to a steric factor that accounts for the fact that macromolecules must be correctly oriented relative to each other to bind together when they collide. Thus, the complementary binding sites are aligned correctly only 0.1% to 5% of the times that the molecules collide.

Many binding reactions between two proteins, between enzymes and substrates, and between proteins and larger molecules (e.g., DNA) are said to be “diffusion limited” in the sense that the rate constant is determined by diffusion-driven collisions between the reactants. Thus, many association rate constants are in the range of 10⁶ to 10⁷ M⁻¹ s⁻¹.

To review, the rate of a second-order reaction is simply the product of a constant that is characteristic of the reaction and the concentrations of the two reactants. In biology, the rates of many bimolecular association reactions are determined by the rates of diffusion-limited collisions between the reactants.

Reversible Reactions

Most reactions are reversible, so the net rate of a reaction is equal to the difference between the forward and reverse reaction rates. The forward and reverse reactions can be any combination of first- or second-order reactions. A reversible conformational change of a protein from A to ^* is an example of a pair of simple first-order reactions:

The forward reaction rate is k₊A with units of M s⁻¹, and the reverse reaction rate is k_–^* with the same units. At equilibrium, when the net concentrations of A and ^* no longer change,

and

This equilibrium constant is unitless, since the units of concentration and the rate constants cancel out.

The same reasoning with respect to the equilibrium constant applies to a simple bimolecular binding reaction:

where A and B are any molecule (e.g., enzyme, receptor, substrate, cofactor, or drug). The forward (binding) reaction is a second-order reaction, whereas the reverse (dissociation) reaction is first-order. The opposing reactions are

The overall rate of the reaction is the forward rate minus the reverse rate:

Depending on the values of the rate constants and the concentrations of A,B, and AB, the reaction can go forward, backward, or nowhere.

At equilibrium, the forward and reverse rates are (by definition) the same:

The equilibrium constant for such a bimolecular reaction can be written in two ways:

This is the classical equilibrium constant used in chemistry, where the strength of the reaction is proportional to the numerical value. For bimolecular reactions, the units of reciprocal molar are difficult to relate to, so biochemists frequently use the reciprocal relationship:

When half of the total A is bound to B, the concentration of free B is simply equal to the dissociation equilibrium constant.

Thermodynamic Considerations

The driving force for chemical reactions is the lowering of the free energy of the system when reactants are converted into products. The larger the reduction in free energy, the more completely reactants will be converted to products at equilibrium. A thorough consideration of thermodynamics is beyond the scope of this text, but an overview of this subject is presented to allow the reader to gain a basic understanding of its power and simplicity.

The change in Gibbs free energy, δG, is simply the difference in the chemical potential, μ, of the reactants (R) and products (P):

The chemical potential of a particular chemical species depends on its intrinsic properties and its concentration, expressed as the equation

where μ⁰ is the chemical potential in the standard state (1 M in biochemistry), R is the gas constant (8.3 J mol⁻¹ degree⁻¹), T is the absolute temperature in degrees Kelvin, and C is the ratio of the concentra-tion of the chemical species to the standard concentration. Because the standard state is defined as 1 μ, the parameter C has the same numerical value as the molar concentration, but is, in fact, unitless. The term RT ln C adjusts for the concentration. When C = 1, μ= μ⁰.

Under standard conditions in which one mole of reactant is converted to one mole of product, the standard free energy change, δG⁰, is

However, because most reactions do not take place under these standard conditions, the chemical potential must be adjusted for the actual concentrations. This can be done by including the concentration term from the definition of the chemical potential. An equation for the free energy change that takes concentrations into account is

Substituting the definition of δG⁰, we have

This relationship tells us that the free energy change for the conversion of reactants to products is simply the free energy change under standard conditions corrected for the actual concentrations of reactant and products.

At equilibrium, the concentrations of reactants and products do not change and the free energy change is zero, so

or

The reader is already familiar with the fact that the equilibrium constant for a reaction is the ratio of the equilibrium concentrations of products and reactants. Thus, that relationship can be substituted in this thermodynamic equation:

or

This profound relationship shows how the free energy change is related to the equilibrium constant. The change in the standard Gibbs free energy, δG⁰, specifies the ratio of products and reactants when the reaction reaches equilibrium, regardless of the rate or path of the reaction. The free energy change provides no information about whether or not a given reaction will proceed on a time scale relevant to cellular activities. Nevertheless, because the equilibrium constant depends on the ratio of the rate constants, knowledge of the rate constants reveals the equilibrium constant and the free energy change for a reaction. Consider the consequences of various values of δG⁰:

It is sometimes said that a reaction with a positive δG⁰ will not proceed spontaneously. This is not strictly true. Reactants will still be converted to products, although relative to the concentration of reactants, the concentration of products will be small. The size and sign of the free energy change tell nothing about the rate of a reaction. For example, the oxidation of sucrose by oxygen is highly favored with a δG⁰ of −5693 kJ/mol, but “a flash fire in a sugar bowl is an event rarely, if ever, seen.”^*

The free energy change is additionally related to two thermodynamic parameters that are important to the subsequent discussion of molecular interactions. The Gibbs-Helmholtz equation is the key relationship:

where δH is the change in enthalpy, an approximation (with a small correction for pressure-volume work) of the bond energies of the molecules. Thus, δH is the heat given off when a bond is made or the heat taken up when a bond is broken. The change in enthalpy is simply the difference in enthalpy of reactants and products. In biochemical reactions, the enthalpy term principally reflects energies of the strong covalent bonds and of the weaker hydrogen and electrostatic bonds. If no covalent bonds change, as in a binding reaction or a conformational change, δH is determined by the difference in the energy of the weak bonds of the products and reactants.

The change in entropy, expressed as δS is a measure of the change in the order of the products and reactants. The value of the entropy is a function of the number of microscopic arrangements of the system, including the solvent molecules. Note the minus sign in front of the TδS term. Reactions are favored if the change in entropy is positive, that is, if the products are less well ordered than the reactants. Increases in entropy drive reactions by increasing the negative free energy change. For example, the hydrophobic effect, which is discussed later in this chapter, depends on an increase in entropy. Increases in entropy provide the free energy change for many biologic reactions, especially macromolecular folding (see Chapters 3 and 17) and assembly (see Chapter 5).

As was emphasized in the case of δG, neither the rate of the reaction nor the path between reactants and products is relevant to the difference in enthalpy or entropy of reactants and products. The reader may consult a physical chemistry book for a fuller explanation of these basic principles of thermodynamics.

Linked Reactions

Many important processes in the cell consist of a single reaction, but most of cellular biochemistry involves a series of linked reactions (Fig. 4-3). For example, when two macromolecules bind together, the complex often undergoes some type of internal rearrangement or conformational change, linking a first-order reaction to a second-order reaction.

Figure 4-3 linked reactions. Two molecules, A and B, bind together weakly and then undergo a favorable conformational change. The binding reaction is unfavorable, owing to the high rate of dissociation of AB, but the favorable conformational change pulls the overall reaction far to the right.

One of thousands of such examples is GTP binding to a G protein, causing it to undergo a conformational change from the inactive to the active state (Figs. 4-6 and 4-7 ahead).

Figure 4-6 Top (A–B), Atomic structures of the small GTPase Ras. GTP hydrolysis and phosphate dissociation cause major changes in the conformations of the switch loops. (A, PDB file: 1Q21.B, PDB file: 121P.) Bottom, Generic GTPase cycle. The size of the arrows indicates the relative rates of the reactions. GAP, GTPase activating protein; G^D, GTPase with bound GDP; GDI, guanine nucleotide dissociation inhibitor; G^DP, GTPase with bound GDP and inorganic phosphate; GEF, guanine nucleotide exchange factor; G^T, GTPase with bound GTP; F_i, phosphate.

Figure 4-7 Kinetic dissection of the Ras gtpase cycle using a series of “single turnover” experiments, in which each enzyme molecule carries out a reaction only once. A, GTP binding. Nucleotide-free Ras is mixed rapidly with a fluorescent derivative of GTP (mGTP), and fluorescence is followed on a millisecond time scale. With 100 mM mGTP (approximately 10% of the cellular concentration), binding is fast (half-time less than 5 ms), but the change in fluorescence is slower, about 30 s⁻¹, since it depends on a subsequent, slower conformational change. Linking the association reaction to this highly favorable (K = 10⁶) first-order conformational change accounts for the exceedingly high affinity (K_d = ˜10⁻¹¹ M) of Ras for GTP. Binding and dissociation of GDP are similar.B, GTP hydrolysis and γ-phosphate dissociation. GTP is mixed with Ras, and hydrolysis is followed by collecting samples on a millisecond time scale with a “quench-flow” device, dissociating the products from the enzyme and measuring the fraction of GTP converted to GDP. The Ras-GDP-P intermediate releases γ-phosphate spontaneously in a first-order reaction. A fluorescent phosphate-binding protein is used to measure free phosphate. On this time scale in this figure, Ras alone does not hydrolyze GTP or dissociated phosphate, since the hydrolysis rate constant is 5 × 10⁻⁵ s⁻¹, corresponding to a half-time of 1400 seconds. The GTPase activating protein (GAP) neurofibromin 1 (NF1) at a concentration of 10 mM increases the rate of hydrolysis to 20 s⁻¹ and allows observation of the time course of phosphate dissociation at 8 s⁻¹. C, GDP dissociation. Ras with bound fluorescent mGDP is mixed with GTP, which replaces the mGDP as it dissociates. The loss of fluorescence over time gives a rate constant for mGDP dissociation of 0.00002 s⁻¹. The guanine nucleotide exchange factor Cdc24^Mn at a concentration of 1 mM increases the rate of mGDP dissociation 500-fold to 0.01 s⁻¹.

(Compiled from experiments reported by Lenzen C, Cool RH, Prinz H, et al: Kinetic analysis by fluorescence of the interaction between Ras and the catalytic domain of the guanine nucleotide exchange factor Cdc24^Mn. Biochemistry 37:7420–7430, 1998; and by Phillips RA, Hunter JL, Eccleston JF, Webb MR: Mechanism of Ras GTPase activation by neurofibromin. Biochemistry 42:3956–3965, 2003.)

Similarly, the basic enzyme reaction considered in most biochemistry books is simply a series of reversible second- and first-order reactions:

where E is enzyme, S is substrate, and P is product. These and more complicated reactions can be described rigorously by a series of rate equations like those explained previously. For example, enzyme reactions nearly always involve one or more additional intermediates between ES and EP, coupled by first-order reactions, in which the molecules undergo conformational changes.

Linking reactions together is the secret of how the cell carries out unfavorable reactions. All that matters is that the total free energy change for all coupled reactions is negative. An unfavorable reaction is driven forward by a favorable reaction upstream or downstream. For example, the unfavorable reaction producing adenosine triphosphate (ATP) from adenosine diphosphate (ADP) and inorganic phosphate is driven by being coupled to an energy source in the form of a proton gradient across the mitochondrial membrane (see Fig. 8-5). This proton gradient is derived, in turn, from the oxidation of chemical bonds of nutrients. To use a macroscopic analogy, a siphon can initially move a liquid uphill against gravity provided that the outflow is placed below the inflow, so that the overall change in energy is favorable.

An appreciation of linked reactions makes it possible to understand how catalysts, including biochemical catalysts—protein enzymes and ribozymes—influence reactions. They do not alter the free energy change for reactions, but they enhance the rates of reactions by speeding up the forward and reverse rates of unfavorable intermediate reactions along pathways of coupled reactions. Given that the rates of both first- and second-order reactions depend on the concentrations of the reactants, the overall reaction is commonly limited by the concentration of the least favored, highest-energy intermediate, called a transition state. This might be a strained conformation of substrate in a biochemical pathway. Interaction of this transition state with an enzyme can lower its free energy, increasing its probability (concentration) and thus the rate of the limiting reaction. Acceleration of biochemical reactions by enzymes is impressive. Enhancement of reaction rates by 10 orders of magnitude is common.

Chemical Bonds

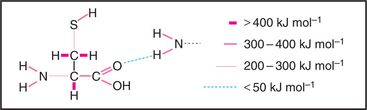
Covalent bonds are responsible for the stable architecture of the organic molecules in cells (Fig. 4-4). They are very strong. C—C and C—H bonds have energies of about 400 kJ mol⁻¹. Bonds this strong do not dissociate spontaneously at body temperatures and pressures, nor are the reactive intermediates required to form these bonds present in finite concentrations in cells. To overcome this problem, living systems use enzymes, which stabilize high-energy transition states, to catalyze formation and dissolution of covalent bonds. Energy for making strong covalent bonds is obtained indirectly by coupling to energy-yielding reactions. For example, metabolic enzymes convert energy released by breaking covalent bonds of nutrients, such as carbohydrates, lipids, and proteins, into ATP (see Fig. 19-4), which supplies energy required to form new covalent bonds during the synthesis of polypeptides. Metabolic pathways relating the covalent chemistry of the molecules of life are covered in depth in many excellent biochemistry books.

Figure 4-4 covalent bonds. Bond energies for the amino acid cysteine.

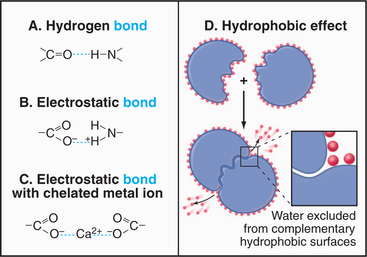
For cell biologists, four types of relatively weak interactions (Fig. 4-5) are as important as covalent bonds because they are responsible for folding macromolecules into their active conformations and for holding molecules together in the structures of the cell. These weak interactions are (1) hydrogen bonds, (2) electrostatic interactions, (3) the hydrophobic effect, and (4) van der Waals interactions. None of these interactions is particularly strong on its own. Stable bonding between subunits of many macromolecular structures, between ligands and receptors, and between substrates and enzymes is a result of the additive effect of many weak interactions working in concert.

Figure 4-5 weak interactions. A, Hydrogen bond. Opposite partial charges in the oxygen and hydrogen provide the attractive force.B, Electrostatic bond. Atoms with opposite charges are attracted to each other. C, Ca²⁺ chelated between two negatively charged oxygens. D, The hydrophobic effect arises when two complementary, apolar surfaces make contact, excluding water molecules that formerly were associated with the surfaces. The increased disorder of the water increases the entropy and provides the decrease in free energy to drive the association. Van der Waals interactions between closely packed atoms on complementary surfaces also stabilize interactions.

A Strategy for Understanding Cellular Functions

One strategy for understanding the mechanism of any molecular process—including binding reactions, self-assembly reactions, and enzyme reactions—is to determine the existence of the various reactants, intermediates, and products along the reaction pathway and then to measure the rate constants for each step. Such an analysis yields additional information about the thermodynamics of each step, as the ratio of the rate constants reveals the equilibrium constant and the free energy change, even for transient intermediates that may be difficult or impossible to analyze separately.

In earlier times, biochemists lacked methods to evaluate the internal reactions along most pathways, but they could measure the overall rate of reactions, such as the steady-state rate of conversion of reactants to products by an enzyme. To analyze these data, they simplified complex mechanisms using relationships such as the Michaelis-Menten equation (described in biochemistry textbooks). Now, abundant supplies of proteins, convenient methods for measuring rapid reaction rates, and computer programs that can be used to analyze complex reaction mechanisms generally make such simplifications unnecessary.

Analysis of an Enzyme Mechanism: The Ras GTPase

This section uses a vitally important family of enzymes called GTPases to illustrate how enzymes work. The example is Ras, a small GTPase that serves as part of a biochemical pathway linking growth factor receptors in the plasma membrane of animal cells to regulation of the cell cycle. The example shows how to dissect an enzyme reaction by kinetic analysis and how crystal structures can reveal conformational changes related to function. GTPases related to Ras regulate a host of systems (see Table 25-3) including nuclear transport (see Fig. 14-17), protein synthesis (see Figs. 17-9 and 17-10), vesicular trafficking (see Fig. 21-6), signaling pathways coupled to seven-helix receptors including vision and olfaction (see Figs. 25-8 and 25-9), the actin cytoskeleton (see Figs. 33-17 and 33-20), and assembly of the mitotic spindle (see Fig. 44-8). This section gives the reader the background required to understand the contributions of GTPases to all of these processes as they are presented in the following sections of the book.

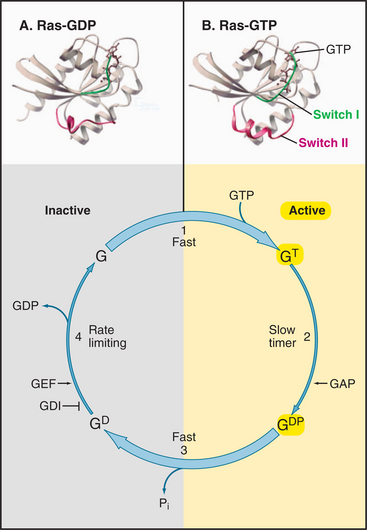
Having evolved from a common ancestor, Ras and its related GTPases share a homologous core domain that binds a guanine nucleotide and use a common enzymatic cycle of GTP binding, hydrolysis, and product dissociation to switch the protein on and off (Fig. 4-6). The GTP-binding domain consists of about 200 residues folded into a six-stranded β-sheet sandwiched between five α-helices. GTP binds in a shallow groove formed largely by loops at the ends of elements of secondary structure. A network of hydrogen bonds between the protein and guanine base, ribose, triphosphate, and Mg²⁺ anchor the nucleotide. Larger GTPases have a core GTPase domain plus domains required for coupling to seven-helix receptors (see Fig. 25-9) or regulating protein synthesis (see Figs. 17-10 and 25-7).

The bound nucleotide determines the conformation and activity of each GTPase. The GTP-bound conformation is active, as it interacts with and stimulates effector proteins. In the example considered here, the Ras-GTP binds and stimulates a protein kinase, Raf, which relays signals from growth factor receptors to the nucleus (see Fig. 27-6). The GDP-bound conformation of Ras is inactive because it does not bind effectors. Thus, GTP hydrolysis and phosphate dissociation switch Ras and related GTPases from the active to the inactive state.

All GTPases use the same enzyme cycle, which involves four simple steps (Fig. 4-6). GTP binding favors the active conformation that binds effector proteins. GTPases remain active until they hydrolyze the bound GTP. Hydrolysis is intrinsically slow, but binding to effector proteins or regulatory proteins can accelerate this inactivation step. GTPases tend to accumulate in the inactive GDP state, because GDP dissociation is very slow. Specific proteins catalyze dissociation of GDP, making it possible for GTP to rebind and activate the GTPase. Seven-helix receptors activate their associat-ed γ-proteins. Guanine nucleotide exchange proteins (GEFs) activate small GTPases.

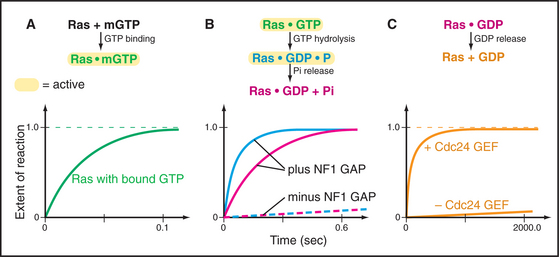
Figure 4-7 illustrates the experimental strategy used to establish the mechanism of the Ras GTPase cycle.

Step 1: GTP binding.

Step 2: GTP hydrolysis.

Step 3: Dissociation of inorganic phosphate.

Step 4: Dissociation of GDP.

Ras and most other small GTPases depend on regulatory proteins to stimulate the two slow steps in the GTPase cycle: GDP dissociation and GTP hydrolysis. For example, when growth factors stimulate their receptors, a series of reactions (see Fig. 27-6) brings a guanine nucleotide exchange factor (GEF) to the plasma membrane to activate Ras by accelerating dissociation of GDP. First the GEF binds Ras-GDP and then favors a slow conformational change that distorts a part of Ras that interacts with the β-phosphate. This allows GDP to dissociate on a time scale of seconds to minutes rather than 10 hours (Fig. 4-7C). Once GDP has dissociated, nucleotide-free Ras can bind either GDP or GTP. Binding GTP is more likely in cells, because the cytoplasmic concentration of GTP (about 1 mM) is 10 times that of GDP. GTP binding activates Ras, allowing transmission of the signal to the nucleus.

GTPase-activating proteins (GAPs) turn off Ras and related GTPases, by binding Ras-GTP and stimulating GTP hydrolysis, thereby terminating GTPase activation (Fig. 4-7B). Ras GAPs stabilize the transition state, by contributing a positively charged arginine side chain that stabilizes the negative charges on the oxygen bridging the β- and γ-phosphates and on the γ-phosphate. GAPs also help to position Gln61 and its attacking water. In the experiment in the figure, a GAP called neurofibromin (NF1) binds Ras with a half-time of 3 ms (not illustrated) and stimulates rapid hydrolysis of GTP at 20 s⁻¹. This is followed by rate-limiting dissociation of γ-phosphate from the Ras-GDP-P intermediate at 8 s⁻¹ and rapid dissociation of NF1 from Ras at 50 s⁻¹. NF1 is the product of a human gene that is inactivated in the disease called neurofibromatosis. Lacking the NF1 GAP activity to keep Ras in check, affected individuals develop numerous neural tumors that disfigure the skin and may compromise the function of the nervous system.

ACKNOWLEDGMENT

Thanks go to Martin Webb for his help with GTPase kinetics.