Orthognathic Surgery Treatment Planning
Part I: The Importance of Proportions and Symmetry to Establish Ideal Facial Aesthetics
Fundamental Concepts
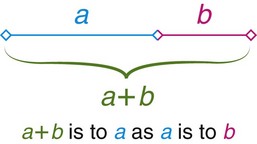
We use the word proportion to describe the comparative relationship between parts of things in an attempt to describe harmonious and disharmonious relationships between different parts of a whole.13,14,49,92 The first clear and still unsurpassed definition of what has become known as the “golden ratio” was put into words by Euclid of Alexandria around 300 B.C.5,29,42–44,61 Euclid is best known as the founder of geometry, which is a branch of mathematics that involves the use of deductive reasoning.68 In the most pure way, Euclid defined a harmonious proportion derived from the simple division (sectioning) of a line into what he called its “extreme and mean ratio.”61 In Euclid’s own words, “[a] straight line is said to have been cut in extreme and mean ratio when, as the whole line is to the greater segment, so is the greater to the lesser.”98,99 (Fig 12-1) This ratio was defined by Euclid as the “golden ratio.” Interestingly, this ratio represents a never-ending (irrational) number 1.6180339887…. The common symbol for the golden ratio is the Greek letter tau (τ), the meaning of which is “the cut” or “the section.” It was later renamed phi (Ψ) after the Greek sculptor Phidias, who was said to have employed it61; this is described in more detail later in this chapter.
A definition of the word beauty can be found in Webster’s unabridged dictionary as “the quality which makes an object seem pleasing or satisfying in a certain way.”63 The golden ratio is said to be an important feature that is found in many classically beautiful works of art, architectural structures, objects designed for human use, the music of the great composers, and most of what is found in nature.17,18,20,45,49,57,58,67,68 “Perfect” proportions are found throughout the art world and throughout the centuries. Objects of “classic” beauty and “attractive” forms found in nature consistently reveal the golden ratio.40 However, an important question remains: “Does form really follow function?” Richard Buckminster Fuller (1895-1983), a great American architect and writer, once stated, “When I am working on a problem, I never think about beauty. I think only of how to solve the problem. But when I finish, if the solution is not beautiful, I know it is wrong.”61
In their simplest form, attractive facial images—whether in drawings, in photographs, or in reality—seem to incorporate a consistent symmetry of proportion. It has been stated that most people have a common appreciation of what is attractive when they visualize it even if they cannot quite explain why. This has been demonstrated across cultures and throughout the ages, and it has been relatively consistent in art and photography.6,19,27,32,48,53,54,63,77,81,80 A strict mathematical formula may not be required to define classic attractiveness, but the question remains: “Can the golden ratio (a mathematical formula) be found in these commonly agreed upon beautiful images?”
Evolution in Thinking
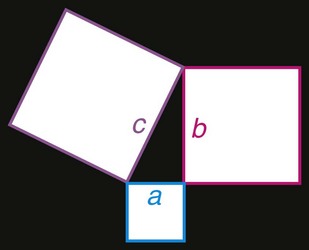
Pythagoras (570 B.C.-522 B.C.) coined the terms philosophy, defined as “a love of wisdom,” and mathematics, defined as “that which is learned.”47 He described a philosopher as someone who “gives himself up to discovering the meaning and purpose of life itself—to uncover the secrets of nature.”100,42,61 Pythagoras and his followers (i.e., Pythagoreans) are best known for their role in the development of mathematics and the application of mathematic principles to the concept of order in the world. The Pythagorean theorem states that, in a right-angle triangle, the square of the hypotenuse (i.e., the side opposite the right angle) is equal to the sum of the squares of the other two sides; in other words, a2 + b2 = c2 (Fig. 12-2).9
According to legend, Pythagoras discovered that musical notes could be translated into an orderly mathematical equation by observing a blacksmith at work. He thought that the sounds emanating from the blacksmith’s anvils, when they were struck, were both beautiful and harmonious. By studying the blacksmith’s tools, Pythagoras discovered that the emanating sounds were “simple ratios of each other and that one was half the size of the first and so on.”61,100 He then constructed metal bells with each sized in equal ratio to the next. When he struck the proportionately sized bells, harmonious tunes resulted in the same way that he had observed with the anvils. The harmony that resulted when striking the proportionately sized bells or anvils, according to Pythagoras, was beautiful.
Pythagoreans also believed that “harmony existed with the spheres of the planets and stars according to a similar strict mathematical equation and thus produced a similar “planetary symphony.”61,100 Pythagoras later discovered flaws in the purity of mathematics. He was the first to write of having knowledge of a number that is “neither a whole—nor a ratio of two whole numbers.”61,100 This discovery was said to have overwhelmed his perspective of a world based on the intrinsic properties of whole numbers and their ratios. He could not find a role for irrational numbers in this world.44 Pythagoreans believed that the existence of such irrational numbers was so horrific that such numbers represented some sort of cosmic mistake and thus should be suppressed and kept secret.
Pythagoras and the Pythagorean school made a great impact in various fields, from sculpture to philosophy.55 Polykleitos (450 B.C.) is a vivid example of the positive influence of Pythagoras’ ideas. Polykleitos consciously created a new approach to sculpture that is immortalized in his treatise Kanon and visually depicted in his design of the male nude (also known as Kanon), which exemplified his aesthetic theories regarding the mathematical basis of artistic perfection.69 His general artistic aims were clarity, balance, and completeness. The Kanon‘s basic principles are represented in Polykleitos’ best-known bronze sculptures, including Doryphoros (Fig 12-3); Diadumenos (Fig 12-4); and Astragalizontes. These sculptures are said to have a precise commensurability (i.e., “symmetria”) of all of the parts with one another. The idea of “symmetria” comes from the Hippocratic principles of “equilibrium” and “rhythmos.”100 Polykleitos also said that “perfection comes about little by little through many numbers.”61,100 This meant that a statue should be composed of clearly definable parts, all of which are related to one another through a system of ideal mathematical proportions and balance. This was expressed in terms of the ratios established by Pythagoras, including in the perfect intervals of the musical scale: 1 : 2 (octave), 2 : 3 (harmonic fifth), and 3 : 4 (harmonic fourth).

Figure 12-3 Doryphoros by Polykleitos. From http://en.wikipedia.org/wiki/File:Doryphoros_MAN_Napoli_Inv6011-2.jpg Photograph by Marie-Lan Nguyen. Creative Commons License: Attribution.

Figure 12-4 Diadumenos by Polykleitos. Image Copyright © The Metropolitan Museum of Art, Image source: Art Resource, NY.
The Polykleitos school continued after Polykleitos’ death and included at least 20 sculptors and artisans through early 200 B.C. They were defined by their adherence to the Kanon and its principles of balance and definition (i.e., clarity in the separation and relationship of the parts to the whole). Polykleitos’ students would think beyond sculpture in bronze; this included the work of Polykleitos’ son, Polykleitos the Younger, whose architectural design of the great theater of Epidaurus was remarkable for its harmony and balance (Fig. 12-5).100 Within the theater, the place for spectators was divided into two tiers: the first one had 34 rows of places, and the second one had 21 rows (i.e., 34/21 = 1.61…). The angle between the theatron and the scene divides the circumference of the basis of the amphitheater in a ratio of 1.618…. Interestingly, both of these meet the standards of the golden section, which was soon to be precisely and clearly defined by Euclid, as described later in this chapter.

Figure 12-5 Theater of Epidaurus in Epidaurus, Greece. Copyright 2013 BootsnAll Travel Network, LLC. All Rights Reserved. http://www.greecelogue.com/the-ancient-theater-of-epidaurus.html.
Polykleitos’ greatest work is said to be the sculpture Doryphoros (i.e., “spear-bearer”), which is considered one of the best-known sculptures of the classic era.100 During the second century A.D., Galen would write about Doryphoros as the perfect visual expression of Greece’s search for harmony and beauty, which is rendered in the perfectly proportioned sculpted male nude. Galen stated the following:
Chrysippos [or Doryphoros, the spear-bearer] holds beauty to consist not in the commensurability or “symmetria” [i.e., proportions] of the constituent elements [of the body], but in the commensurability of the parts, such as that of finger to finger, and of all the fingers to the palm and wrist, and of those of the forearm, and of the forearm to the upper arm, and in fact, of everything to everything else, just as it is written in the Canon of Polyclitus [Polykleitos]. For having taught us in that work all the proportions of the body, Polyclitus supported his treatise with a work: he made a statue according to the tenets of his treatise and called the statue, like the work, the “Canon” [i.e., Kanon].61,100
A contemporary of Polykleitos was Phidias (480 B.C.),100 who is regarded as one of the greatest sculptors of bronze, gold, and ivory from Greek antiquity. Phidias’ statue of Zeus at Olympia was considered one of the seven manmade wonders of the Ancient World (Fig. 12-6). He also created the statue of the Goddess Athena on the Athenian Acropolis (Fig. 12-7) as well as the Athena Promachos (i.e., a bronze statue of Athena) adjacent to the entrance of the Acropolis in Athens (Fig. 12-8). The influence of Pythagorean mathematics, the Kanon (i.e., the relationship of the parts to the whole), and the golden ratio (although not spoken of as a specific number) are clearly represented in all of Phidias’ works.

Figure 12-8 Statue of the Goddess Athena at the entrance of the Athenian Acropolis by Phidias. From http://en.wikipedia.org/wiki/File:NAMA_Ath%C3%A9na_Varvakeion.jpg Photograph by Marsyas. Creative Commons License: Attribution.
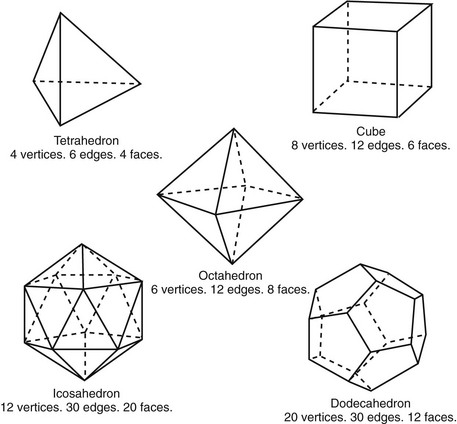
Pythagoras’ writings also influenced Plato (428 B.C.-337 B.C.), who is considered one of the most extraordinary minds of Ancient Greece and of Western civilization in general.100 His text, the Republic, states that mathematics is an absolute must for the education of all state leaders and philosophers (Fig. 12-9). Plato was intrigued by the five regular polyhedrons that are now considered the Platonic solids (Fig. 12-10). He used their design to emphasize the importance of proportions for the achievement of “ideal” beauty. His thinking must have also been greatly influenced by Polykleitos’ sculptures and written words in the Kanon. Plato is quoted as follows:
Two parts or values cannot be satisfactorily connected among themselves without a third part; the most beautiful link is that, which together with two initial values gives the perfect unit. It is reached in the best way by proportion [analogy], in which among three numbers, planes or bodies, the mean one so concerns to the second one, as the first one to the mean one, and also the second one to the mean one as the mean one to the first one. This implies, that the mean one can exchange the first one and the second one, the first one and the second one can exchange the mean one and all things together thus makes an indissolvable unit.61,100
Plato’s favored student Aristotle (384 B.C.-?) was able to take Pythagorean ideas and the Kanon of Polykleitos a step further forward when he wrote that the main requirements of beauty include order, proportionality, and control of sizes (i.e., the relationship of the parts to each other).100 Order was said to arise “when between parts of the whole there are definite ratios in proportions.”61,100 In music, for example, Aristotle recognized the octave as the most beautiful consonance when taking into consideration that the number of oscillations between the basic tone and the octave can be expressed by the first numbers of a natural series: 1 : 2. He also had the opinion that rhythmic relations of a poetic verse should be based on small numerical ratios to reach a beautiful impression.41 Like Plato, Aristotle believed that beauty was commensurate of the separate parts to the whole.
The young Alexander the Great of Macedonia (Fig. 12-11) succeeded to the throne after his father’s assassination (336 B.C.). He went on to conquer most of Asia Minor, Syria, Egypt, and Babylon, and he then founded the City of Alexandria near the mouth of the Nile. Alexandria developed into the intellectual center of the hemisphere, and it included a library that is said to have held more than 700,000 books. The first group of scholars at the center included the young Euclid (300 B.C.), who authored a complete text about the history of mathematics that included many of his own original ideas (Fig. 12-12). The Elements was a 13-volume work on geometry and number theory.43 Each book contained a number of theorems (i.e., from 10 to 100) that follow a series of definitions. Scholars believe that the Elements is primarily a collection of theorems described by other mathematicians that was then supplemented by at least some original work by Euclid. Proclus, a Greek mathematician who lived several centuries after Euclid, wrote the following in his commentary about the Elements: “Euclid, who put together the Elements, collecting many of Eudoxus’ theorems, perfecting many of Theaetetus, and also bringing to irrefutable demonstration the things which were only somewhat loosely proven by his predecessors.”5 For example, Pythagoras was probably the source of most of Books 1 and 2; the ideas of Hippocrates were found in Book 3, and those of Eudoxus were in Book 5; Books 4, 6, 11, and 12 probably came from other Pythagorean or Athenian mathematicians. Euclid is credited with replacing Fallacious’ proofs with his own more rigorous versions. Most of the definitions, postulates, and axioms within the Elements date back to Plato. Euclid also authored almost a dozen other books that covered topics from music to mechanics and optics. His book Optics contains some of the earliest studies of perspective. Interestingly, until the 20th century, Euclid’s Elements remained second only to the Bible with regard to the number of books sold worldwide. Euclid’s textbook, through various translations, has remained actively referenced for more than 2300 years.

Figure 12-11 Sculpture of Alexander the Great of Macedonia. From http://en.wikipedia.org/wiki.File:Alexander1256.jpg
The golden ratio or golden section appears in the Elements, primarily in relation to proportions in Book 6: On Divisions of Figures.43 Book 6 survives only partially in an Arabic translation, and it discusses the division of geometric figures into two or more parts or into parts in given ratios. It is likely that Euclid’s primary interest in the golden ratio was as a means of geometric interpretation of the construction of the five Platonic solids. This discussion is also found in Book 13, which constructs the five regular Platonic solids that are inscribed within a sphere. Euclid calculated the ratio of their edges to the radius of the sphere and proved that there are no other regular solids to be found. Curiosity about the structure and design of these Platonic Solids were of much interest to Ancient Greek intellectuals.47 In addition, throughout the Elements, Euclid described several theorems and their proofs, which also employed the golden ratio. Although no documentation has been found to confirm Euclid’s knowledge of Polykleitos’ Kanon or Aristotle’s stated requirements of beauty (i.e., order, proportionality, and control of sizes), it is almost inconceivable that these ideas did not influence him.
Marcus Vitruvius Polio (80 B.C.-15 B.C.) came two centuries after Euclid; he was a Roman writer, architect, and engineer. At one point, he even served as Chief of Artillery for the Roman Army. His written work De Architectura, a 10-volume treatise on architecture, is not just remarkable for its originality and contents but also for being the only surviving major book about architecture from classic antiquity (Fig. 12-13). This treatise indicates a mastery of architecture and engineering, and it crystallizes the importance of precise proportions as a fundamental component of beauty in the human form. Interestingly, in the records that remain, no mention is made by Vitruvius of Pythagoras, Polykleitos, or Euclid. This lack of acknowledgement by Vitruvius is striking in its absence; thus, it likely represents lost documents rather than a lack of awareness.
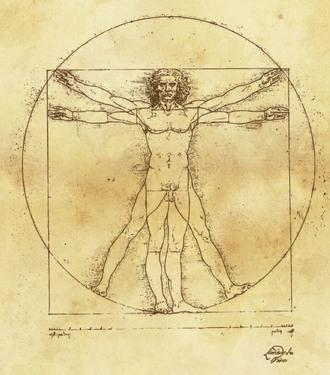
Fortunately, Vitruvius’ treatise would later be rediscovered by the Florentine humanist Poggio Bracciolini (1414). It was Leon Battista Alberti (1404-1472) who then revitalized Vitruvius’ works by writing a widely read seminal treatise on architecture, De Re Aedificatoria, which was published in 1450.35 During the High Italian Renaissance, this volume had a great influence on artists, scholars, and architects, including Leonardo da Vinci (1452-1519) and Michelangelo (1475-1564).72 Vitruvius is most remembered for his basic assertion that a structure must exhibit the three qualities of firmitas, utilitas, and venustas: it must be strong (durable), useful, and beautiful. According to Vitruvius, architecture is an imitation of nature: “As birds and bees built their nests, so humans construct housing, from natural materials that give them shelter against the elements.”61 When perfecting the art of building, ancient greeks designed and defined the three architectural orders: Doric (Fig. 12-14), Ionic (Fig. 12-15), and Corinthian (Fig. 12-16).100 These architectural orders gave the Ancient Greek buildings a sense of proportion and continuity that was believed to be as important as the proportions of the greatest known work of art: the human body. This led Vitruvius to define the proportions of the human body in words that would later be illustrated by Leonardo da Vinci’s Vitruvian Man (1437; Fig. 12-17). The human body as described by Vitruvius was inscribed in a circle and in a square. These are considered the fundamental geometric patterns of the cosmic order.102
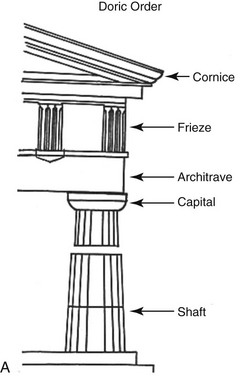

Figure 12-14 A, Illustrations of Doric architecture. B, Doric architecture as demonstrated by the Parthenon from Ancient Greece. Courtesy Charlotte-Mecklenburg Historic Landmarks Commission. http://www.cmhpf.org/kids/dictioary/classicalorders.html
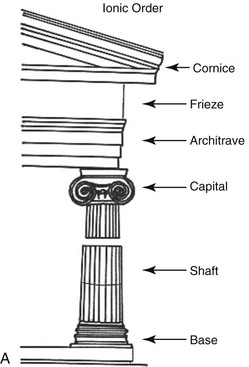

Figure 12-15 A, Illustrations of Ionic architecture. B, Ionic architecture as demonstrated by the Temple of Athena Nike from Ancient Greece. Courtesy Charlotte-Mecklenburg Historic Landmarks Commission. http://www.cmhpf.org/kids/dictioary/classicalorders.html
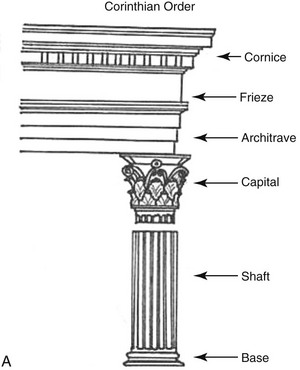

Figure 12-16 A, Illustrations of Corinthian architecture. B, Corinthian architecture as demonstrated by the Temple of the Sybil from Ancient Greece. Courtesy Charlotte-Mecklenburg Historic Landmarks Commission. http://www.cmhpf.org/kids/dictionary/classicalorders.html.
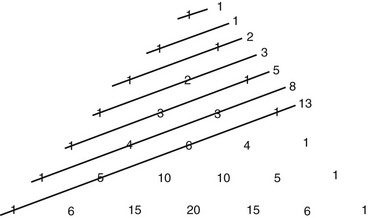
Abu Kmil’s books would later serve as a basis for Leonardo of Pisa’s further understanding of Euclid’s geometric principles. Leonardo of Pisa (1170-1250) was also known as Fibonacci, Leonardo Bonacci, Leonardo Pisano Bogollo, and Leonardo Fibonacci; he was considered the most talented mathematician of the late middle ages.25 He is best remembered by the modern world for spreading knowledge of the Hindu-Arabic numeral system (i.e., digits 0 through 9) in Europe; this was primarily done through publication during the earlier 13th century of the Book of Calculations or Liber Abaci.97 His second contribution was the recognition of a number sequence that became known as the Fibonacci numbers (Fig 12-18). Within the Liber Abaci, Fibonacci posed and solved a classic problem involving the growth of a hypothetical population of rabbits that was based on idealized assumptions (Fig 12-19). The solution was a sequence of numbers that was later named after Fibonacci. Although this number sequence was known to Indian mathematicians as early as the sixth century, it was Fibonacci’s Liber Abaci that introduced it to the West.101 In the sequence of the Fibonacci numbers, each number is the sum of the previous two numbers, starting with 0 and 1. Thus, the sequence begins 0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, and so on. Interestingly, the higher up in the sequence, the closer two consecutive numbers in the sequence—when divided by each other—will approach the golden ratio of approximately 1 : 1.618 or 0.618 : 1. The sequence of the Fibonacci numbers and their relative ratios have been found with consistency in nature, art, and classic objects of beauty. They would soon be recognized and used by the Renaissance painters.
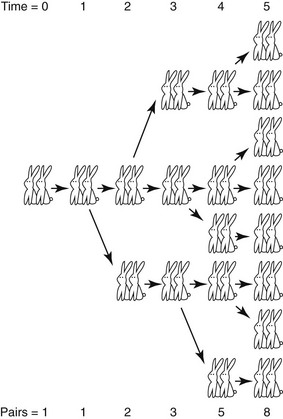
Figure 12-19 Fibonacci numbers as described through breeding rabbits. The sequence 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, and so on—in which each term (starting with the third) is equal to the sum of the two preceding terms—was appropriately dubbed the Fibonacci numbers during the 19th century by the French mathematician Edouard Lucas (1842-1891).
The Fibonacci numbers and the golden ratio are found in the most basic forms in nature.25 For example, spirals arise from a property of growth called “self-similarity” (i.e., the tendency to grow in size but to maintain the same shape). This is seen in the simplest of natural forms: the shell of the chambered nautilus. As the nautilus outgrows each chamber, it builds a new chamber for itself, but this always has the same shape (Fig. 12-20). If the nautilus lives a long life, it will spiral around and around, growing even larger but always looking exactly the same at every scale. Interestingly, by using the Fibonacci sequence of numbers, you can build a nautilus that is based on squares by starting with a square of size 1 and successively building new “rooms” with sizes that correspond with the Fibonacci sequence.39 Running through the centers of the squares, a smooth curve can be drawn that corresponds to the nautilus spiral (see Fig. 12-20, B). This self-similar curve, which keeps its shape at every scale, is called equiangular because a radial line from the center always makes the same angle to the curve. This curve was also known to Archimedes of Ancient Greece, who—philosophically and religiously—thought of this curve as both spiraling inward forever as well as spiraling outward forever. Euclid’s golden ratio mathematically describes this finding both in nature and in the Fibonacci numbers.
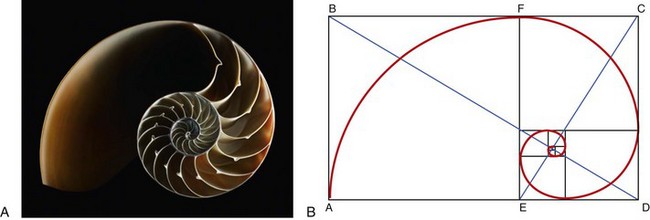
Figure 12-20 A, Photograph of the chambered nautilus, which demonstrates structural anatomy. B, The golden ratio as found in the spiral structural anatomy of the nautilus.
Euclid’s ideas have had an enormous impact throughout the centuries,5 first on the medieval Italian philosopher St. Thomas Aquinas (1225-1274) and then on the Renaissance Italian architect L.B. Alberti (1404-1472).72 Aquinas captured the fundamental relationship between beauty and mathematics when he stated that “humans seem to react with a sense of pleasure to forms that possess certain symmetries [proportions] and obey certain mathematical rules.”35,61
Leon Battista Alberti was an author, artist, architecture, poet, priest, linguist, philosopher, cryptographer, and general Renaissance humanist. Alberti regarded mathematics (as summarized by Euclid) to be the common ground of the arts and the sciences. For Alberti, beauty was “the harmony of all parts in relation to one another.” He stated that “this concord is realized in a particular number, proportion and arrangement demanded by harmony.”35,61 Alberti’s thoughts on harmony were referenced back to Pythagoras and Euclid, but he set them in a fresh context. He studied Ancient Roman sites, ruins, and objects and made detailed observations that are cataloged in his Ten Books of Architecture (De Re Aedificatoria), which was published in 1450 A.D. His writings were patterned after the De Architectura by Vitruvius (46 B.C.-30 B.C.) and covered a wide range of subjects, from history to town planning to engineering to the philosophy of beauty.
Three Renaissance genius mathematicians and artists were clearly influenced by Euclid’s ideas72: Piero della Francesca (1415-1492), Leonardo da Vinci (1452-1519), and Luca Pacioli (1446-1517). Piero della Francesca was known as a mathematician, a geometer, and an artist. His painting was characterized by its serene humanism and its use of geometric forms, particularly in relation to perspective and foreshortening (Fig. 12-21). It is known that he was at least acquainted with Leon Battista Alberti’s mathematical and architectural work and that he would also have a significant influence on Luca Pacioli’s thinking.71 Three important mathematical treatises were written by Piero: Abacus Treatise (Trattato d’Abaco); Short Book on the Five Regular Solids (Libellius de Quinque Corporibus Regularibus); and Perspective on Painting (De Prospectiva Pingendi). The subjects covered in these writings include mathematics, algebra, geometry, and innovative work on both solid geometry and perspective. Much of Piero’s work would later be rewritten by others, including Luca Pacioli.71 Piero’s work on solid geometry appears in Pacioli’s De Divina Proportione, which is believed to have been illustrated by Leonardo da Vinci. Piero’s Perspective on Painting (De Prospectiva Pingendi) clearly refers to Euclid’s Elements and Optics. Piero used Euclid’s written works to demonstrate that, to achieve perspective in a painting, the artist must rely on the scientific basis of visual perception. Piero’s treatise De Prospectiva Pingendi became the standard manual of the day for artists who attempted to paint plain figures and solids.
Luca Pacioli (Fra Luca Bartolomeo de Pacioli) lived from 1446 to 1517. He was a Franciscan friar and an Italian mathematician.71 He later collaborated with Leonardo da Vinci, and he also made seminal contributions to the field that is now known as accounting. In 1494, his first book—Summa di Aritmetica, Geometria, Proporzioni e Proporzionalità was published in Venice. This text compiled the knowledge of the time related to arithmetic, algebra, geometry, and trigonometry. Pacioli identified Fibonacci (Leonardo of Pisa) as the primary source for his basic facts. Several years later, he met, collaborated with, lived with, and taught mathematics to Leonardo da Vinci. Pacioli also completed the three-volume treatise The Divine Proportion (De Divina Proportione), which applies to geometrical proportions, letters, and architecture constructions. The original manuscript, which was written around 1496 to 1498, is preserved in Milan; it was published in Venice in 1509.71 Within the text, Pacioli lists five reasons why a more appropriate name for Euclid’s golden ratio would be “the divine proportion.” References and analogies to the church and godliness clarify Pacioli’s belief that the divine proportion is spiritual and universal. He was also interested in the process of painting, and he emphasized his desire to reveal the secret to the creation of harmonic forms through the use of the golden ratio (or the divine proportion). Pacioli used the square and the compass (which are clearly visible in the background of his well-known self-portrait) to both analyze the works of others and to help artists achieve divine proportions in their paintings (Fig. 12-22). It is believed that Pacioli invited Leonardo da Vinci to provide 60 illustrations, primarily of solid objects, to clarify the importance of the divine proportion. (Fig. 12-23). Leonardo’s drawings are the first illustrations of geometric figures in the dual state of skeletonic (vacuum) and solid (solidum) (Fig. 12-24). This allowed for a distinct interpretation between front and back. Pacioli wrote of da Vinci’s contributions to society by stating that “he is the most excellent painter in perspective, architect, musician and man endowed with all virtues.”71 Pacioli’s text largely restates the earlier published work of the Roman architect Marcus Vitruvius Polio.5 Within the text, Pacioli states the following:
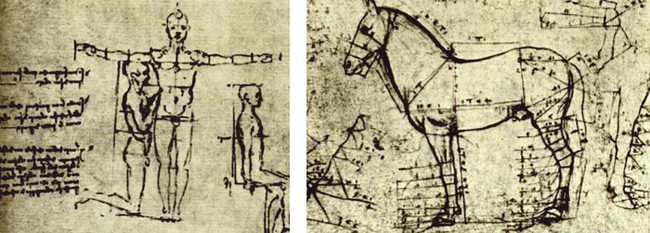
Figure 12-23 Drawings by Leonardo da Vinci confirming the golden ratio found in humans and other mammals. From Royal Collection Trust/©Her Majesty Queen Elizabeth II 2013.

Figure 12-24 Virgin of the Rocks by Leonardo da Vinci, confirming the golden ratio. From Stamperia d’Arte Fratelli Alinari. Bibliotecca Reale, Turin, Italy. Photo Credit: Alinari/Art Resource, NY.
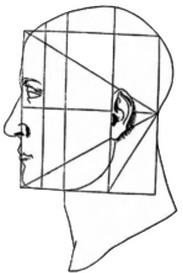
It is clear from a woodcut sketch included in De Divina Proportione (1509) that Luca Pacioli understood the importance of the golden ratio as it applies to the human face (Fig. 12-25). It is also believed that, at the request of Pacioli, Leonardo da Vinci took Vitruvius’ written words and drew them out in a diagram that he called Vitruvian Man (1487; see Fig 12-17).61 The image depicts a male figure in two superimposed positions with his arms and legs apart and simultaneously inscribed in a circle and a square. The illustrated interpretation of Vitruvius’ words is sometimes also called the Canon of Proportions; the original drawing is stored in the Galleria dell’Accademia in Venice, Italy. It is known that Vitruvius described the human figure as being the principal source of correct proportions and symmetry among the classic orders of architecture. da Vinci’s Vitruvian Man beautifully demonstrates the blend of art and science envisioned by intellectuals during the renaissance, and it provides a clear understanding of the artist’s keen interest in the proportions of the human form. Like Plato, da Vinci believed the workings of the human body to be an analogy of the workings of the universe. According to Leonardo himself, in a note that accompanied the drawing, “it was made as a study of the proportions of the male human body as described by Vitruvius” (“Palmus autem habet quattuor digitos”).61,72 Vitruvius’ words, which Leonardo attempted to draw out in pen and ink on paper, are found in Vitruvius’ De Architectura 3.1.2-3, which reads as follows:
For the human body is so designed by nature that the face, from the chin to the top of the forehead and the lowest roots of the hair, is a tenth part of the whole height; the open hand from the wrist to the tip of the middle finger is just the same; the head from the chin to the crown is an eighth, and with the neck and shoulder from the top of the breasts to the lowest roots of the hair is a sixth; from the middle of the breasts to the summit of the crown is a fourth. If we take the height of the face itself, the distance from the bottom of the chin to the underside of the nostrils is one-third of it; the nose from the underside of the nostrils to a line between the eyebrows is the same; from there to the lowest roots of the hair is also a third, comprising the forehead. The length of the foot is one-sixth of the height of the body; of the forearm, one-fourth; and the breadth of the breasts is also one-fourth. The other members, too, have their own symmetrical proportions, and it was by employing them that the famous painters and sculptors of antiquity attained to great and endless renown. Similarly, in the members of a temple, there ought to be the greatest harmony in the symmetrical relations of the different parts to the general magnitude of the whole. Then again, in the human body, the central point is naturally the navel. For if a man is placed flat on his back, with his hands and feet extended, and a pair of compasses centered at the navel, the fingers and the toes of his two hands and feet will touch the circumference of a circle described therefrom. Just as the human body yields a circular outline, so too a square figure may be found from it. For if we measure the distance from the soles of the feet to the top of the head, and then apply that measure to the outstretched arms, the breadth will be found to be the same as the height, as in the case of plain surfaces which are perfectly squared.61,72
For some scholars and artists of the High Italian Renaissance, the golden ratio was elevated from a simple mathematical formula to become a way of explaining natural phenomena and a tool to be used in the painting of objects and human forms.66,93 By the mid 1400s, advances in technology allowed artists to use lenses, concave mirrors, and other methods to help them to create realistic-looking paintings. Many of today’s critics believe that, simply by using their innate aesthetic sense, talented artists, architects and designers of that time period created art, built structures, and designed objects that closely approximate the golden ratio.46 Skeptics often claim that the most attractive and harmonious objects were methodically designed by their artists to adhere to the golden ratio measurements; they also believe that many of the great artists’ designs were simply superimposed on laid-out golden ratios. Interestingly, many other scholars deny that the Greeks (e.g., Euclid) made any direct aesthetic associations with the golden ratio. Thus, some questions remain unanswered: Throughout antiquity and during the Renaissance, did artists consciously use the golden ratio as part of their technique? Did they model their work on precise mathematical dimensions, or was their aesthetic and artistic sense the guiding force that inevitably closely approximated the golden ratio?
The analysis of Leonardo’s drawing entitled A Head of an Old Man (Fig. 12-26) leaves little doubt that he was, at the very least, intrigued by various proportions of the face. This drawing shows how Leonardo used standard rectangles to determine dimensions in his paintings. Because classic art requires “harmony” and because we know that Leonardo collaborated with Pacioli and that he was believed to be the illustrator of Divine Proportions, Leonardo’s use of the golden ratio in his paintings would be an obvious extension of his mathematic knowledge and artistic principles. Most of today’s art critics place Leonardo’s Mona Lisa on a short list of the most beautiful faces ever painted (Fig. 12-27). Debate continues regarding Leonardo’s conscious use of the golden ratio in this well-known work. Interestingly, da Vinci is said to have revised the Mona Lisa for more than 10 years before his death without ever fully finishing the work to his own satisfaction.
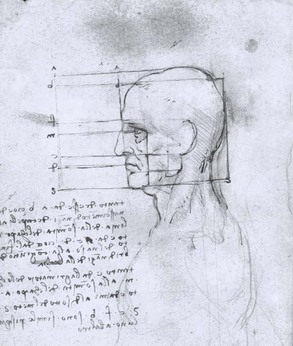
Figure 12-26 Head of an Old Man by Leonard da Vinci, confirming the golden ratio. From Royal Library, Windsor Castle, Windsor, Great Britain. Photo Credit: Alinari/Art Resource, NY.
After Leonardo, the golden ratio as an artistic principle likely fell from favor. To my knowledge, no prominent philosopher or artist was known to have actively used the golden ratio again until Adolf Zeising (1810-1876).105 Zeising was formally trained in psychology, mathematics, and philosophy. He found the golden ratio expressed in all things, including in the skeletons of animals and the branching of their veins and nerves (Fig. 12-28). In connection with his theory involving golden-ratio–based human body proportions, Zeising (1854) wrote what he called the “universal law of proportions,” “in which is contained the ground-principle of all formative striving for beauty and completeness in the realms of both nature and art, and which permeates, as a paramount spiritual ideal, all structures, forms and proportions, whether cosmic or individual, organic or inorganic, acoustic or optical; which finds its fullest realization, however, in the human form.”34
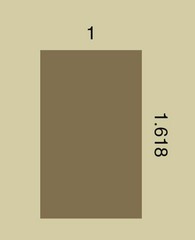
The famous German psychologist, Gustav Fechner, was inspired by Adolf Zeising’s book Der Goldene Schnitt and began a series of inquiries to see if the golden rectangle had psychological aesthetic impact.34 A golden rectangle is one with side lengths that reflect the golden ratio. A distinctive feature of this shape is that, when a square section is removed, the remainder creates another golden rectangle. In other words, it has the same proportions as the first. Another section can be removed infinitely, with the same results. Fechner’s work was published in 1876. He made thousands of measurements of commonly seen rectangles (e.g., writing pads, books, playing cards, windows) and found that most were close to phi (Fig. 12-29). He also tested people’s preferences and found that most people prefer the shape of the golden rectangle. Fechner’s experiments were repeated and validated by others, including Witmar (1894), Lalo (1908), and Thorndike (1917).34
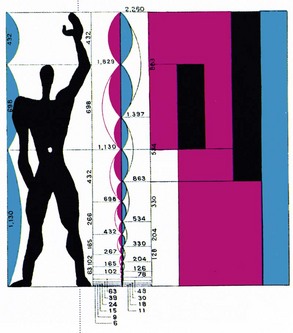
By the late 1800s, knowledge of and the conscious use of the golden ratio by painters, architects, and designers was well documented.34 There are clear examples where these professionals have modeled their end products to conform with Euclid’s golden ratio. In 1946 in The Geometry of Art and Life, Matila Ghyka presented an analysis of the face of the tennis champion Helen Wills.34 He made several measurements of Wills’ face, all of which were shown to be consistent with the divine proportion (Fig. 12-30). More recent examples include Salvador Dali’s use of the golden ratio in his masterpiece The Sacrament of the Last Supper (1955; Fig. 12-31) and the experimental piece of music Binary Universe (2006) by Brian Transeau. A strong advocate for the conscious application of the golden ratio to art, the design of objects, and architecture was Le Corbusier (1887-1965).32,59 His legacy remains one of the most influential in modern architecture. He developed an intense interest in proportional systems and their role in all forms of aesthetics. Le Corbusier’s fascination with the importance of the golden ratio in aesthetics centered around his belief that basic forms and structures are the underpinning of natural phenomenon. He developed what he called the Modulor in the tradition of Vitruvius with the intention of improving both the appearance and function of all manmade objects and architecture (Fig. 12-32).59 He was also a strong believer in the idea that ideal harmony in music (as stated by Pythagoras) could only be achieved through the application of the golden ratio to numbers.
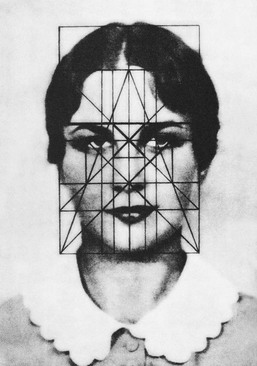
Figure 12-30 Facial proportions of Helen Wills by Matila Ghyk, confirming the golden ratio. From Matila Ghyk: The Geometry of Art and Life, 1946, Kessinger Publishing, Oct. 30, 2004, p. 102.
Current Philosophy
From Pythagoras, Polykleitos, Plato, Aristotle, Euclid, Vitruvius, Galen, Fibonacci, Piero, da Vinci, and Pacioli as well as the more recent Le Corbusier and all of their disciples for more than 2.5 millennia, we have learned that the proportions of the parts to the whole affect the observers’ impression of beauty and attractiveness.1,3,7,23,26,29,36,56,62,82,96 From the premier artists, architects, and designers throughout the centuries, we know that mirror-image symmetry and the harmony of proportions matter when judging facial aesthetics. They have also shown by example that the precise—even to the millimeter—achievement of an exacting mathematical ratio is not sufficient or even preferred for the creation of the most important end products; rather, an approximation of the golden ratio is.
In the realm of facial aesthetics and reconstructive surgery, clinicians from all involved fields have from time to time attempted to match facial aesthetics to a strict mathematical model (e.g., normative cephalometric measurements) without always considering individual biologic variations and aesthetic nuances* (Fig. 12-33). When doing so, they have often fallen short of their objective. In 1984, Ricketts reported about a significant number of golden proportions when analyzing the facial skeleton and overlying soft tissues.83–91 He was a strong advocate of the use of these divine proportions as a guide for planning the correction of dentofacial deformities via orthognathic surgery (Fig. 12-34). The validity of Ricketts’ approach to facial analysis for the correction of jaw deformities has been questioned by some while supported by most.8,12,15,30,50,73,74,75,76,94 For the clinician who is asked to correct a congenital or developmental craniofacial or maxillofacial deformity, attention is directed to both form and function. As R. Buckminster Fuller stated, “rarely are the two objectives divergent.”61 For the achievement of a harmonious face, attention is directed toward the practical objectives of improving the upper airway and the occlusion, which will affect speech, swallowing, chewing, and breathing. Facial aesthetic perfection is not a specific millimeter distance or degree of angulation but rather a visual impression at conversational distance in three-dimensional life. At the same time, neither divine proportions nor biologic realities and the patient’s personal preferences can be ignored throughout the process.
Part II: Clinical Evaluation of the Patient with a Dentofacial Deformity
Background
In the correction of a dentofacial deformity, the clinician’s aesthetic sense and knowledge of patient-specific biologic and psychosocial factors must be condensed into precise millimeter measurements and angular changes that are then accomplished on the surgically sectioned (osteotomized) maxilla, mandible, and chin region with a goal of establishing Euclidean facial proportions and improved function.* Although there is no singular normative table of numbers or a formula that can be relied on to direct the surgeon to the golden ratio, a methodical approach is nevertheless required. The clinician’s vision is based on 1) fundamental aesthetic principles 2) the biologic realities of the tissues 3) patient-specific dysfunctions (e.g., chewing, swallowing, speech articulation, breathing, lip closure/posture) and 4) individual treatment preferences (e.g., timing, techniques, extent of intervention).† It is expected that a degree of uneasiness about the end result will be felt by both the operating surgeon as well as the patient and his or her family during this process. With clinician experience, this creative tension for the surgeon and the uncertainty of the patient and his or her family are reduced; however, these can never be fully alleviated before surgery.
At the time of the preoperative, direct, face-to-face planning examination of the patient by the operating surgeon, several evaluations will have already been carried out.118,136,193,199,197,247 A review of relevant medical data in combination with facial photographic, radiographic, and dental analysis should be fresh in the surgeon’s mind. The patient’s facial structures are examined while he or she is upright and sitting in the natural head position (NHP). This is considered the most rational, physiologic, and anatomic orientation of the head and neck for the evaluation of the face, jaws, and teeth.* This is generally not the head and neck position in which the patient with a dentofacial deformity will naturally relax. Rather, the clinician must place the patient in the NHP. The teeth are also placed in centric relation or with a normal freeway space, and the lips are relaxed (i.e., not pursed together). The clinician then observes the patient three dimensionally at different angles in both repose and with dynamic facial expressions. It is from the NHP that the surgeon makes crucial decisions regarding what facial features are abnormal and the extent of required surgical reorientation.
Global Head and Neck Assessment: Seven Critical Aspects
1. Quality of the overlying soft-tissue envelope of the head and neck. The surgeon must recognize distortions of the soft-tissue envelope that will self-correct after the skeleton is reconstructed versus intrinsic soft-tissue malformations and deformities that will not (e.g., hypoplasia, hyperplasia, effects of trauma and aging).
2. Symmetry and harmony of the upper facial skeleton (i.e., cranial vault, orbits, nasofrontal process, and zygomatic arches). Although most individuals with dentofacial deformities have relatively normal upper facial skeletons, some do not (i.e., those with hemifacial microsomia or Treacher Collins syndrome).
3. Morphology of unique aesthetic units of the face (e.g., external nose, external ears, periorbital soft tissues).
4. History of temporomandibular joint symptoms and signs and the documentation of any limitations in the mandibular range of motion.
5. History of cervical spine symptoms and signs and the documentation of the safe neck range of motion.
6. Symmetry and harmony of the lower facial skeleton (i.e., maxilla, mandible, and chin region). The patient with a dentofacial deformity will have significant variations from normal in this region of the craniofacial skeleton.
7. Dental rehabilitative needs (i.e., restorative and periodontal) as well as orthodontic aspects.
Evaluation of the Overlying Soft-Tissue Envelope
An example of soft-tissue distortion (not deformity) is seen with maxillomandibular deficiency (see Chapter 23). The impression of “chubby cheeks” and excess fat in the neck is typical. With maxillomandibular (skeletal) advancement and vertical lengthening, the normal facial contours and curvatures will be restored.
There are exceptions with which the soft-tissue envelope is directly affected by a malformation (e.g., Treacher Collins syndrome, hemifacial microsomia, cleft lip, hemifacial hypertrophy), previous trauma (e.g., burns, lacerations), or scarring after infection. When a jaw deformity is associated with a syndrome (see Chapters 27 through 31), clefting (see Chapters 32, 33, and 34), or a soft-tissue traumatic deformity (see Chapter 35), the soft-tissue envelope may require special consideration.
Evaluation of the Upper Facial Skeleton
In the presence of a dentofacial deformity the cheeks and cheekbones may appear bigger or smaller than they actually are. The correction of the jaw deformity toward Euclidian proportions is likely to change the observer’s impression. An example of this phenomenon is seen in the patient with maxillary deficiency in combination with relative mandibular excess (Fig. 12-35, K & L). When the maxilla is advanced and rotated clockwise in conjunction with mandibular ramus osteotomies, the apparent flat cheekbones, deficient infraorbital rims, and enlarged nose typically disappear (see Chapter 20).
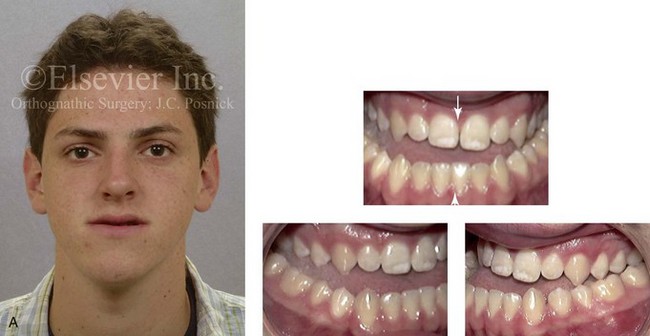
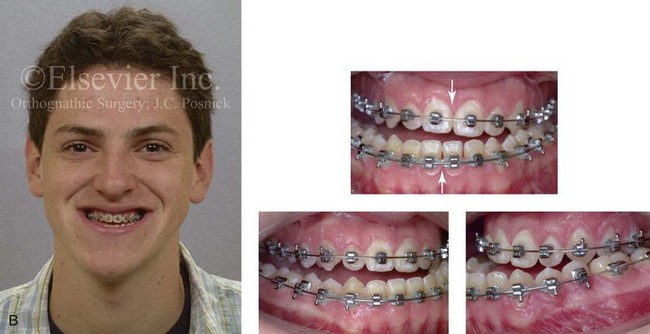
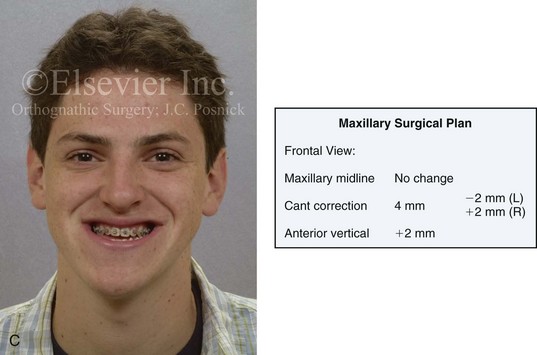
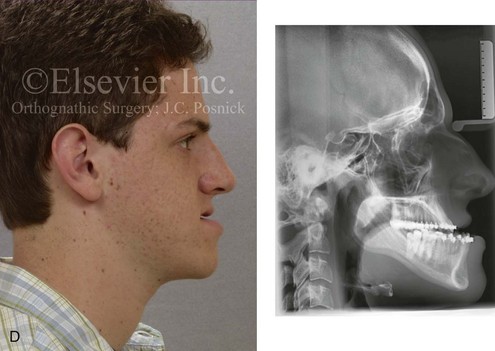
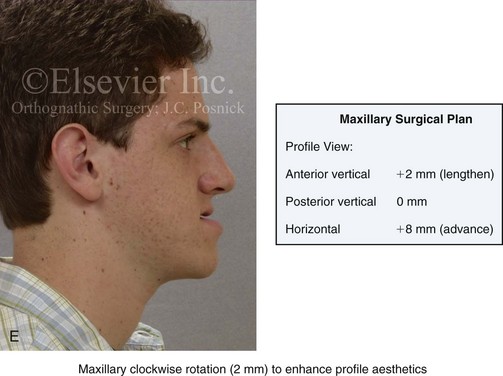
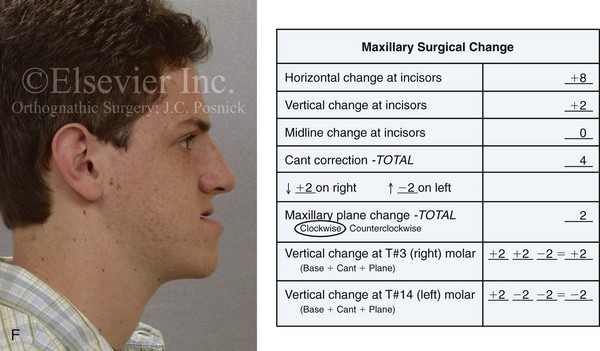
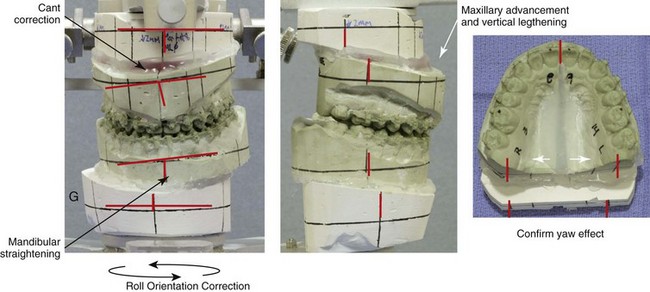
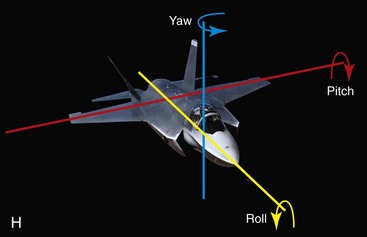

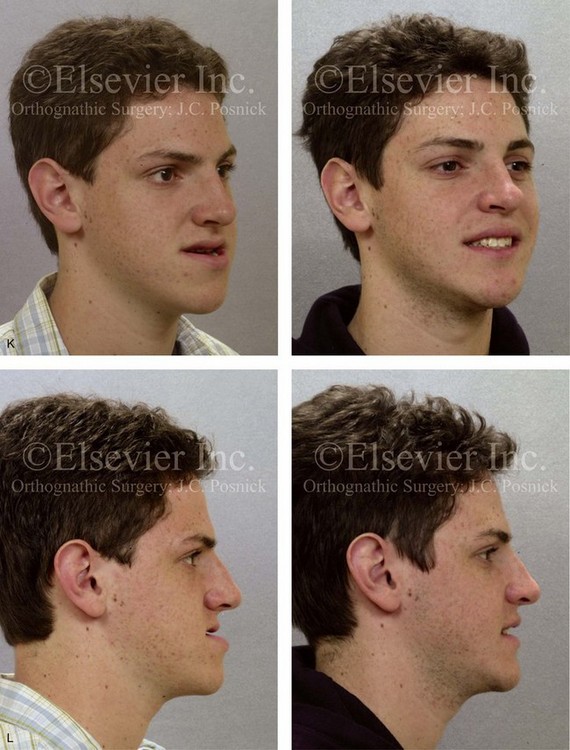
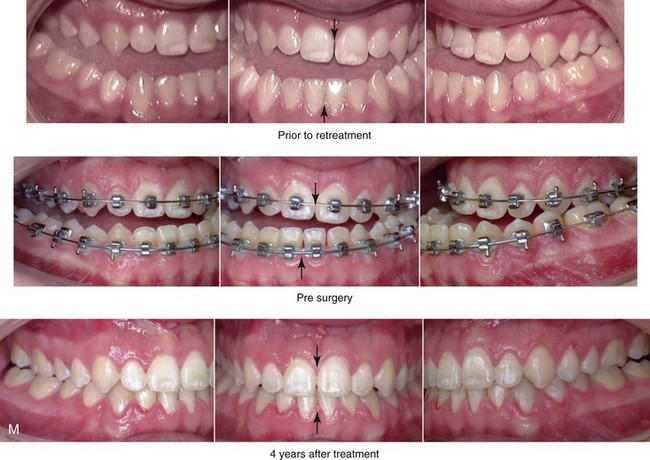
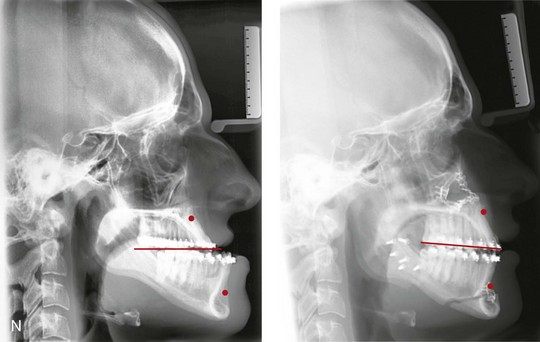
Figure 12-35 A 17-year-old boy with a diagnosis of left hemimandibular elongation. This dentofacial deformity affects the patient’s chewing, swallowing, speech articulation, lip closure/position, and breathing. He has normal cervical spine function and neck range of motion. He has no temporomandibular disorder, and he has a full range of mandibular motion. The upper facial skeleton (i.e., the cranial vault, the orbits, and the zygoma) is symmetric and with normal proportions. The facial soft-tissue envelope is distorted by the maxillomandibular deformity but without malformation. During his early teenage years, the patient underwent an initial phase of orthodontics without success. He was referred for surgical evaluation and then underwent a combined orthodontic and surgical approach. With the relief of dental compensation, his surgery included a maxillary Le Fort I osteotomy in segments (horizontal advancement, cant correction, vertical change, arch expansion, and clockwise rotation); bilateral sagittal split ramus osteotomies (cant correction and mandibular straightening); osseous genioplasty (straightening and minimal advancement); and septoplasty/inferior turbinate reduction. A, Frontal facial view in repose before treatment. Occlusal views before orthodontic retreatment are also shown. B, Frontal view with smile before surgery. Occlusal views with orthodontic (dental) decompensation in progress. C, The planned surgical repositioning of the maxilla is clarified during the preoperative direct visual examination. Frontal view changes will include no maxillary dental midline change; 4-mm cant correction (up 2 mm at the left first molar and down 2 mm at the right first molar); and a 2-mm vertical lengthening at the incisors. D, Profile facial view before treatment. Lateral cephalometric radiograph before treatment. E, The planned maxillary repositioning is clarified during the preoperative direct visual examination. Profile view changes will include vertical lengthening of the incisors (2 mm); horizontal advancement at the incisors (8 mm); and maxillary clockwise rotation (2 mm). F, The surgical plan for the repositioning of the maxilla is indicated on the standard orthognathic surgery model-planning data sheet. The data is then relied on to reorient the maxilla on the articulator with use of the Erickson Model Table. This is accomplished before splint construction. G, Articulated dental casts indicate analytic model planning (see Chapter 13). H, Illustration of roll, yaw, and pitch orientation. Consideration of reorienting the maxilla in accordance with these parameters is an essential part of planning. I, Frontal views in repose before and after reconstruction. J, Frontal views with smile before and after reconstruction. K, Oblique facial views before and after reconstruction. L, Profile views before and after reconstruction. M, Occlusal views before retreatment, with orthodontic decompensation in progress, and 4 years after treatment. N, Lateral cephalometric radiographs before and after surgery. H from Ackerman JL, Proffit WR, Sarver DM, et al: Pitch, roll, and yaw: describing the spatial orientation of dentofacial traits, Am J Orthod Dentofacial Orthop 131:305–310, 2007.
Evaluation of Unique (Discrete) Facial Aesthetic Units
The periorbital (adnexal) tissues may be of special concern as a result of 1) congenital hypoplasia (e.g., Treacher Collins syndrome, hemifacial microsomia, upper eyelid ptosis), 2) previous trauma (e.g., medial or lateral canthal injury, nasolacrimal apparatus injury, upper or lower eyelid injury), or 3) the normal aging process (e.g., descent, deflation, ptosis). The individual’s eyelid appearance may either add a sparkle to or be a distracting focal point of the face. For example, distortions of the lower eyelids are generally seen in patients with dentofacial deformities that are associated with maxillary deficiency and relative mandibular excess (i.e., negative vector relationship of the eyeballs and lower lids). This negative vector relationship affects the aesthetic appearance of the midface, which only worsens with age (see Chapter 25 and Fig. 12-35, D). Maxillary horizontal advancement and clockwise rotation in conjunction with mandibular ramus osteotomies corrects the skeleton and has positive effects on the soft tissues of the eyelids and the midface contours (see Chapter 20 and Fig. 12-35, K & L).
The external ears display considerable variations in size, shape, extent of protuberance, and asymmetry (see Chapter 39). Forensic pathologists consider details of auricular morphology to be as unique to the individual as that individual’s fingerprints. In association with specific syndromes (e.g., hemifacial microsomia, Treacher Collins syndrome), there may be auricular hypoplasia or a malformation that ranges from total microtia to cupping to just an abnormal location of the ears on the side of the face (see Chapters 27 and 28). Significant disproportion of the external ears, as a standalone and distinct feature, can be a distracting aesthetic aspect in the presence of an otherwise attractive face. An external ear set-back procedure may be carried out as part of the orthognathic surgery or later as a separate operation (see Chapter 39).
The external nose will display a wide range of aesthetic variation due to unique bony, cartilaginous, and soft-tissue anatomy (see Chapter 38). In the presence of a dentofacial deformity, the middle third of the central face (i.e., the nose) may appear bigger or smaller than its actual size (Fig. 12-35, L). The correction of the presenting jaw discrepancy toward Euclidean proportions will improve harmony between the upper, middle, and lower thirds of the face. After this is accomplished, a more accurate assessment of the actual nasal aesthetic unit can be made. Disproportion of the nose as a standalone aesthetic unit may nevertheless exist and benefit from alteration (see Chapter 38). Dysfunction of the nose characterized by chronic obstructive nasal breathing and inadequate sinus drainage should also be recognized and dealt with at the appropriate time (see Chapter 10). Clarification of these issues with the patient and his or her family at the first consultation visit as well as additional discussion at the time of orthognathic planning and again after surgical healing is essential to achieve patient satisfaction (see Chapter 7).
Assessment for Temporomandibular Disorder and Current Mandibular Range of Motion
An accurate history of any past and current temporomandibular disorder symptoms and signs should be documented, including any previous therapy; subjective complaints; joint noise; mandibular range of motion; any centric relation–centric occlusion discrepancy; masticatory muscle tenderness to palpation; and current radiographic findings. Any active temporomandibular disorder will require treatment to diminish symptoms and to achieve adequate range of motion before orthodontic treatment is initiated (see Chapter 9).
Evaluation of the Lower Facial Skeleton
Confirmation that the upper facial skeleton and the soft-tissue envelope of the face do not require surgical alteration and that the neck and mandible have an intact safe range of motion without significant limitations or discomforts is essential before focusing on detailed maxillomandibular surgical planning.142
Qualitative decisions concerning which components of the maxillomandibular skeleton are asymmetric or disproportionate and thus require surgical repositioning will have already been discussed with the patient, his or her family, and other treating clinicians before the initiation of treatment. These details should be reconfirmed by the orthognathic surgeon during the immediate preoperative planning visit.120,121,130,131,132,152,153,155,170
Assuming that both the maxilla and the mandible are part of the dentofacial deformity that requires reconstruction, quantitative (to the millimeter) decisions of the preferred aesthetic and best functional repositioning of the upper jaw (at the time of the Le Fort I osteotomy) must first be made.* When thinking through the details of maxillary surgical repositioning, it is understood that the “new” upper jaw position (in two-jaw surgery) will serve as the platform on which the mandible is to be placed. Where the lower jaw ends up is important and will be entirely dependent on the new surgical position of the maxilla and the orthodontic alignment of the teeth.159,160,187,195,196,198,205,212,213,214,216–221,235–237,240
Evaluation of Overall Dental Rehabilitation Needs
Recognizing when a patient who is seeking orthognathic surgery has additional dental needs beyond standard orthodontics is essential to the achievement of a favorable outcome. The need for periodontal evaluation and treatment as well as the need for complex dental restorative work should be considered for every patient before the initiation of a coordinated orthodontic and jaw surgical approach. This is especially true for the individual who has suffered dental and periodontal sequelae from a longstanding uncorrected dentofacial deformity and who may have undergone camouflage treatment in an attempt to neutralize the occlusion (e.g., orthodontics, occlusal equilibration, restorative dentistry) (see Chapters 5 & 6).
Detailed Maxillomandibular Surgical Planning: Eight Critical, Quantitative, To-the-Millimeter Decisions
1. Assess the current and preferred position of the maxillary dental midline with reference to the upper face (i.e., yaw orientation). Anticipate any potential yaw effect of the new surgical maxillary orientation on the mandible and the midface (i.e., the cheeks) (see Fig. 12-35, B).
2. Assess for the presence of any maxillary cant (i.e., roll orientation) with reference to the upper face. Determine the preferred upper jaw repositioning for cant correction (see Fig 12-35, B).
3. Assess the current and preferred vertical position of the maxillary incisor crown with reference to the upper face. Consider the upper-lip–incisor relationship in both repose and smile (see Fig 12-35, A and B).
4. Assess the current and preferred horizontal position of the maxillary incisor crown with reference to the upper face (see Fig 12-35, D).
5. Assess the current and preferred A-point to B-point relationship of the jaws (i.e., pitch orientation). When the face is viewed in profile in the NHP, consider the need to differentially alter the vertical maxillary height at the first molars and the incisors (i.e., maxillary plane change) to achieve specific objectives (see Fig 12-35, D, E, and F).
6. Assess the baseline pyriform rim, floor of nose, and anterior nasal spine morphology. Anticipate the location of each after midface reorientation, including any planned maxillary plane change. Consider the advantages of the recontouring of the pyriform rims, the floor of the nose, and the anterior nasal spine to achieve airway and aesthetic objectives (see Fig 12-35, D, E, and F).
7. Assess the baseline chin morphology. Anticipate the location of the pogonion after jaw reorientation that may also include mandibular plane change (i.e. pitch orientation). Consider the advantages of chin reshaping (i.e., osseous genioplasty) to achieve the preferred horizontal and vertical aesthetics and shape of the chin (see Fig 12-35, D, E, and F).
8. Reconfirm the advantage of and need for the segmentation of the maxilla to achieve the preferred arch form and occlusion with the mandible (see Fig 12-35, G).
Assess the Current and Preferred Position of the Maxillary Dental Midline (i.e., Yaw Orientation) with Reference to the Upper Face
After the decision has been made to surgically reposition the maxilla and the mandible (i.e., two-jaw surgery), no additional risk is encountered by making a change in the maxillary dental midline position. In fact, the surgeon must make a conscious decision to either leave the midline as it is or to adjust it. During the immediate preoperative, direct, face-to-face examination, the operating surgeon should consider the preferred location of the maxillary dental midline.10,117,120,167,176,183 This is a clinical observation when viewing the patient at conversational distance and closer up. It is measured in millimeters of variation of the current maxillary dental midline position (i.e., the point between the incisors at the incisal edge) in comparison with the baseline upper facial midline.
Ideally, the midline between the maxillary central incisors should coincide, within 2 mm, with the facial midline. When the upper face has satisfactory basic symmetry, the decision of where to position the maxillary dental midline is generally straightforward (see Fig. 12-35, C). In the presence of upper face asymmetry—especially in combination with canting of the maxilla and asymmetry or disproportion of the mandible (see Chapter 28)—the decision of where to reposition the maxillary midline becomes more complex.
When the surgeon determines the preferred (new) location of the maxillary dental midline, the yaw effect of the midline change must also be taken into account (see Fig. 12-35, G). For example, the maxilla may be either bodily moved to one side to correct the incisor midline, or the maxillary dental midline maybe corrected while leaving the buttress regions (i.e., side to side) essentially unchanged. This would require a twisting of the maxilla rather than a bodily movement so that the posterior maxilla moves slightly anterior on one side while the other side moves slightly posterior. This is a similar movement to the twisting open of a lid on a jar. As stated, a twisting movement of the maxilla around the vertical axis of rotation (i.e., yaw orientation) results in an incisor midline shift and a simultaneous anterior-posterior shift at the molars. When twisting the maxilla, the resulting posterior shift will cause a relative Class III on one side and a Class II molar relationship on the other side. Alternatively, a lateral (side-to-side) shift of the maxilla would cause a different change in the yaw orientation (see Fig. 12-35, H).
Establishing the correct maxillary dental midline position and yaw orientation is especially of concern in cases that present with moderate to severe three-dimensional facial asymmetry of multiple bones (i.e., hemifacial microsomia). A three-dimensional computed tomography scan (i.e., from the top of the skull through bottom of the chin) can be helpful to appreciate where specific mirror-image dysmorphology exists and how best to manage it (see Chapter 27 & 28).
Assess for the Presence of Any Maxillary Cant (i.e., Roll Orientation) with Reference to the Upper Face
During the preoperative direct planning examination, the clinician should make an observation when viewing the patient face to face of whether the maxilla is vertically even (i.e., from side to side) or, if not, of how much of a deviation (cant) is present.109,117,141,157,192,231 What is generally considered a maxillary cant (i.e., vertical height discrepancies of the maxilla from side to side) may also be viewed from the perspective of the aesthetic line of the dentition or at the functional line of the occlusion (see Fig. 12-35, J). Maxillary cant considerations take into account the upper face (i.e., the structures above the maxilla). When the upper face is basically symmetric, the interpupillary line may be used as an accurate baseline reference (see Fig. 12-35, B and C).
Thought is simultaneously given to the yaw orientation (i.e., rotation around the Y axis) of the presenting maxillary deformity and the yaw effect at the level of the mandibular angles and the midface (cheek) regions anticipated as a result of the (planned) maxillary change (see Fig. 12-35, G). In complex facial asymmetry cases, the clinician’s ability to simultaneously analyze the presenting yaw and roll orientations and the subsequent aesthetic changes of the planned correction can be augmented with the use of craniofacial three-dimensional computed tomography scan imaging.
1. Use of a Fox plane during the direct visual examination. The placement of a Fox plane directly over the occlusal surfaces of the maxillary dentition is helpful to judge the presence and extent of maxillary cant as compared with the upper face (i.e., the interpupillary reference line). Soft wax can be added to the deficient side (i.e., wax can be placed in between the Fox plane and the occlusal surfaces of teeth) until the cant is corrected. The millimeter amount of wax buildup (e.g., at the first molar) is a measure of the cant. When using a Fox plane, the clinician must also consider the patient’s maxillary arch form. Often, two separate dentoalveolar planes (i.e., in the presence of a steep curve of Spee) have been accentuated by the orthodontic mechanics and will be managed with segmental osteotomies. How the Fox plane is seated to the patient’s maxillary occlusal plane is important when assessing for a cant.
2. Comparison measurements from a posterior-anterior cephalometric radiograph (left side to right side)
• Incisal edge to nasofrontal suture (millimeter distance, left and right sides)
• Canine edge to nasofrontal suture (millimeter distance, left and right sides)
• First molar edge to frontozygomatic suture (millimeter distance, left and right sides)
3. Comparison measurements from a three-dimensional craniofacial computed tomography scan (left side to right side)
• Incisal edge to nasofrontal suture (millimeter distance, left and right sides)
• Canine edge to nasofrontal suture (millimeter distance, left and right sides)
• First molar edge to frontozygomatic suture (millimeter distance, left side and right side)
4. Comparison measurements taken directly from the anthropometric (facial) surface (left side to right side)
Assess the Current and Preferred Vertical Position of the Maxillary Incisor Crown with Reference to the Upper Face
The relationship between the upper lip and the maxillary incisors represents a key facial aesthetic landmark. Ideally, with the upper lip at rest, several millimeters (i.e., 1 mm to 3 mm) of the lower aspect to the maxillary incisor crowns should be seen in frontal view. With a broad smile, the upper lip elevates and, ideally, the entire maxillary incisor crown and at least a few millimeters of the gingiva will be seen. This will vary when evaluating a posed smile (as for a photograph) versus a dynamic smile (as seen when laughing).* The aesthetic line of the dentition follows the surfaces of the maxillary anterior and posterior teeth. The functional line of occlusion takes into account arch form, arch width, and symmetry. In the presence of malocclusion—as a result of distortions in arch width, curve of Spee, and overall arch form—the functional line of occlusion may not be even or on a single plane (see Fig 12-35, J). With many of the typical developmental jaw deformities, the lip-to-tooth relationship (i.e., the aesthetic line and the functional line) will show variation from ideal. Affected individuals will benefit from differential surgical repositioning of the anterior and posterior maxilla (i.e., intrusion or lengthening) as part of the surgical correction not only to positively influence the occlusion itself but also to influence the aesthetic line of the dentition and the functional line of occlusion.
Through a Le Fort I osteotomy with vertical intrusion, less gingiva with smile and incisor crown height in repose will be seen. With surgical (vertical) lengthening of the upper jaw during the Le Fort I osteotomy, more dental show and gingival exposure are to be expected (see Fig 12-35, I and J). Unfortunately, even with a pure vertical vector change of the maxilla, the upper lip soft-tissue response is generally less than a one-to-one relationship and not entirely predictable. Soft-tissue predictions become even less accurate in combination with other upper jaw vector changes (i.e., horizontal, and transverse); in the presence of maxillary asymmetries (i.e., cant and dental midline shifts); when other procedures are simultaneously carried out (e.g., mandible and chin osteotomies, pyriform rim/nasal floor/anterior nasal spine recontouring); and in the presence of a hypotonic upper lip (e.g., longstanding skeletal Class III malocclusion) or an immobile scarred upper lip (e.g., fibrosis after cleft lip repair).
Nevertheless, with thoughtful planning, a favorable aesthetic outcome involving the relationship of the incisor vertical position to the upper lip should be achieved for the vast majority of orthognathic patients (see Fig. 12-35, I and J). As a general rule, it is best to prevent too little maxillary incisor and gingival show in relationship to the upper lip, because this will result in an edentulous, aged, or sad look. Remember that, with age, the upper lip lengthens as a result of a loss of muscle tone. As long as excess lip separation at rest and mentalis strain with closure are avoided, erring on the side of more rather than less incisor show is generally the preferred aesthetic objective.
Assess the Current and Preferred Horizontal Position of the Maxillary Incisor Crown with Reference to the Upper Face
1. Cephalometric analysis. A lateral cephalogram of the patient is taken in (or adjusted to) the NHP, in centric relation, and with the lips relaxed.
2. Andrews’ analysis. A lateral facial photograph of the patient is taken in (or adjusted to) the NHP, in centric relation, with a broad smile (i.e., exposing the maxillary incisors) and with the exposure of the forehead skin (i.e., with the hair pulled back).
3. Anthropometric surface analysis. A direct visual examination of the patient’s face is made by viewing him or her in the NHP from various perspectives, both in repose and with a smile.
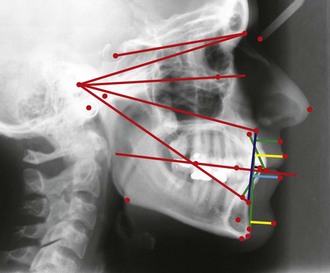
As stated, Andrews’ analysis can be a helpful tool to assess the preferred horizontal (sagittal) projection of the maxilla in the patient with a dentofacial deformity. This author’s application of Andrews’ “Element II of Orofacial Harmony” is illustrated in Figure 12-36. A step-by-step outline of how to do so is also given here:
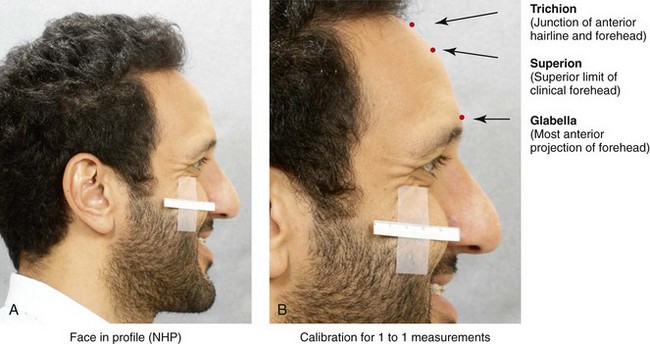
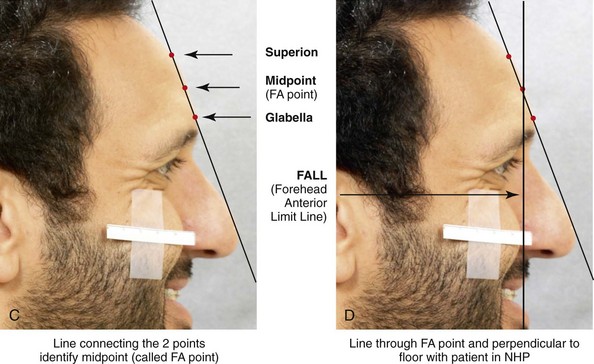
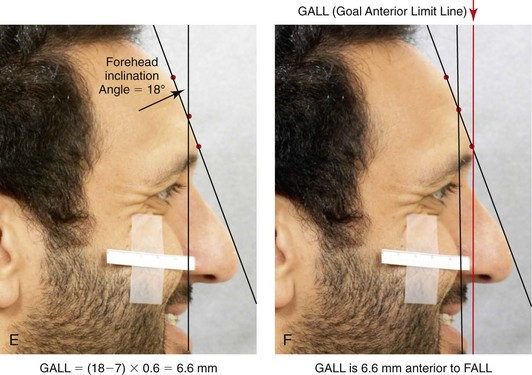
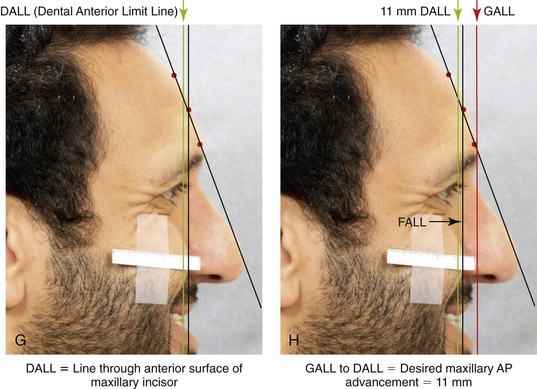
Figure 12-36 Patient viewed in profile in the natural head position (NHP). He is shown as a case example to demonstrate application of Andrews’ “Element II of Orofacial Harmony.” (See text for explanation.)
• View a photograph of the individual’s head and neck in profile. The photograph is best taken in the NHP and with a broad smile so that the labial surfaces of the maxillary incisors are fully visualized. The forehead skin up to the hairline must also be exposed, so the hair should be pulled back, if necessary. There should be a calibration scale on the facial photograph to confirm that it is life size (i.e., 1 to 1) so that accurate measurements can be made.
• Identify the trichion: This is the junction of the forehead skin and the anterior hairline. It is considered the most superior aspect of the forehead when the forehead contour is relatively flat.
• Identify the superion: This is typically considered the most superior aspect of the clinical forehead. This is the point used when the forehead is either rounded or angular in contour.
• Identify the glabella: This is the most anterior projection of the lower forehead.
• Draw a line that connects the superion and the glabella (assuming that the patient has an angular or rounded forehead).
• Identify the midpoint between the superion and the glabella: This is called the forehead anterior point (FA point).
• Locate and drop a vertical line through the FA point. This line should be perpendicular to the floor when viewing the patient’s photograph in the NHP. This is called the forehead anterior limit line (FALL).
• Measure the angle at the superior forehead between these two lines. This angle is a measure of forehead inclination.
• Locate and drop a second vertical line that is anterior and parallel to the FALL. This second line is called the goal anterior limit line (GALL). The location of this line is measured in millimeters anterior to the FALL in accordance with the following standard formula: (Forehead inclination angle − 7) × 0.6 = _____ mm.
• Locate and drop a third vertical line that is parallel to both the FALL and the GALL. This line is called the dental anterior limit line (DALL). The line runs through the anterior surface of the maxillary incisors. The difference (in mm) between the GALL and the DALL is the “recommending” distance that the maxilla should be horizontally (sagittally) advanced to improve midface aesthetics in the patient with a maxillary deficiency. This is assuming that Andrews’ Element I is correct (i.e., that the proper arch shape-positioning of the maxillary teeth [roots] over basal bone is correct).
Additional Considerations to Andrews’ Standard Analysis
• I have found that Andrews’ analysis for the preferred maxillary incisor position (i.e., Element II) is best suited for Caucasian patients. When applying Andrews’ formula to Asian, African, or Indian morphology, caution is warranted. Facial variations in forehead, nasal, and midface morphology are characteristically influenced by race.
• I have found that variations in skin thickness can have a significant effect on the preferred aesthetic sagittal position of the maxilla. This is an additional factor that should be taken into account when reorienting the maxilla in conjunction with a Le Fort I osteotomy. For example, the individual with a thick soft-tissue envelope (e.g., a patient with obstructive sleep apnea and an elevated body mass index) may handle a greater horizontal advancement than Andrews’ analysis indicates. The opposite may be true for the individual with a thin soft-tissue envelope.
• Andrews points out that midface aesthetics in profile are highly dependent on the ideal positioning of the maxillary teeth within the arch (i.e., Element I). An example of this is seen in the patient with a maxillary deficient and relative mandibular excess growth pattern. If maxillary bicuspid extractions are not carried out (when required) to provide space for the orthodontic retraction of the incisors toward a normal inclination, then midface aesthetics will be negatively affected. Unless Element I has first been achieved, the procumbent maxillary incisors will negatively affect the nasolabial angle in repose, the draping of the upper lip with a smile, and the baseline nasal base aesthetics.
The achievement of a favorable smile is influenced by several factors, including but not limited to: 1) the quality of the perioral soft tissues 2) the vertical position of the maxilla 3) the horizontal position of the maxilla 4) the maxillary arch width and 5) the inclination of the anterior teeth within the arch (see Fig. 35, I and J). The smile arc is analyzed in the frontal view by evaluating parallel lines that represent the lower lip and the upper dentition. The ideal smile arc (i.e., the consonant smile line) is characterized by the curvature of the lower lip line parallel to the curvature of the maxillary incisal edges. A consonant smile line is felt to project a happy and youthful appearance. The buccal corridor is the space that is created between the buccal surfaces of the posterior teeth and the inner aspect of the oral commissure when the individual is smiling. Those with excessive buccal corridors (i.e., negative space) are generally rated as having less attractive smiles than those with smaller buccal corridors. When the maxilla is narrow and horizontally retrusive, the visual appearance of dark buccal corridors reduces the attractiveness of the smile. Smile aesthetics are enhanced when the display zone is filled with the maxillary teeth. This concept is best appreciated when viewing photographs of the smile before and after orthognathic surgery for an individual with maxillary deficiency in combination with relative mandibular excess who presents with a Class III negative overjet malocclusion (see Fig. 12-35, I and J). The improvement of the smile aesthetics in the patient with a maxillary deficiency are often accomplished through a combination of maxillary arch expansion, horizontal advancement, and the adjustment of the vertical height.
When determining the preferred horizontal (surgical) repositioning of the maxilla, an important facial aesthetic consideration is the interrelationship of the anterior maxillary wall (i.e., above the alveolar process), the ocular globes, and the lower eyelids (see Fig. 12-35, D). Horizontal advancement and clockwise rotation of the upper jaw (after Le Fort I osteotomy) for individuals who present with maxillary deficiency and relative mandibular excess are typically required to achieve and maintain favorable periorbital aesthetics (i.e., a positive vector relationship; see Chapter 20).
William Hogarth was an artist during the mid 1700s who published an analysis of facial beauty.188 He documented the aesthetic importance of establishing an unbroken convex contour from the lower eyelid to the cheek (see Fig 12-35, L). He felt strongly that this convex shape, also called the midface ogee curve, was fundamental to a youthful and attractive midface. Dr. John Little further characterized the ideal midface ogee curve when viewing the individual at conversational distance.170 This can also be appreciated on a medical-quality, high-resolution, oblique facial photograph. Little suggested that the surgeon use the midface ogee curve as an aesthetic reference standard when attempting to rejuvenate an aging face.
A lack of this youthful cheek region is often seen in individuals with either a long face Class II mandibular deficiency anterior open-bite growth pattern (see Chapter 21) or a maxillary deficiency relative mandibular excess growth pattern (see Fig. 12-35, D). Projecting the midface forward and appropriately rotating the maxillary plane (i.e., pitch orientation) as part of the orthognathic correction is often useful to recreate the natural attractive curvatures and contours of the otherwise deficient midface (see Fig. 12-35, L). This generally requires counterclockwise rotation for the long face growth pattern and clockwise rotation for the maxillary deficient relative mandibular excess growth pattern.
Assess the Current and Preferred A-Point to B-Point Relationship of the Jaws (i.e., Pitch Orientation)
The facial aesthetic advantage of achieving an ideal A-point to B-point relationship has been discussed by contemporary orthodontists and orthognathic surgeons.109,161,162,187,198,205,212–217 The individual who presents with a dentofacial deformity will frequently have a disharmonious A-point to B-point relationship, which results in negative influences on facial aesthetics (see Fig. 12-35, D). This deviation from ideal, when viewed in profile and in the NHP, tends to be predictable in accordance with the specific pattern of developmental jaw deformity (e.g., long face growth pattern, maxillary deficiency with relative mandibular excess, primary mandibular deficiency).
For most patients with dentofacial deformities, the achievement of preferred facial aesthetics requires the planned operative rotation of the maxillary and mandibular planes (i.e., pitch orientation) to correct A-point to B-point disproportion (see Fig. 12-35, L and N). This may require clockwise or counterclockwise rotation of the maxillary and mandibular planes, but it rarely requires a true lengthening or shortening of the posterior facial height.198,205,212–217
When using sagittal split ramus osteotomies, the unchanged proximal segment of the mandible establishes the posterior facial height, whereas any plane change carried out occurs in the distal segment. The height of the proximal segment (i.e., the posterior facial height) does not change with either clockwise or counterclockwise rotation of the distal segment in conjunction with a sagittally split ramus osteotomy (see Chapter 15).
The assessment of the profile aesthetics should be made with the patient in the Natural Head Position with the teeth in centric relation and then with the normal freeway space. The lips are first relaxed (i.e., not pursed together) and then also assessed with full smile (see Fig. 12-35 A, B, and D). Individuals who present with a jaw discrepancy frequently have an altered mode of respiration and tend to rest their heads in positions that an experienced evaluator will view as involving either neck extension or flexion or a head tilt. The adjustment of the individual’s head position by the clinician to the NHP is required to accurately assess the profile.
Oftentimes, the patient’s lateral facial photograph and lateral cephalometric radiograph are not taken with the patient’s head in the NHP. If the profile photograph, the three-dimensional computed tomography scan, and the lateral cephalometric radiograph are to be used for facial aesthetic analysis, then the image should first be rotated to establish NHP (see Fig. 12-35, D). From this position, a perpendicular line may be dropped (e.g., from the nasion, the soft-tissue glabella or from subnasion) as a vertical reference line from which to assess the A-point to B-point relationship, the horizontal jaw projection, and the effects on the overlying soft tissues. The clinician should then consider the need for operative rotation (i.e. pitch alteration) of the maxillary and mandibular planes as part of the orthognathic correction. This is to bring the A-point just in front of the B-point and to bring both of them in correct proximity to the vertical reference line (which is perpendicular to the NHP) to achieve enhanced facial projection.
The establishment of a favorable A-point to B-point relationship is an important part of approximating Euclidian proportions and of recreating the natural attractive curvatures and contours of the face (see Chapter 40). Achieving the preferred A-point and B-point relationship will influence the relative position of the skeletal structures above (i.e., the anterior wall of the maxilla, the pyriform rims, and the anterior nasal spine) and below (i.e., the pogonion) (see Fig. 12-35, L and N). The position of these superior and inferior skeletal structures will then be reflected through the overlying facial soft-tissue envelope. This includes the soft tissues of 1) the lower eyelids (i.e., improvements in the tear trough and the negative vector); 2) the cheeks (i.e., improvements in the nasolabial folds); 3) the nose (i.e., improvements in depressed nasal sills and a plunging tip); 4) the lips (i.e., improvements in turned-down oral commissures and marionette lines); 5) the chin (i.e., the prevention of ptosis); and 6) the neck (i.e., improvements in an obtuse neck angle and a double chin).
The surgical repositioning of the jaws to improve the A-point to B-point relationship is also carried out with consideration of its effects on the relative inclination of the maxillary and mandibular incisors (see Fig 12-35, N). The rotation of the maxillary plane can improve the relationship of the maxillary smile arc to make it consonant with the lower lip. With respect to incisor inclination, when the maxillary plane is surgically rotated clockwise, the relative incisor inclination decreases. When the maxillary plane is surgically rotated counterclockwise, the incisors become more procumbent. When the mandibular plane is rotated clockwise, the incisors become more proclined. When the mandibular plane is rotated counterclockwise, the incisors become more retroclined. These effects must be considered during the presurgical orthodontic phase and again at the time of detailed preoperative planning.
Assess the Baseline Pyriform Rims, Floor of Nose, and Anterior Nasal Spine Morphology: Anticipate the Location of each after Maxillary Reorientation
The satisfactory surgical positioning of the A-point and the B-point (i.e., pitch orientation) will generally result in more pleasing aesthetics at the pyriform aperture (paranasal) regions154,178,198,205,212–217 (see Fig. 12-35, L and N). However, at the time of Le Fort I osteotomy, the recontouring of the pyriform rims, the floor of the nose, and the anterior nasal spine region to correct baseline skeletal deformities may also be beneficial (see Chapter 15). The advantage of these recontouring procedures to the upper lip and tooth relationship and the aesthetics of the base of the nose should always be considered during preoperative planning.
Riola’s textbook of cephalometric measurements confirms that the normal mean vertical height of the anterior nasal spine down to the maxillary incisal edge is 30 mm (±3 mm) for females and 33 mm (±3 mm) for males.209 At the time of the Le Fort I osteotomy, the patient’s specific measurements can be confirmed with a caliper. This is useful information to guide nasal floor reconstruction. For example, with the long face growth pattern, a patient who is undergoing Le Fort I osteotomy with vertical intrusion to reduce a gummy smile will frequently have an average height of between 38 mm and 45 mm. At the time of LeFort I osteotomy, recontouring and lowering the floor of the nose and the anterior nasal spine toward 30 mm is useful to normalize anatomy. In so doing, the nasal airway and the nasal aesthetic unit will be favorably enhanced (see Fig. 16-19).
Assess the Baseline Chin Morphology: Anticipate the Location of the Pogonion after Jaw Reorientation
An individual’s lateral cephalometric radiograph when viewed in the NHP is useful to assess the 1) anterior facial heights, 2) any baseline bony chin dysmorphology, 3) the maxillary and mandibular incisor inclination and 4) the A-point to B-point relationships (see Fig. 12-35, N).
Riola’s table of normative mandibular cephalometric values confirms that the mean vertical height of the chin (i.e., from the mandibular incisal edge to the menton) is approximately 42 mm (±2.7 mm) for females and 49.4 mm (±2.9 mm) for males.210 These measurements can be a useful benchmark from which to judge the preferred vertical height of the chin.
The effective alteration of the A-point to B-point relationship at the time of orthognathic surgery will in itself generally have a positive effect on the chins appearance.198,205,212–217 Additional direct surgical alteration of the chin (i.e., osseous genioplasty) is also frequently beneficial to achieve enhanced facial aesthetics (i.e., vertical lengthening or shortening, midline shifts, horizontal advancement or in some cases recontouring of the chin surface anatomy; see Chapter 37).
Reconfirm the Advantage of and Need for the Segmentation of the Maxilla
Segmentation is generally performed as either a “two-piece” osteotomy, with an interdental osteotomy between the incisors and then parasagittally through the hard palate, or as a “three-piece” osteotomy, with interdental osteotomies between the lateral incisor and canine on each side and then down the parasagittal midline on one or both sides (see Chapter 15).
The segmentation of the upper jaw is not an excuse for poor orthodontic preparation. It does provide an opportunity to correct skeletal issues through the basal bone rather than through orthodontic compensation that would otherwise position the teeth outside or on the edge of the alveolar process (see Chapter 17). An orthodontic compensation (i.e., dental camouflage) approach will likely be unstable for the occlusion and the periodontium in the long run (see Chapters 6 and 17).
These decisions (i.e., plans for maxillary segmentation) should be agreed upon before the initiation of orthodontic treatment, reconfirmed at the time of immediate presurgical model planning, demonstrated on the articulated dental models, registered on the prefabricated acrylic splints, and then executed during surgery (see Fig. 12-35, G).
References
Part I: Facial Proportions and Symmetry
1. Alley, TR, Cunningham, MR. Average faces are attractive, but very attractive faces are not average. Psychol Sci. 1991; 2:123–125.
2. Altemus, L. Comparative integumental relationships. Angle Orthod. 1962; 33:217–221.
3. Amoric, M. The golden number: application to craniofacial evaluation. Funct Orthod. 1995; 12(1):18–21.
4. Arnett, GW, McLaughlin, RP. Facial and dental planning for orthodontists and oral surgeons. London: Mosby/Elsevier; 2004.
5. Artmann, B. Euclid: the creation of mathematics. New York: Springer; 1999.
6. Auger, TA, Turley, PK. The female soft tissue profile as presented in fashion magazines during the 1900s: a photographic analysis. Int J Adult Orthodon Orthognath Surg. 1999; 14:7–18.
7. Baig, MA. Surgical enhancement of facial beauty and its psychological significance. Ann R Australas Coll Dent Surg. 2004; 17:64–67.
8. Baker, BW, Woods, MG. The role of the divine proportion in the aesthetic improvement of patients undergoing combined orthodontic/orthognathic surgical treatment. Int J Adult Orthodon Orthognath Surg. 2001; 16(2):108–120.
9. Ball, WWR. A short account of the history of mathematics, ed 4. New York: Dover Publications; 1960.
10. Bashour, M. An objective system for measuring facial attractiveness. Plast Reconstr Surg. 2006; 118:757–774.
11. Bass, NM. The aesthetic analysis of the face. Eur J Orthod. 1991; 13:343–350.
12. Benson, P, Perrett, D. Face to face with the perfect image. New Scientist. 1992; 133:32.
13. Boyer, CB. A history of mathematics, ed 2. New York: John Wiley & Sons Inc; 1991.
14. Carl, B, Merzbach, U. A history of mathematics, ed 2. New York: John Wiley & Sons Inc; 1989.
15. Cook, TA. The curves of life. New York: Dover Publications; 1979.
16. Cotton, WN, Takano, WS, Wong, WW, Wylie, WL. The Downs analysis applied to three other ethnic groups. Angle Orthod. 1951; 21:213–220.
17. Cox, NH, van der Linden, FPGM. Facial harmony. Am J Orthod. 1971; 60:175–183.
18. Coxeter, HSM. Regular polytopes, ed 3. New York: Dover Publications; 1973.
19. DeBruine, LM, Jones, BC, Unger, L, et al. Dissociating averageness and attractiveness: attractive faces are not always average. J Exp Psychol Hum Percept Perform. 2007; 33:1420–1430.
20. Decker, JD. The divine proportion. Am J Orthod Dentofacial Orthop. 2004; 126(1):19A–20A.
21. Delaire, J. L’analyse architecturale et structurale craniofaciale (de profil): principes theoriques: quelques exemples d’emploi en chirurgie maxillofacile. Rev Stomatol. 1978; 79:1–33.
22. Delaire, J, Schendel, S, Tulasne, JF. An architectural and structural craniofacial analysis: a new lateral cephalometric analysis. Oral Surg. 1981; 52:226–238.
23. De Smit, A, Dermaut, L. Soft tissue profile preferences. Am J Orthod. 1984; 86:67–73.
24. Donatsky, O, Bjorn-Jorgensen, J, Hermund, NU, et al. Accuracy of combined maxillary and mandibular repositioning and of soft tissue prediction in relation to maxillary antero-superior repositioning combined with mandibular set back: a computerized cephalometric evaluation of the immediate postsurgical outcome using the TIOPS planning system. J Craniomaxillofac Surg. 2009; 37:279.
25. Dunlap, RA. The golden ratio and Fibonacci numbers. Singapore and River Edge, NJ: World Scientific Publishing; 1997.
26. Dunlevy, HA, White, RP, Proffit, WR, Turvey, TA. Professional and lay judgment of facial esthetic changes following orthognathic surgery. Int J Adult Orthod Orthognath Surg. 1974; 2:151–158.
27. Eagly, AH, Ashmore, RD, Makhijani, iviG, Longo, LC. What is beautiful is good, but … : a meta-analytic review of research on the physical attractiveness stereotype. Psychol Bull. 1991; 110:109–128.
28. Epker, BN, Stella, JP, Fish, LC, Essentials of treatment planning. Dentofacial deformities: integrated orthodontic and surgical correction. ed 2. Mosby-Year Book, St. Louis, 1995.
29. Farkas, LG, Cheung, G. Facial asymmetry in healthy North American Caucasians: an anthropometrical study. Angle Orthod. 1981; 51:70–77.
30. Farkas, LG, Kolar, JC. Anthropometrics and art in the aesthetics of women’s faces. Clin Plast Surg. 1987; 14:599–615.
31. Ferrario, VF, Sforza, C, Ciusa, V, et al. The effect of sex and age on facial asymmetry in healthy subjects: a cross-sectional study from adolescence to mid-adulthood. J Oral Maxillofac Surg. 2001; 59:382–388.
32. Fischler, R. The early relations of Le Corbusier to the golden numbers. Environ Plann B Plann Des. 1979; 6:95–103.
33. Fitzgerald, R, Graivier, MH, Kane, M, et al. Facial aesthetic analysis. Aesthet Surg J. 2010; 30(Suppl):25S–27S.
34. Ghyka, M. The geometry of art and life. New York: Dover Publications Inc; 1977.
35. Gille, B. Alberti, Leone Battista. In: Dictionary of Scientific Biography, I. New York: Charles Scribner’s Sons; 1970:96–98.
36. Glassenberg, AN, Feinberg, DR, Jones, BC, et al. Sex-dimorphic face shape preference in heterosexual and homosexual men and women. Arch Sex Behav. 2010; 39:1289–1296.
37. Gonzalez-Ulloa, M. Quantitative principles in cosmetic surgery of the face (profileplasty). Plast Reconstr Surg. 1962; 29:186.
38. Gonzalez-Ulloa, M. A quantum method for the appreciation of the morphology of the face. Plast Reconstr Surg. 1964; 34:241.
39. Grimm, RE. The autobiography of Leonardo Pisano. Fibonacci Quarterly. 1973; 11(1):99–104.
40. Hambridge, J. Dynamic symmetry: the Greek vase. New Haven, Conn: Yale University Press; 1920.
41. Harkleroad, L. The math behind the music. Cambridge, UK: Cambridge University Press; 2006.
42. Heath, TL. Euclid and the traditions about him. In: Heath TL, ed. Euclid elements 1:1-6. Perseus Digital Library, 1908.
43. Heath, TL. The thirteen books of Euclid’s elements, ed 2. New York: Dover Publications; 1956.
44. Heath, TL. A history of Greek mathematics. New York: Dover Publications; 1981.
45. Hemenway, P. Divine proportion: phi in art, nature, and science. New York: Sterling; 2005.
46. Henri, R. The art spirit. Philadelphia: J. B. Lippincott; 1960.
47. Hermann, A. To think like God: Pythagoras and Parmenides—the origins of philosophy. Chicago: Parmenides Publishing; 2005.
48. Hogarth, W. Analysis of beauty. In: Paulson R, ed. New Haven. Conn: Yale University Press; 1997:5.
49. Huntley, HE. The divine proportion: a study in mathematical beauty. New York: Dover Publications; 1970.
50. Iglesias-Linares, A, Yanez-Vico, R-M, Moreno-Manteca, B, et al. Common standards in facial esthetics: craniofacial analysis of most attractive black and white subjects according to People magazine during previous 10 years. J Oral Maxillofac Surg. 2011; 69:e216–e224.
51. Jacobson, A. Orthognathic diagnosis using the proportionate template. J Oral Surg. 1980; 38:820–833.
52. Jacobson, A. Planning for orthognathic surgery—art or science? Int J Adult Orthod Orthognath Surg. 1990; 5(4):217–224.
53. Kiekens, RM, Kuijpers-Jagtman, AM, van’t Hof, MA, et al. Putative golden proportions as predictors of facial aesthetics in adolescents. Am J Orthod Dentofacial Orthop. 2008; 134:480–483.
54. Kiekens, RM, Kuijpers-Jagtman, AM, van’t Hof, MA, et al. Facial aesthetics in adolescents and its relationship to “ideal” ratios and angles. Am J Orthod Dentofacial Orthop. 2008; 133:188.
55. Kingsley, P. Ancient philosophy, mystery and magic: Empedocles and the Pythagorean tradition. Oxford, UK: Oxford University Press; 1995.
56. Komori, M, Kawamura, S, Ishihara, S. Averageness or symmetry: which is more important for facial attractiveness? Acta Psychol (Amst). 2009; 131:136–142.
57. Langlois, JH, Roggman, LA. Attractive faces are only average. Psychol Sci. 1990; 1:115–121.
58. Langlois, JH, Roggman, LA, Musselman, L. What is average and what is not average about attractive faces? Psychol Sci. 1994; 5:214–220.
59. Le, Corbusier. The modulor. London: Faber and Faber Ltd; 1951.
60. Lele, S, Richtsmeier, JT. Euclidean distance matrix analysis: a coordinate-free approach for comparing biological shapes using landmark data. Am J Phys Anthropol. 1991; 86:415–427.
61. Livio, M. The golden ratio: the story of phi, the world’s most astonishing number. New York: Broadway Books; 2002.
62. Lombardi, RE. The principles of visual perception and their clinical application to denture esthetics. J Prosthet Dent. 1973; 29:358–382.
63. Martin, JG. Racial ethnocentrism and judgment of beauty. J Soc Psychol. 1964; 63:59–63.
64. McNamara, JA, Jr. Cephalometric analysis of untreated adults with ideal facial and occlusal relationships. Int J Orthodon Orthognath Surg. 1988; 3:221–231.
65. Merrifield, LL. The profile line as an aid in critically evaluating facial aesthetics. Am J Orthod. 1966; 52:804–822.
66. Midhat Gazale, G. From pharaohs to fractals. Princeton, NJ: Princeton University Press; 1999.
67. Nguyen, DD, Turley, PK. Changes in the Caucasian male facial profile as depicted in fashion magazines during the twentieth century. Am J Orthod Dentofacial Orthop. 1998; 114:208–217.
68. O’Connor, JJ, Robertson, EF. Euclid of Alexandria. http://www-history. mcs. st-andrews. ac. uk/Biographies/Euclid. html.
69. O’Meara, DJ. Pythagoras revived. Oxford, UK: Oxford University Press; 1989.
70. Ore, O. Number theory and its history. McGraw-Hill; 1948.
71. Pacioli, L. De divina proportione. Venice: Paganinus de Paganinis; 1509.
72. Panofsky, E. Renaissance and renascenses in Western art. Stockholm: Almqvist & Wiksell; 1960.
73. Peck, H, Peck, S. Concept of facial aesthetics. Angle Orthod. 1970; 40:284.
74. Peck, H, Peck, S. The aesthetically pleasing face: an orthodontic myth. Trans Eur Orthod Soc. 1971; 47:175.
75. Peck, S, Peck, L, Kataja, M. Some vertical lineaments of lip position. Am J Orthod Dentofacial Orthop. 1992; 101:519–524.
76. Peck, S, Peck, L. Selected aspects of the art and science of facial esthetics. Semin Orthod. 1995; 1:105–126.
77. Perrett, DI, May, KA, Yoshikawa, S. Facial shape and judgments of female attractiveness. Nature. 1994; 368:239–242.
78. Powell, N, Humphreys, B. Proportions of the aesthetic face. New York: Thieme-Stratton; 1984.
79. Reyneke, JP. Essentials of orthognathic surgery. Chicago: Quintessence Publishing Co Inc; 2003.
80. Rhodes, G, Harwood, K, Yoshikawa, S, et al. The attractiveness of average faces: cross-cultural evidence and possible biological basis. In: Rhodes G, Zebrowitz L, eds. Facial attractiveness: evolutionary, cognitive, and social perspectives. Westport, Conn: Ablexm, 2001.
81. Rhodes, G, Tremewan, T. Averageness, exaggeration, and facial attractiveness. Psychol Sci. 1996; 7:105–110.
82. Rhodes, G, Yoshikawa, S, Clark, A, et al. Attractiveness of facial averageness and symmetry in non-Western cultures: in search of biologically based standards of beauty. Perception. 2001; 30:611–625.
83. Ricketts, RM. Planning treatment on the basis of the facial pattern and an estimate of its growth. Angle Orthod. 1957; 27:14–37.
84. Ricketts, RM. Cephalometric synthesis. Am J Orthod. 1960; 46:647–673.
85. Ricketts, RM. Cephalometric analysis and synthesis. Angle Orthod. 1961; 31:141.
86. Ricketts, RM. Esthetics, environment, and the law of lip relation. Am J Orthodont. 1968; 54:272–289.
87. Ricketts, RM. A detailed consideration of the line of occlusion. Angle Orthod. 1978; 48:274–282.
88. Ricketts, RM. Perspectives in the clinical application of cephalometrics, the first 50 years. Angle Orthod. 1981; 51:115.
89. Ricketts, RM. Divine proportion in facial aesthetics. Clin Plast Surg. 1982; 9(4):401–422.
90. Ricketts, RM. The biologic significance of the divine proportion and Fibonacci series. Am J Orthod. 1982; 81(5):351–370.
91. Ricketts, RM, Roth, RH, Chaconas, SJ, et al. Orthodontic diagnosis and planning. Denver: Rocky Mountain Data Systems; 1982.
92. Runion, GE. The golden section and related curiosa. Glenview, Ill: Scott, Foresman & Co; 1972.
93. Sahlqvist, L. Cardinal alignments and the golden section: principles of ancient cosmography and design, ed 3. Charleston SC: BookSurge; 2008.
94. Sassouni, V. A classification of skeletal facial types. Am J Orthod Dentofacial Orthop. 1969; 55:109.
95. Sharabi, SE, Hatef, DA, Hollier, LH. Facial attractiveness: is the whole more than the sum of its parts? Aesthetic Surg J. 2010; 30:154–160.
96. Shell, TL, Woods, MG. Facial aesthetics and the divine proportion: a comparison of surgical and non-surgical Class II treatment. Aust Orthod J. 2004; 20:51–63.
97. Sigler, LE. Fibonacci’s Liber Abaci. Springer-Verlag; 2002.
98. Sinclair, PM. The divine proportion. Am J Orthod. 1982; 82(2):166–167.
99. Stakhov, AP. The mathematics of harmony: from Euclid to contemporary mathematics, mathematics and computer science. Singapore: World Scientific Publishing; 2009.
100. Stewart, A. One hundred Greek sculptors: their careers and extant works, part III of Stewart’s Greek sculpture. New Haven, Conn: Yale University Press; 1990.
101. Struik, DJ. A concise history of mathematics. New York: Dover Publications; 1967.
102. Weyl, H. Symmetry. Princeton, NJ: Princeton University Press; 1952.
103. Wolfe, SA, Hu, L, Berkowitz, S. In search of the harmonious face: Apollo revisited, with an examination of the indications for retrograde maxillary displacement. Plast Reconstr Surg. 1997; 99:1261.
104. Xia, J, Shevchenko, L, Gateno, J, et al. Outcome study of computer-aided surgical simulation in the treatment of patients with craniomaxillofacial deformities. J Oral Maxillofac Surg. 2011; 69:2014–2024.
105. Zeising, A. Neue lehre van den proportionen des meschlischen korpers. Leipzig; 1854.
Part II: Clinical Evaluation of the Patient
106. Ackerman, J, Ackerman, MB, Brensinger, CM, Landis, JR. A morphometric analysis of the posed smile. Clin Orthod Res. 1998; 1:2–11.
107. Ackerman, JL, Proffit, WR. Soft tissue limitations in orthodontics: treatment planning guidelines. Angle Orthod. 1997; 67:327–336.
108. Ackerman, JL, Proffit, WR, Sarver, DM. The emerging soft tissue paradigm in orthodontic diagnosis and treatment planning. Clin Orthod Res. 1999; 2:49–52.
109. Ackerman, JL, Proffit, WR, Sarver, DM, et al. Pitch, roll, and yaw: describing the spatial orientation of dentofacial traits. Am J Orthod Dentofacial Orthop. 2007; 131:305–310.
110. Ackerman, M, Ackerman, J. Smile analysis and design in the digital era. J Clin Orthod. 2002; 36:221.
111. Alexander, SA. Diagnosis and treatment planning in orthodontics. Curr Opin Dent. 1992; 2(4):9–13.
112. Altemus, LA. A comparison of cephalofacial relationships. Angle Orthod. 1960; 30:223–240.
113. Andrews, W, Andrews, L. Six elements of orofacial harmony. The Andrews Journal of Orthodontics and Orofacial Harmony. 2000; 1:1.
114. Arnett, GW, Bergman, RT. Facial keys to orthodontic diagnosis and treatment planning—part I. Am J Orthod Dentofacial Orthop. 1993; 103:299–312.
115. Arnett, GW, Bergman, RT. Facial keys to orthodontic diagnosis and treatment planning—part II. Am J Orthod Dentofacial Orthop. 1993; 103:395–411.
116. Arnett, GW, Jelic, JS, Kim, J, et al. Soft tissue cephalometric analysis: diagnosis and treatment planning of dentofacial deformity. Am J Orthod Dentofacial Orthop. 1999; 116:239–253.
117. Babister, W. Aircraft dynamic stability and response. Elsevier; 1980.
118. Bell, R, Kiyak, HA, Joondeph, DR, et al. Perceptions of facial profile and their influence on the decision to undergo orthognathic surgery. Am J Orthod. 1985; 88:323–832.
119. Bell, WH, Jacobs, JD, Quejada, JG. Simultaneous repositioning of the maxilla, mandible and chin: treatment planning and analysis of soft tissues. Am J Orthod. 1986; 89:28–50.
120. Beyer, JW, Lindauer, SJ. Evaluation of dental midline position. Semin Orthod. 1998; 4:146–152.
121. Bishara, SE. Longitudinal soft-tissue profile changes: a study of three analyses. Am J Orthod. 1985; 88:209–223.
122. Bishara, SE, Jakobsen, JR, Hession, TJ, Treder, JE. Soft tissue profile changes from 5 to 45 years of age. Am J Orthod Dentofacial Orthop. 1998; 114:698–706.
123. Bittner, C, Pancherz, H. Facial morphology and malocclusions. Am J Orthod Dentofacial Orthop. 1990; 97:308–315.
124. Bjerin, R. A comparison between the Frankfort horizontal and the sella turcica-nasion as reference planes in cephalometric analysis. Acta Odontol Scand. 1957; 15:1–13.
125. Bjork, A. The face in profile: an anthropological x-ray investigation on Swedish children and conscripts. Svensk Tandl Tidskr. 40(Suppl), 1947.
126. Bjork, A. Cranial base development. Am J Orthodont. 1955; 41:198–225.
127. Broadbent, BH, Sr., Broadbent, GH, Jr., Golden, WH. Bolton standards of dentofacial developmental growth. St. Louis: C. V. Mosby; 1975.
128. Burstone, CJ. The integumental profile. Am J Orthod. 1958; 44:1–25.
129. Burstone, CJ. Integumental contour and extension patterns. Angle Orthod. 1959; 29:93–104.
130. Burstone, CJ. Lip posture and its significance in treatment planning. Am J Orthod. 1967; 53:262–284.
131. Carlotti, AE, Aschaffenburg, PH, Schendel, SA. Facial changes associated with surgical advancement of the lip and maxilla. J Oral Maxillofac Surg. 1986; 44:593.
132. Chaconas, SJ, Bartroff, JD. Prediction of normal soft tissue facial changes. Angle Orthod. 1975; 45:12–25.
133. Cleall, J, Alexander, WJ, McIntyre, HM. Head posture and its relationship to deglutition. Angle Orthod. 1966; 36:335–350.
134. Cooke, MS, Wei, SH. A summary five-factor cephalometric analysis based on natural head posture and true horizontal. Am J Orthod Dentofac Orthop. 1988; 93:213–233.
135. Cooke, MS, Wei, SH. The reproducibility of natural head posture: a methodological study. Am J Orthod Dentofac Orthop. 1988; 93:280–288.
136. Cunningham, SJ, Crean, SJ, Hunt, NP, et al. Preparation, perceptions and problems: a long-term follow-up study of orthognathic surgery. Int J Adult Orthod Orthognath Surg. 1996; 11:41–47.
137. Dann, JJ, Fonseca, RJ, Bell, WH. Soft tissue changes associated with total maxillary advancement: a preliminary study. J Oral Surg. 1976; 34:19.
138. Downs, WB. The role of cephalometrics in orthodontic case analysis and diagnosis. Am J Orthod. 1952; 38:162–182.
139. Downs, WB. Analysis of the dentofacial profile. Am J Orthodont Oral Surg. 1956; 26:192–212.
140. Ekman, P, Davidson, RJ, Friesen, WV. The Duchenne smile: emotional expression and brain physiology. J Pers Soc Psychol. 1990; 58:3432.
141. Ellis, E, III., Johnson, DG, Hayward, JR. Use of orthognathic surgery simulating instrument in the presurgical evaluation of facial asymmetry. J Oral Maxillofac Surg. 1984; 42:805.
142. Enlow, D, Kuroda, T, Lewis, A. The morphological and morphogenic basis for craniofacial form and pattern. Angle Orthod. 1971; 41:161–188.
143. Epker, BN, Stella, JP, Fish, LC, Essentials of treatment planning. Dentofacial deformities: integrated orthodontic and surgical correction. ed 2. Mosby-Year Book, St. Louis, 1995.
144. Farkas, LG, Posnick, JC. Growth and development of regional units in the head and face based on anthropometric measurements. Cleft Palate Craniofac J. 1992; 29(4):301–302.
145. Farkas, LG, Posnick, JC, Hreczko, T. Anthropometric growth study of the ear. Cleft Palate Craniofac J. 1992; 29(4):324–329.
146. Farkas, LG, Posnick, JC, Hreczko, T. Anthropometric growth study of the head. Cleft Palate Craniofac J. 1992; 29(4):303–308.
147. Farkas, LG, Posnick, JC, Hreczko, T. Growth patterns of the face: a morphometric study. Cleft Palate Craniofac J. 1992; 29(4):308–315.
148. Farkas, LG, Posnick, JC, Hreczko, T, Pron, G. Growth patterns in the orbital region: a morphometric study. Cleft Palate Craniofac J. 1992; 29(4):315–318.
149. Farkas, LG, Posnick, JC, Hreczko, T, Pron, G. Growth patterns of the nasolabial region: a morphometric study. Cleft Palate Craniofac J. 1992; 29(4):318–324.
150. Fish, LC, Epker, BN. Surgical-orthodontic cephalometric prediction tracing. J Clin Orthod. 1980; 14:36–52.
151. Fjellvang, H, Solow, B. Craniocervical postural relations and craniofacial morphology in 30 blind subjects. Am J Orthodont Dentofacial Orthop. 1986; 90:327–334.
152. Fogel, ML, Stranc, MF. Lip function: a study of normal lip parameters. Br J Plast Surg. 1984; 37:542.
153. Freihofer, HPM, Jr. The lip profile after correction of retro-maxillism in cleft and non-cleft patients. J Maxillofac Surg. 1976; 4:136–141.
154. Freihofer, HPM, Jr. Changes in nasal profile after maxillary advancement in cleft and non-cleft patients. J Maxillofac Surg. 1977; 5:20–27.
155. Friede, H, Kahnberg, KE, Adell, R, et al. Accuracy of cephalometric prediction in orthognathic surgery. J Oral Maxillofac Surg. 1987; 45:754.
156. Gateno, J, Teichgraeber, JF, Xia, JJ. Three-dimensional surgical planning for maxillary and midface distraction osteogenesis. J Craniofac Surg. 2003; 14:833–839.
157. Gateno, J, Xia, JJ, Teichgraeber, JF, et al. Clinical feasibility of computer-aided surgical simulation (CASS) in the treatment of complex cranio-maxillofacial deformities. J Oral Maxillofac Surg. 2007; 65(4):728–734.
158. Hambleton, RS. The soft tissue covering of the skeletal face as related to orthodontic problems. Am J Orthod. 1964; 50:405–420.
159. Hayes, RJ, Sarver, DM, Jacobson, AJ. Quantification of cervicomental angle changes with mandibular advancement. Am J Orthod Dentofacial Orthop. 1994; 105:383–391.
160. Hoffman, GR, Staples, G, Moloney, FB. Cephalometric alterations following facial advancement surgery: 1. Statistical evaluation: a review of aesthetic evaluation of the face and an attempt to assess treatment validity. J Craniomaxillofac Surg. 1994; 22:214–219.
161. Holdaway, RA. A soft tissue cephalometric analysis and its use in orthodontic treatment planning: part 1. Am J Orthod. 1983; 84(1):1–28.
162. Holdaway, RA. A soft-tissue cephalometric analysis and its use in orthodontic treatment planning: part II. Am J Orthod. 1984; 85:279–293.
163. Hulsey, CM. An aesthetic evaluation of tooth-lip relationships present in smile. Am J Orthod. 1970; 57:132–144.
164. Jacobson, A. The “Wits” appraisal of jaw disharmony. Am J Orthod. 1975; 67:125–138.
165. Johnston, CD, Burden, DJ, Stevenson, MR. The influence of dental midline discrepancies on dental attractiveness ratings. Eur J Orthod. 1999; 21:517–522.
166. Krogman, WM, Sassouni, V. A syllabus in roentgenographic cephalometry. Philadelphia: Philadelphia Center for Research in Child Growth; 1957.
167. Latta, GH. The midline and its relation to anatomic landmarks in the edentulous patient. J Prosthet Dent. 1988; 59:681–683.
168. Lavelle, CL. A study of craniofacial form. Am J Orthod. 1979; 49:65–72.
169. Legan, HL, Burstone, CI. Soft tissue cephalometric analysis for orthognathic surgery. J Oral Surg. 1980; 38:744–751.
170. Little, JW. Volumetric perceptions in midfacial aging with altered priorities for rejuvenation. Plast Reconstr Surg. 2000; 105:252.
171. Lundström, A, Lundström, F, Lebret, LM, Moorrees, CF. Natural head position and natural head orientation: Basic considerations in cephalometric analysis. Eur J Orthod. 1995; 17:111–120.
172. Lundström, F, Lundström, A. Natural head position as a basis for cephalometric analysis. Am J Orthod Dentofac Orthop. 1992; 101:244–247.
173. Mansour, S, Burstone, C, Legan, H. An evaluation of soft-tissue changes resulting from Le Fort I maxillary surgery. Am J Orthod. 1983; 84:37–47.
174. Mauchamp, O, Sassouni, V. Growth and prediction of the skeletal and soft tissue profiles. Am J Orthod. 1973; 64:83–94.
175. Michiels, LYF, Tourne, LPM. Nasion true vertical: a proposed method for testing the clinical validity of cephalometric measurements applied to a new cephalometric reference line. Int J Adult Orthod Orthog Surg. 1990; 5:43–52.
176. Miller, EL, Bodden, WR, Jamison, HC. A study of the relationship of the dental midline to the facial median line. J Prosthet Dent. 1979; 41:657–660.
177. Mohl, N. Head posture and its role in occlusion. Int J Orthodont. 1977; 15:6–14.
178. Mommaerts, MY, Lippens, F, Abeloos, J, et al. Nasal profile changes after maxillary impaction and advancement surgery. J Oral Maxillofac Surg. 2000; 58:1198.
179. Moore, T, Southard, KA, Casko, JS, et al. Buccal corridors and smile esthetics. Am J Orthod Dentofacial Orthop. 2005; 127:208.
180. Moorrees, CF. Natural head position: a revival. Am J Orthod Dentofacial Orthop. 1994; 105:512–513.
181. Moorrees, CF, Kean, MR, Coenroad, FA. Natural head position: a basic consideration for the analysis of cephalometric radiographs. Am J Phys Anthropol. 1958; 16:213–234.
182. Morley, J, Eubank, J. Macroesthetic elements of smile design. J Am Dent Assoc. 2001; 132:39–45.
183. Moses, JJ, Lo, HH. The use of asymmetric yaw in the correction of lateral facial defects in hemifacial microsomia deformities: a case report. Int J Adult Orthodon Orthognath Surg. 1992; 7(4):229–234.
184. Mulhave, A. En biostatick undersogelse: mennekts staende stilling teorestisk og statometrisk belyst. Copenhagen: Munksgard; 1958.
185. Murphy, KE, Preston, CB, Evans, WG. The development of instrumentation for the dynamic measurement of changing head posture. Am J Orthod Dentofacial Orthop. 1991; 99:520–526.
186. Nahoum, HI. Anterior open-bite: a cephalometric analysis and suggested treatment procedures. Am J Orthod. 1975; 67:513–521.
187. Nattestad, A, Vedtofte, P. Mandibular autorotation in orthognathic surgery: a new method of locating the centre of mandibular rotation and determining consequences in orthognathic surgery. J Craniomaxillofac Surg. 1992; 20:163.
188. O’Ryan, F, Lassetter, J. Optimizing facial esthetic in the orthognathic surgery patient. J Oral Maxillofac Surg. 2011; 69:702–715.
189. O’Ryan, F, Schendel, S. Nasal anatomy and maxillary surgery: I. Esthetic and anatomic principles. Int J Adult Orthodon Orthognath Surg. 1989; 4:27.
190. O’Ryan, F, Schendel, S. Nasal anatomy and maxillary surgery: II. Unfavorable nasolabial esthetics following the Le Fort I osteotomy. Int J Adult Orthodon Orthognath Surg. 1989; 4:75.
191. O’Ryan, F, Schendel, S. Nasal anatomy and maxillary surgery: III. Unfavorable nasolabial esthetics following the Le Fort I osteotomy. Int J Adult Orthodon Orthognath Surg. 1989; 4:157.
192. Padwa, BL, Kaiser, MO, Kaban, LB, et al. Occlusal cant in the frontal plane as a reflection of facial asymmetry. J Oral Maxillofac Surg. 1997; 55:811.
193. Papadopoulos, MA, Lazaridou-Terzoudi, T, Oland, J, et al. Comparison of soft and hard tissue profiles of orthognathic surgery patients treated recently and 20 years earlier. Oral Surg Oral Med Oral Pathol Oral Radiol Endod. 2009; 108:e8.
194. Parekh, S, Fields, HW, Beck, FM, et al. The acceptability of variations in smile arc and buccal corridor space. Orthod Craniofac Res. 2007; 10:15.
195. Park, YC, Burstone, CJ. Soft tissue profile—fallacies of hard tissue standards in treatment planning. Am J Orthod Dentofacial Orthop. 1986; 90(I):52–62.
196. Posnick, JC. Discussion: Occlusal plane rotation: aesthetic enhancement in mandibular micrognathia. Plast Reconstr Surg. 1993; 91:1241–1244.
197. Posnick, JC. Quantitative computer tomographic scan analysis: normal values and growth patterns. In: Posnick JC, ed. Craniofacial and maxillofacial surgery in children and young adults. Philadelphia: W. B. Saunders Company; 2000:36–54.
198. Posnick, J, Fantuzzo, J, Orchin, J. Deliberate operative rotation of the maxillo-mandibular complex to alter the A-point to B-point relationship for enhanced facial esthetics. J Oral Maxillofac Surg. 2006; 64:1687–1695.
199. Posnick, JC, Farkas, L. Anthropometric surface measurements in the analysis of craniomaxillofacial deformities: normal values and growth trends. In: Posnick JC, ed. Craniofacial and maxillofacial surgery in children and young adults. Philadelphia: W. B. Saunders Company; 2000:55–79.
200. Proffit, WR, Epker, BN. Treatment planning for dentofacial deformities. In: Bell WH, Proffit WR, White R, eds. Surgical correction of dentofacial deformities. Philadelphia: W. B. Saunders Company, 1980.
201. Proffit, WR, Fields, HW, Sarver, DM. Contemporary orthodontics, ed 4. St. Louis: C. V. Mosby; 2007.
202. Proffit, WR, White, RP. Surgical-orthodontic treatment. St. Louis: C. V. Mosby; 1991.
203. Proffit, WR, White, RP, Jr., Sarver, DM. Contemporary treatment of dentofacial deformities. St. Louis: C. V. Mosby; 2002.
204. Reidel, RA. An analysis of dentofacial relationships. Am J Orthod. 1957; 43:103–119.
205. Reyneke, JP, Evans, WG. Surgical manipulation of the occlusal plane. Int J Adult Orthodon Orthognath Surg. 1990; 5:99–110.
206. Richardson, A. An investigation into the reproducibility of some points, planes and lines used in cephalometric analysis. Am J Orthod. 1966; 52:631–651.
207. Rickets, RM. The evolution of diagnosis to computerized cephalometrics. Am J Orthod. 1969; 55:795–803.
208. Riedel, RA. An analysis of dentofacial relationships. Am J Orthod. 1957; 43:i3–i19.
209. Rigsbee, OH, Sperry, TP, BeGole, EA. The influence of facial animation in smile characteristics. Int J Adult Orthodon Orthognath Surg. 1988; 3:233–239.
210. Riolo, ML, Moyers, RE, McNamara, JA, Hunter, WS. An atlas of craniofacial growth: cephalometric standards from the University School Growth Study. Craniofacial Growth Series Monograph No. 2. Ann Arbor: Center for Human Growth and Development, University of Michigan; 1974.
211. Rosen, H. Aesthetics in facial skeletal surgery. Perspectives in Plastic Surgery. 1993; 6:1.
212. Rosen, HM. Maxillary advancement for mandibular prognathism: indications and rationale. Plast Reconstr Surg. 1991; 87:823.
213. Rosen, HM. Facial skeletal expansion: treatment strategies and rationale. Plast Reconstr Surg. 1992; 89:798.
214. Rosen, HM. Aesthetic perspectives in jaw surgery. New York: Springer; 1999.
215. Salzmann, JA. The research workshop on cephalometrics. Am J Orthod. 1960; 46:834–847.
216. Sarver, DM. Diagnosis and treatment planning of hypodivergent skeletal pattern with clockwise occlusal plane rotation. Int J Adult Orthodon Orthognath Surg. 1993; 8:113–121.
217. Sarver, DM. Aesthetic orthodontics and orthognathic surgery. St. Louis: Mosby; 1997.
218. Sarver, DM. The importance of incisor positioning in the esthetic smile: the smile arc. Am J Orthod Dentofacial Orthop. 2001; 120:98–111.
219. Sarver, DM, Ackerman, JL. Orthodontics about face: the reemergence of the aesthetic paradigm. Am J Orthod Dentofacial Orthop. 2000; 117:575–576.
220. Sarver, DM, Ackerman, MB. Dynamic smile visualization and quantification. Part 2: Smile analysis and design. Am J Orthod Dentofacial Orthop. 2003; 124:116–127.
221. Sassouni, V, Nanda, S. Analysis of dentofacial vertical proportions. Am J Orthod. 1964; 50:801.
222. Savara, BS, Singh, IJ. Norms of size and annual increments of seven anatomical measures of maxillae in boys from 3 to 16 years of age. Angle Orthod. 1968; 38:104.
223. Scheideman, GB, Bell, WH, Legan, HL, et al. Cephalometric analysis of dentofacial normals. Am J Orthod. 1980; 78(4):404–420.
224. Schendel, SA, Carlotti, AE. Nasal considerations in orthognathic surgery. Am J Orthod Dentofac Orthop. 1991; 100:197–208.
225. Solow, B, Tallgren, A. Natural head position in standing subjects. Acta Odontol Scand. 1971; 29:591–607.
226. Solow, B, Tallgren, A. Head posture and craniofacial morphology. Am J Phys Anthropol. 1976; 44:417–436.
227. Spoylar, JL. Head position error in cephalometric radiography: an implant study. Angle Orthod. 1987; 57:77–88.
228. Steiner, CC. Cephalometrics in clinical practice. Angle Orthod. 1959; 29:8–29.
229. Steiner, CC. The use of cephalometrics as an aid to planning and assessing orthodontic treatment. Am J Orthod Dentofacial Orthop. 1960; 46:721.
230. Stella, JP, Streater, MR, Epker, BN, Sinn, DP. Predictability of upper lip soft tissue changes with maxillary advancement. J Oral Maxillofac Surg. 1989; 47:697–703.
231. Stengel, RF. Flight dynamics. Princeton, NJ: Princeton University Press; 2004.
232. Subtelny, JD. A longitudinal study of soft tissue facial structures and their profile characteristics, defined in relation to underlying skeletal structures. Am J Orthod. 1959; 45:481–507.
233. Talass, MF, Baker, RC. Soft tissue profile changes resulting from retraction of maxillary incisors. Am J Orthod Dentofacial Orthop. 1987; 91:385–394.
234. Thompson, JR. The rest position of the mandible and its application to analysis and correction of malocclusion. Angle Orthod. 1949; 19:162–187.
235. Tjan, A, Miller, G, The, G. Some esthetic factors in a smile. J Prosthet Dent. 1984; 51:24–28.
236. Troulis, MJ, Everett, P, Seldin, EB, et al. Development of a three-dimensional treatment planning system based on computed tomographic data. Int J Oral Maxillofac Surg. 2002; 31(4):349–357.
237. Turvey, TA, et al. Surgical-orthodontic treatment planning for simultaneous mobilization of the maxilla and mandible in the correction of dentofacial deformities. Oral Surg. 1982; 54:491.
238. Tweed, CH. Frankfort mandibular incisor angles in diagnosis, treatment planning and prognosis. Angle Orthod. 1954; 24:121–169.
239. Usumez, S, Orhan, M. Reproducibility of natural head position measured with an inclinometer. Am J Orthod Dentofacial Orthop. 2003; 123:451–454.
240. Vanarsdale, R, White, R. Three-dimensional analysis for skeletal problems. Int J Adult Orthodon Orthognath Surg. 1994; 9:159.
241. Viazis, AD. A cephalometric analysis based on natural head position. J Clin Orthod. 1991; 25:172–181.
242. Viazis, AD. Comprehensive assessment of anteroposterior jaw relationships. J Clin Orthod. 1992; 26:673–680.
243. Vig, PS, Showfety, KJ, Phillips, C. Experimental manipulation of head posture. Am J Orthod. 1978; 48:175–186.
244. Vig, R, Brundo, G. The kinetics of anterior tooth display. J Prosthet Dent. 1978; 39(5):502–504.
245. Wagner, I, Carlsson, G, Ekstrand, K, et al. A comparative study of assessment of dental appearance by dentists, dental technicians and laymen using computer-aided image manipulation. J Esthet Dent. 1996; 8:199–205.
246. West, RA, McNeill, RW. Maxillary alveolar hyperplasia, diagnosis and treatment planning. J Maxillofac Surg. 1975; 3:239.
247. Wolfe, SA, Berkowitz, S. Plastic surgery of the facial skeleton. Boston: Little, Brown and Company; 1989.
248. Worms, FW, Isaacson, RJ, Speidel, TM. Surgical orthodontic treatment planning: profile analysis and mandibular surgery. Angle Orthod. 1976; 46(1):1–25.
249. Wylie, GA, Fish, LC, Epker, BN. Cephalometrics: a comparison of five analyses currently used in the diagnosis of dentofacial deformities. Int J Adult Orthod Orthog Surg. 1987; 2(1):15–36.
250. Zachrisson, BU. Aesthetic factors involved in anterior tooth display and the smile; vertical dimension. J Clin Orthod. 1998; 32:432–445.
*2, 4, 10, 11, 16, 21, 22, 24, 28, 31, 33, 37, 38, 51, 52, 60, 64, 65, 70, 78, 79, 95, 103, 104, 108, 153, 177, 200, 241, 246
*107, 111, 112, 122, 125, 138, 139, 161, 162, 166, 206, 207, 210, 215, 219, 223, 228, 229, 240, 248, 249
†114, 115, 116, 123, 127, 128, 129, 143, 144–150, 158, 174, 186, 189–191, 201–204, 208, 211, 222
*24, 124, 126, 133–135, 151, 168, 172, 171, 177, 181, 180, 184, 185, 225–227, 234, 238, 239, 243
*106, 109, 110, 113, 117, 119, 137, 140, 141, 156, 157, 163–165, 167–169, 170, 175, 176, 179, 182, 183, 188, 194, 224, 230–233, 242, 244, 245
*References 106, 110, 130, 131, 137, 140, 152, 173, 179, 194, 209, 218, 220, 230, 233, 244, 250